A uniform rod of length l and mass m is given a charge Q and is suspended vertically by means of a hinge at the top end. A horizontal electric field E is switched on, in the direction in which the rod can sway freely. Find the angle made by the rod with the vertical in equilibrium.
The correct Answer is:tan−1(QEmg)
To solve the problem, we need to analyze the forces acting on the uniformly charged rod when it is subjected to a horizontal electric field. Here’s a step-by-step solution:
Step 1: Identify the Forces Acting on the Rod
The rod experiences two main forces:
1. Weight (W): The weight of the rod acts downward and is given by W=mg, where m is the mass of the rod and g is the acceleration due to gravity.
2. Electric Force (F): The electric force acting on the rod due to the electric field E is given by F=QE, where Q is the charge on the rod.
Step 2: Set Up the Equilibrium Condition
In equilibrium, the rod will make an angle θ with the vertical. The forces acting on the rod can be resolved into components. The weight acts vertically downward, while the electric force acts horizontally.
Step 3: Analyze the Forces
At equilibrium, the tangent of the angle θ can be expressed as the ratio of the electric force to the weight of the rod:
tan(θ)=Electric ForceWeight=FW
Substituting the expressions for the electric force and weight, we have:
tan(θ)=QEmg
Step 4: Solve for the Angle θ
To find the angle θ, we take the arctangent of both sides:
θ=tan−1(QEmg)
Final Answer
Thus, the angle made by the rod with the vertical in equilibrium is:
θ=tan−1(QEmg)
The rod experiences two main forces:
1. Weight (W): The weight of the rod acts downward and is given by W=mg, where m is the mass of the rod and g is the acceleration due to gravity.
2. Electric Force (F): The electric force acting on the rod due to the electric field E is given by F=QE, where Q is the charge on the rod.
In equilibrium, the rod will make an angle θ with the vertical. The forces acting on the rod can be resolved into components. The weight acts vertically downward, while the electric force acts horizontally.
At equilibrium, the tangent of the angle θ can be expressed as the ratio of the electric force to the weight of the rod:
tan(θ)=Electric ForceWeight=FW
Substituting the expressions for the electric force and weight, we have:
tan(θ)=QEmg
To find the angle θ, we take the arctangent of both sides:
θ=tan−1(QEmg)
Thus, the angle made by the rod with the vertical in equilibrium is:
θ=tan−1(QEmg)
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-I)13videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Exercise57videos
ELECTRIC CHARGES AND FIELDS
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Additional Exercise11videos
ELECTROMAGNETIC INDUCTION
Similar Questions
A uniform rod of length l and mass m is free to rotate in a vertical plane about A as shown in Fig. The rod initially in horizontal position is released. The initial angular acceleration of the rod is
 View Solution
View SolutionA rod AB of length L and mass m is uniformly charged with a charge Q, and it is suspended from end A as shown in fig. The rod can freely rotate about A in the plane of the figure. An electric field E is suddenly switched on in the horizontal direction due to which the rod gets turned by a maximum angle of 90∘. The magnitude of E is equal of nMg/Q. Find the value of n.
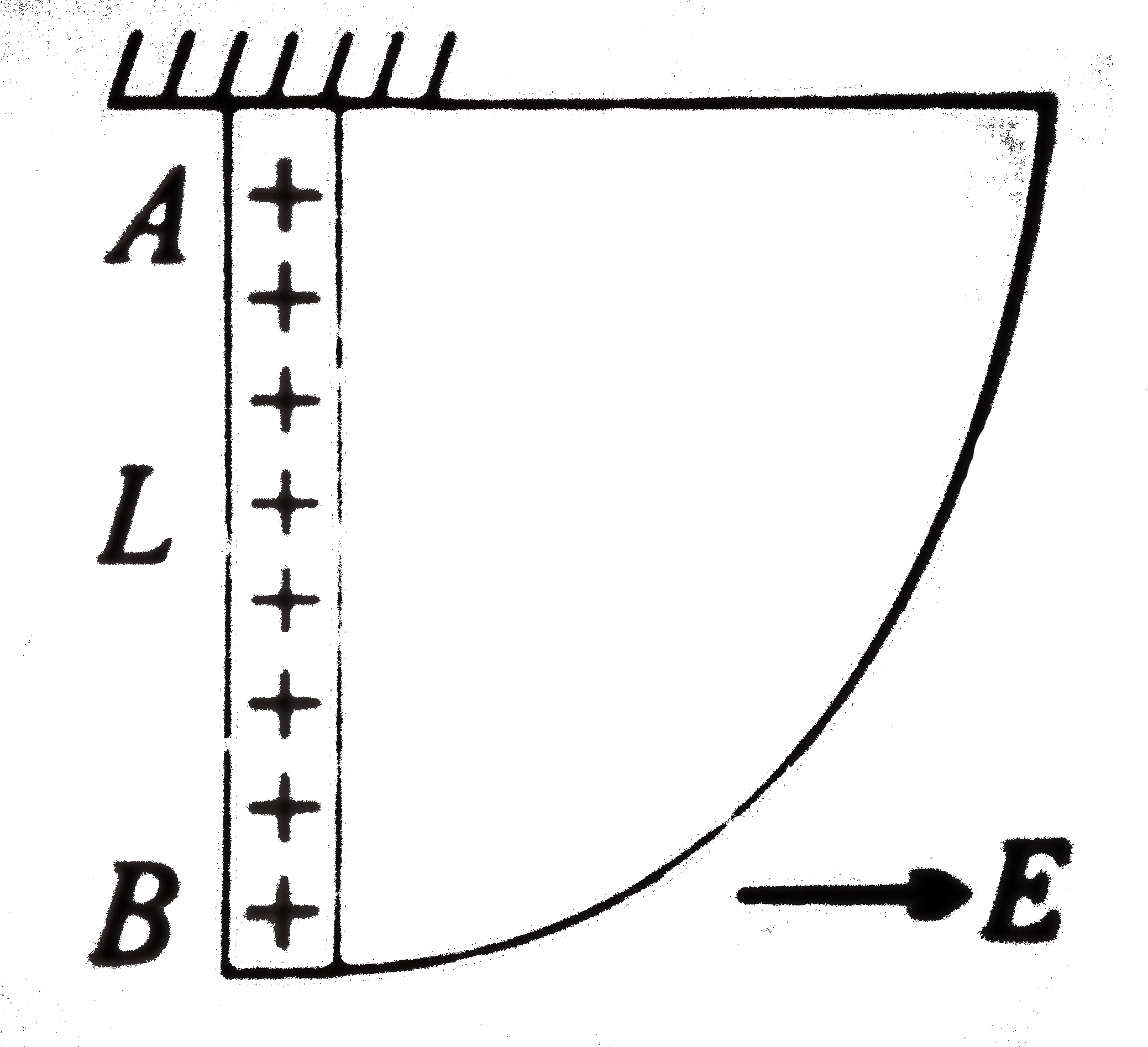 View Solution
View SolutionOne fourth length of a uniform rod of length 2l and mass m is place don a horizontal table and the rod is held horizontal. The rod is released from rest. Find the normal reaction on the rod as soon as the rod is released.
 View Solution
View SolutionA uniform rod of length l and mass 2 m rests on a smooth horizontal table. A point mass m moving horizontally at right angles to the rod with velocity v collides with one end of the rod and sticks it. Then
View SolutionA uniform rod of length l and mass 2 m rests on a smooth horizontal table. A point mass m moving horizontally at right angles to the rod with velocity v collides with one end of the rod and sticks it. Then
View SolutionA uniform rod of length L and mass M is pivoted freely at one end and placed in vertical position.
a. What is angular acceleration of the rod when it is at ann angle θ with the vertical?
b. What is the tangential linear acceleration of the free end when the rod is horizontal?View SolutionA uniform rod of mass m and length l is suspended about its end. Time period of small angular oscillations is
 View Solution
View SolutionA uniform thin rod of length l and mass m is hinged at a distance l/4 from one of the end and released from horizontal position as shown in Fig. The angular velocity of the rod as it passes the vertical position is
 View Solution
View Solution
A uniform rod AB of length L and mass m is suspended freely at A and hangs vertically at rest when a particle of same mass m is fired horizontally with speed v to strike the rod at its mid point. If the particle is brought to rest after the impact. Then the impulsive reaction at A is horizontal direction isView SolutionA rod of length l, has a uniform positive charge per unit length and a total charge Q. calculate the electric field at a point P located along the long axis of the rod and at a distance a from one end
 View Solution
View Solution
