A uniform rod of mass m and length 2a lies at rest on rotating with angular speed ω0=40rad/s is placed between two smooth walls on a rough ground. Distance between the walls is slightly greater than the lenght of the rod . Cofficent of friction between the rod and the ground is μ=0.1. rod will stop rotating after time t=.......s.
The correct Answer is:C
To solve the problem, we need to determine the time it takes for the uniform rod to stop rotating after being placed on a rough ground. Here's the step-by-step solution:
Step 1: Identify the given values
- Initial angular speed, ω0=40rad/s
- Coefficient of friction, μ=0.1
- Acceleration due to gravity, g=10m/s2
- Length of the rod, L=2a (not directly needed for calculations)
Step 2: Calculate the torque due to friction
The torque (τ) acting on the rod due to friction can be calculated using the formula:
τ=μmgr
where r is the radius of the rod. For a uniform rod, we can consider r to be half the length of the rod, which is a.
Step 3: Calculate the moment of inertia of the rod
The moment of inertia (I) of a uniform rod about its end is given by:
I=13mL2=13m(2a)2=43ma2
Step 4: Calculate the angular retardation (α)
Using the relationship between torque, moment of inertia, and angular acceleration:
α=τI
Substituting the values we have:
α=μmgr43ma2
Since r=a:
α=μmga43ma2=3μg4a
Substituting μ=0.1 and g=10:
α=3×0.1×104=34=0.75rad/s2
Step 5: Use the angular motion equation to find time (t)
We use the equation for angular motion:
ωf=ωi+αt
Where:
- ωf=0 (final angular velocity)
- ωi=40rad/s
- α=−0.75rad/s2 (negative because it's retardation)
Setting up the equation:
0=40−0.75t
Rearranging gives:
0.75t=40
Thus,
t=400.75=400075≈16s
Final Answer
The rod will stop rotating after approximately t=16s.
---
- Initial angular speed, ω0=40rad/s
- Coefficient of friction, μ=0.1
- Acceleration due to gravity, g=10m/s2
- Length of the rod, L=2a (not directly needed for calculations)
The torque (τ) acting on the rod due to friction can be calculated using the formula:
τ=μmgr
where r is the radius of the rod. For a uniform rod, we can consider r to be half the length of the rod, which is a.
The moment of inertia (I) of a uniform rod about its end is given by:
I=13mL2=13m(2a)2=43ma2
Using the relationship between torque, moment of inertia, and angular acceleration:
α=τI
Substituting the values we have:
α=μmgr43ma2
Since r=a:
α=μmga43ma2=3μg4a
Substituting μ=0.1 and g=10:
α=3×0.1×104=34=0.75rad/s2
We use the equation for angular motion:
ωf=ωi+αt
Where:
- ωf=0 (final angular velocity)
- ωi=40rad/s
- α=−0.75rad/s2 (negative because it's retardation)
0=40−0.75t
Rearranging gives:
0.75t=40
Thus,
t=400.75=400075≈16s
The rod will stop rotating after approximately t=16s.
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - More than one option is correct
36 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Comprehension Type Questions
40 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
Similar Questions
A solid sphere of mass 5 kg and and radius 1 m after rotating with angular speed ω0=40rad/s is placed between two smooth walls on a rough ground. Distance between the walls is slightely greater than the diameter of the sphere. Coffiecient of friction between the sphere and the ground is μ=0.1. sphere will stop rtotating after time t = ...........s .

View SolutionA disc of mass m and radius R rotating with angular speed ω0 is placed on a rough surface (co-officient of friction =μ). Then

View Solution
A rod of mass 2 kg ad length 2 m is rotating about its one end O with an angular velocity ω=4rad/s. Find angular momentum of the rod about the axis rotation.
View SolutionA uniform rod of mass m and length L lies radialy on a disc rotating with angular speed ω in a horizontal plane about vertical axis passing thorugh centre of disc. The rod does not slip on the disc and the centre of the rod is at a distance 2L from the centre of the disc. them the kinetic energy of the rod is

View Solution_2019_CBT4_E01_067_Q01.png)
A uniform rod of mass M and length L highed at centre is rotating in horizontal plane with angular speed ω0 now two object each of mass m are kept on rod near thhe hinge on both sides. They starts sliding towards ends. Find ω of rod finally
View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View SolutionA uniform rod of mass 300 g and length 50 cm rotates at a uniform angular speed of 2 rad/s about an axis perpendicular to the rod through an end. Calculate a. the angular momentum of the rod about the axis of rotation b. the speed of the centre of the rod and c. its kinetic energy.
View SolutionA rod of length 20 cm is rotating with angular speed of 100 rps in a magnetic field of strength 0.5 T about it’s one end . What is the potential difference betweet two ends of the rod :
View SolutionA uniform rod AB of mass m and length 2a is falling freely without rotation under gravity with AB horizontal. Suddenly the end A is fixed when the speed of the rod is v. The angular speed which the rod begains to rotate is
View SolutionAn L shaped uniform rod of mass 2M and length 2L(AB=BC=L) is held as shown in Fig. with a string fixed between C and wall so that AB is vertical and BC is horizontal. There is no friction between the hinge and the rod at A.
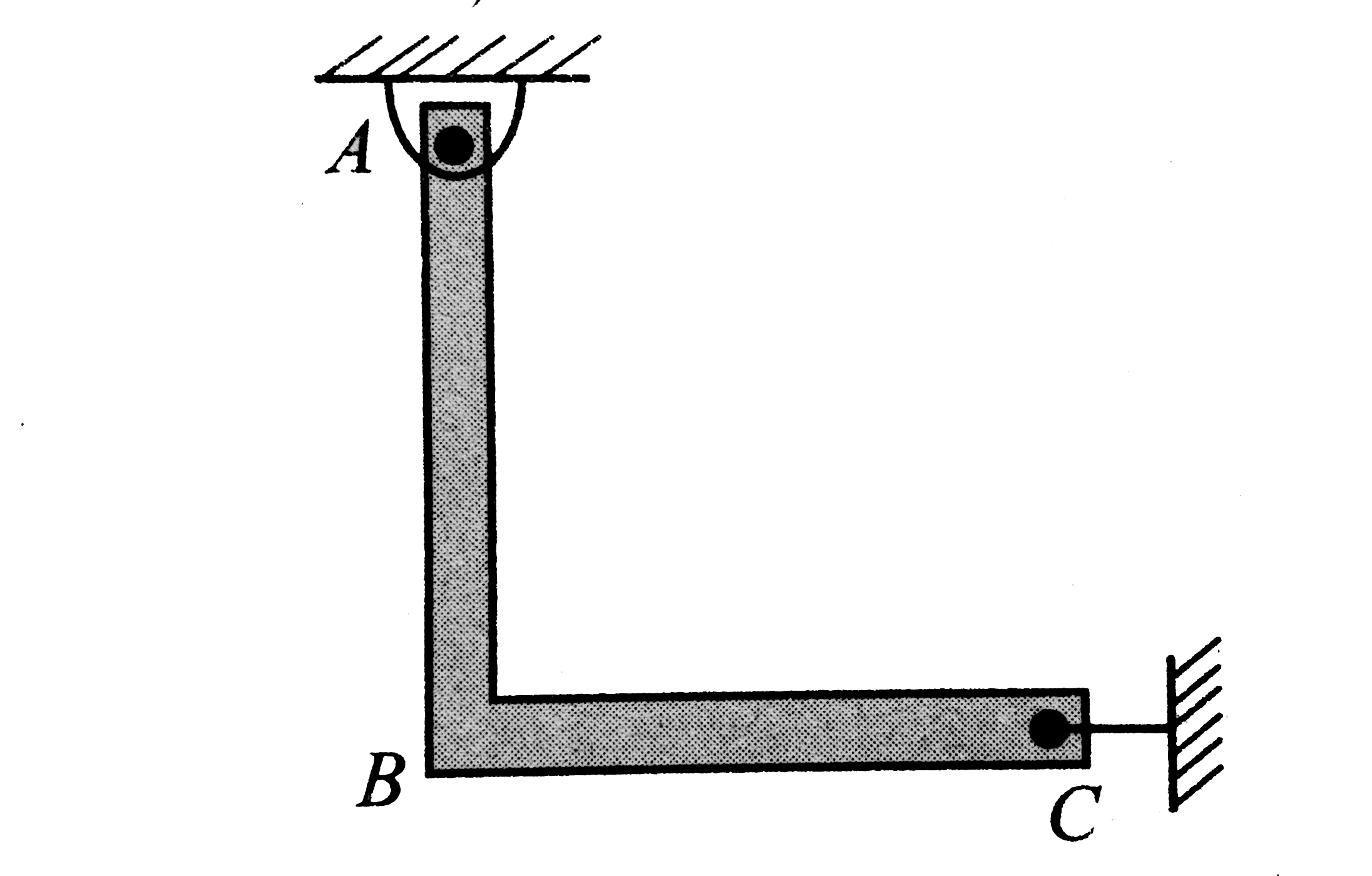 What will be the reaction between hinge and rod at point A?
What will be the reaction between hinge and rod at point A?
View Solution
