An ant with mass m is standing peacefully on top of a horizontal, stretched rope. The rope has mass per unit length μ and is under tension F. Without warning, a student starts a sinusoidal transverse wave of wavelength λ propagating along the rope. the motion of the rope is in a verticle plane. what minimum wave amplitude (in mm) will make the ant feel weightless momentarily? Assume that m is so small that the presence of the ant has no effect on the propagation of the wave.
[Given: λ=0.5m,μ=0.1kg/m,F=3.125N,takeg=π2]
To solve the problem, we need to find the minimum wave amplitude that will make the ant feel weightless momentarily. Let's break down the solution step by step.
Step 1: Understanding the Condition for Weightlessness
For the ant to feel weightless, the upward acceleration of the wave must equal the acceleration due to gravity. This means that the maximum acceleration of the wave must be equal to g.
Step 2: Maximum Acceleration of the Wave
The maximum acceleration amax of a wave on a rope is given by the formula:
amax=ω2A
where ω is the angular frequency and A is the amplitude of the wave.
Step 3: Angular Frequency
The angular frequency ω can be expressed in terms of the wave speed v and the wavelength λ:
ω=2πvλ
Step 4: Wave Speed
The speed v of a wave on a stretched rope is given by:
v=√Fμ
where F is the tension in the rope and μ is the mass per unit length of the rope.
Step 5: Substitute for v
Substituting the expression for v into the equation for ω:
ω=2πλ√Fμ
Step 6: Substitute ω into amax
Now substituting ω into the equation for maximum acceleration:
amax=(2πλ√Fμ)2A
This simplifies to:
amax=4π2Fλ2μA
Step 7: Set amax Equal to g
For the ant to feel weightless, we set amax=g:
4π2Fλ2μA=g
Step 8: Solve for Amplitude A
Rearranging the equation to solve for A:
A=gλ2μ4π2F
Step 9: Substitute Given Values
Now we substitute the given values into the equation:
- λ=0.5m
- μ=0.1kg/m
- F=3.125N
- g=π2
Substituting these values:
A=π2⋅(0.5)2⋅0.14π2⋅3.125
Step 10: Simplify
The π2 cancels out:
A=(0.5)2⋅0.14⋅3.125
Calculating (0.5)2=0.25:
A=0.25⋅0.14⋅3.125=0.02512.5=0.002m
Step 11: Convert to Millimeters
To convert meters to millimeters:
A=0.002m=2mm
Final Answer
The minimum wave amplitude that will make the ant feel weightless momentarily is:
2mm
For the ant to feel weightless, the upward acceleration of the wave must equal the acceleration due to gravity. This means that the maximum acceleration of the wave must be equal to g.
The maximum acceleration amax of a wave on a rope is given by the formula:
amax=ω2A
where ω is the angular frequency and A is the amplitude of the wave.
The angular frequency ω can be expressed in terms of the wave speed v and the wavelength λ:
ω=2πvλ
The speed v of a wave on a stretched rope is given by:
v=√Fμ
where F is the tension in the rope and μ is the mass per unit length of the rope.
Substituting the expression for v into the equation for ω:
ω=2πλ√Fμ
Now substituting ω into the equation for maximum acceleration:
amax=(2πλ√Fμ)2A
This simplifies to:
amax=4π2Fλ2μA
For the ant to feel weightless, we set amax=g:
4π2Fλ2μA=g
Rearranging the equation to solve for A:
A=gλ2μ4π2F
Now we substitute the given values into the equation:
- λ=0.5m
- μ=0.1kg/m
- F=3.125N
- g=π2
A=π2⋅(0.5)2⋅0.14π2⋅3.125
The π2 cancels out:
A=(0.5)2⋅0.14⋅3.125
Calculating (0.5)2=0.25:
A=0.25⋅0.14⋅3.125=0.02512.5=0.002m
To convert meters to millimeters:
A=0.002m=2mm
The minimum wave amplitude that will make the ant feel weightless momentarily is:
2mm
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Comprehension45videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct9videos
TRANSMISSION OF HEAT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
In figure the tension in the rope (rope is light) is
 View Solution
View SolutionA thick uniform rope of length L is hanging from a rigid support. A transverse wave of wavelength λ0 is set up in the middle of the rope. The wavelength of the wave as it reaches the top most point is
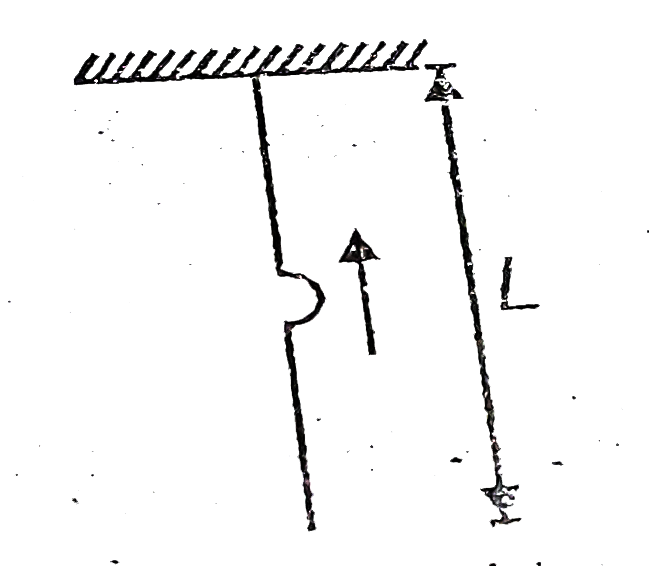 View Solution
View SolutionWhat is the speed of a transverse wave in a rope of length 10 m and mass 80 gm under a tension of 80 N?
View SolutionA transverse wave is propagating on a stretched string of mass per unit length32gm−1. The tension on the string is 80 N. The speed of the wave over the string is
View SolutionA unifrom rope of mass 0.1kg and length 2.45 m hangs from a rigid support. The time taken by the transverse wave formed in the rope to travel through the full length of the rope is (Assume g==9.8m/s2)
View SolutionA man of mass m is suspended in air by holding the rope of a ballon of mass M. As the man climbs up the rope, the ballon
 View Solution
View SolutionA man of mass m is suspended in air by holding the rope of a ballon of mass M. As the man climbs up the rope, the ballon
 View Solution
View SolutionA cart of mass M is tied to one end of a massless rope of length 10m. The other end of the rope is in the hands of a man of mass M. The entire system is on a smooth horizontal surface. The man is at x=0 and the cart at x=10m. If the man pulls the cart by the rope, the man and the cart will meet at the point
View SolutionA monkey of mass m clings a rope to a slung over a fixed pulley .The opposite end of the rope is tried to a weight of mass M tying on a horizontal table is μ Find the acceleration of weight .The monkey move downward with respect to the rope with an acceleration b.
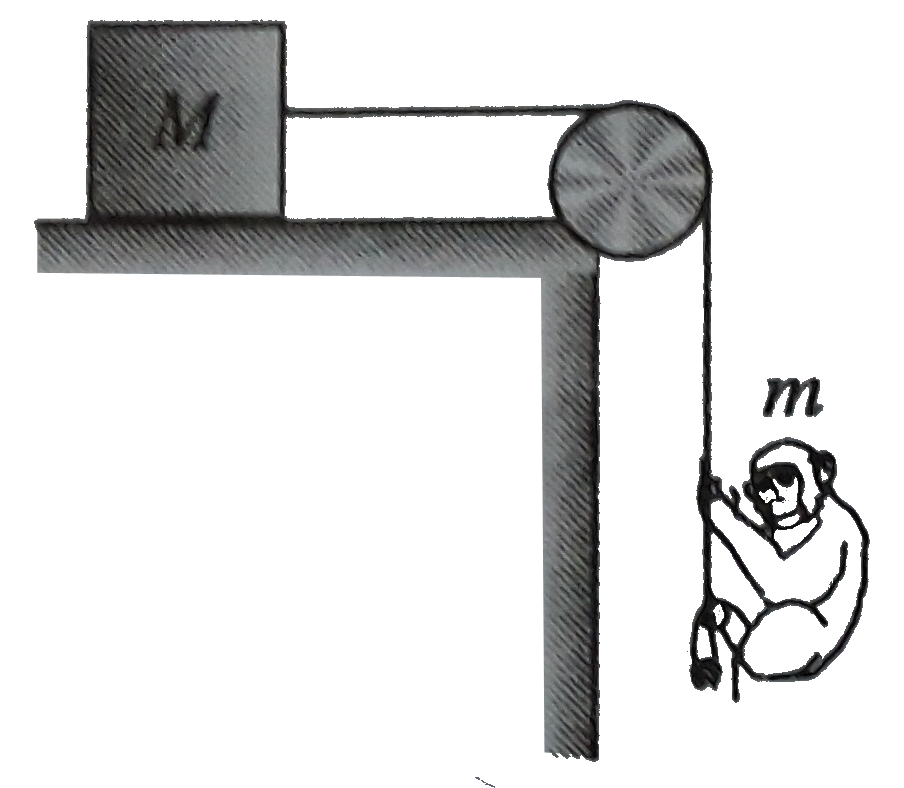
The acceleration of weight isView SolutionA rope of length L and mass m hangs freely from the ceiling. The velocity of transverse wave as a function of position x-along the rope is proportional to
View Solution

