An engine is attached to a wagon through a shock absorber of length 1.5 m. The system with a total mass of 40,000 kg is moving with a speed of 72kmh1 when the brakes tare applied to bring it to rest. In the process of the system being brought to rest, the spring of the shock absorber gets compressed by 1.0 m. If 90% of energy of the wagon is lost due to friction, the spring constant is ________ ×105 N/m.
To solve the problem, we will follow these steps:
Step 1: Convert the speed from km/h to m/s
The speed given is 72 km/h. We can convert this to meters per second using the conversion factor:
Speed in m/s=Speed in km/h×518
Calculating this:
Speed=72×518=20 m/s
Step 2: Calculate the initial kinetic energy of the system
The total mass of the system is given as 40,000 kg. The kinetic energy (KE) can be calculated using the formula:
KE=12MV2
Substituting the values:
KE=12×40000×(20)2=12×40000×400=8000000 J=8×106 J
Step 3: Determine the energy lost due to friction
According to the problem, 90% of the energy is lost due to friction. Therefore, the remaining energy (10% of the initial energy) can be calculated as:
Remaining Energy=0.1×KE=0.1×8×106=0.8×106 J
Step 4: Relate the remaining energy to the spring's potential energy
The energy stored in the spring when it is compressed can be expressed as:
Potential Energy in Spring=12kx2
Where k is the spring constant and x is the compression of the spring (1.0 m). Setting this equal to the remaining energy:
12k(1)2=0.8×106
This simplifies to:
12k=0.8×106
k=2×0.8×106=1.6×106 N/m
Step 5: Express k in the required format
The problem asks for the spring constant in the form x×105 N/m. We can express 1.6×106 as:
k=16×105 N/m
Final Answer
The spring constant k is 16×105 N/m.
---
The speed given is 72 km/h. We can convert this to meters per second using the conversion factor:
Speed in m/s=Speed in km/h×518
Calculating this:
Speed=72×518=20 m/s
The total mass of the system is given as 40,000 kg. The kinetic energy (KE) can be calculated using the formula:
KE=12MV2
Substituting the values:
KE=12×40000×(20)2=12×40000×400=8000000 J=8×106 J
According to the problem, 90% of the energy is lost due to friction. Therefore, the remaining energy (10% of the initial energy) can be calculated as:
Remaining Energy=0.1×KE=0.1×8×106=0.8×106 J
The energy stored in the spring when it is compressed can be expressed as:
Potential Energy in Spring=12kx2
Where k is the spring constant and x is the compression of the spring (1.0 m). Setting this equal to the remaining energy:
12k(1)2=0.8×106
This simplifies to:
12k=0.8×106
k=2×0.8×106=1.6×106 N/m
The problem asks for the spring constant in the form x×105 N/m. We can express 1.6×106 as:
k=16×105 N/m
The spring constant k is 16×105 N/m.
Topper's Solved these Questions
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics (Section A)81videos
JEE MAINS 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics (Section B)40videos
JEE MAINS 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics Section A39videos
JEE MAINS 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2020EXERCISE - PHYSICS246videos
JEE MAINS 2020
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - PHYSICS (SECTION -B)10videos
JEE MAINS 2022
Similar Questions
An engine is attahed to a wagon through a shock absorber of length 1.5m. The system with a total mass of 50,000kg is moving with a speed of 36kmh−1 when the brakes are applied to bring it to rest. In the process of the system being brought to rest, the spring of the shock absorber gets compressed by 1.0m. If 90% of energy of the wagon is lost due to friction, calculate the spring constant.
View SolutionA car having a mass of 1000 kg is moving at a speed of 30metres/sec. Brakes are applied to bring the car to rest. If the frictional force between the tyres and the road surface is 5000 newtons, the car will come to rest in
View Solution
Knowledge Check
- Question 1 - Select One
A railway engine (mass 104 kg) is moving with a speed of 72 km/h. The force which should be applied to bring it to rest over a distance of 20m is
A7200NB1000NC3600ND100000N - Question 2 - Select One
A motorcycle is being driven at a speed of 20 m/s when brakes are applied to bring it to rest in five seconds. The deacceleration produced in this case will be :
A+4m/s2B−4m/s2C+0.25m/s2D−0.25m/s2 - Question 3 - Select One
A block of mass 1kg moving with a speed of 4ms−1, collides with another block of mass 2kg which is at rest. The lighter block comes to rest after collision. The loss in KE of the system is
A8 JB4×10−7JC4 JD0 J
A car was movig at a rate of 18kmh−1 . When the brakes were applied, it comes to rest in a distance of 100 m. Calculate the retardation produced by the brakes.
View SolutionThe block shown in figure is released from rest. Find out the speed of the block when the spring is compressed by 1 m
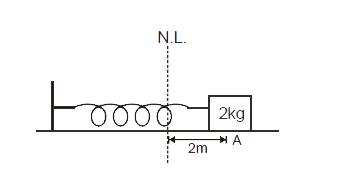 View Solution
View SolutionIn the above example, what is (i) the speed of te mass when the spring is compressed by 1.0 cm, (ii) potential energy of the mass when it momentarily comes to rest, (iii) total energy of the oscillating mass.
View SolutionA car is moving at a speed of 72kmh−1. The diameter of its wheel is 0.50m. If the wheels are stopped in 20 rotations by applying brakes, calculate the angular retardation produced by the brakes.
View SolutionWhen a car driver travelling at a speed of 10 m/s applies brakes and brings the car to rest in 20 s, then retardation will be :
View Solution

