An equilateral triangle ABC if formed by two Cu rods AB and BC and one Al rod
![An equilateral triangle ABC if formed by two Cu rods AB and BC and one Al rod it is heated in such a way that temperature of each rod increases by Delta T. Find change in the angle ABC. [Coeff. Of linear expansion for Cu. is alpha1, Coeff. of linear expansion for Al is alpha2]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/PR_XI_V02_C07_S01_742_Q01.png)
it is heated in such a way that temperature of each rod increases by ΔT. Find change in the angle ABC. [Coeff. Of linear expansion for Cu. is α1, Coeff. of linear expansion for Al is α2]
Consider the diagram shown
Let l1=AB,l2=AC,l3=BC
∴ cosθ=l23+l21−l222l3l1 (assume∠ABC=θ)
⇒ 2l3l1cosθ=l23+l21−l22
Differentiating 2 (l3dl1+l1dl3)cosθ−2l1l3sinθdθ
=2l3dl1+2l1dl1−2l2dl2
Now, dl1=l1α1Δt (where, Δt=change in temperature)
dl2=l2α1Δt⇒dl3=l3α2Δt
and l1=l2=l3=l
(l2α1Δt+l2α1Δt)cosθ+l2sinθdθ=l2α1Δt+l2α1Δt−l2α2Δt
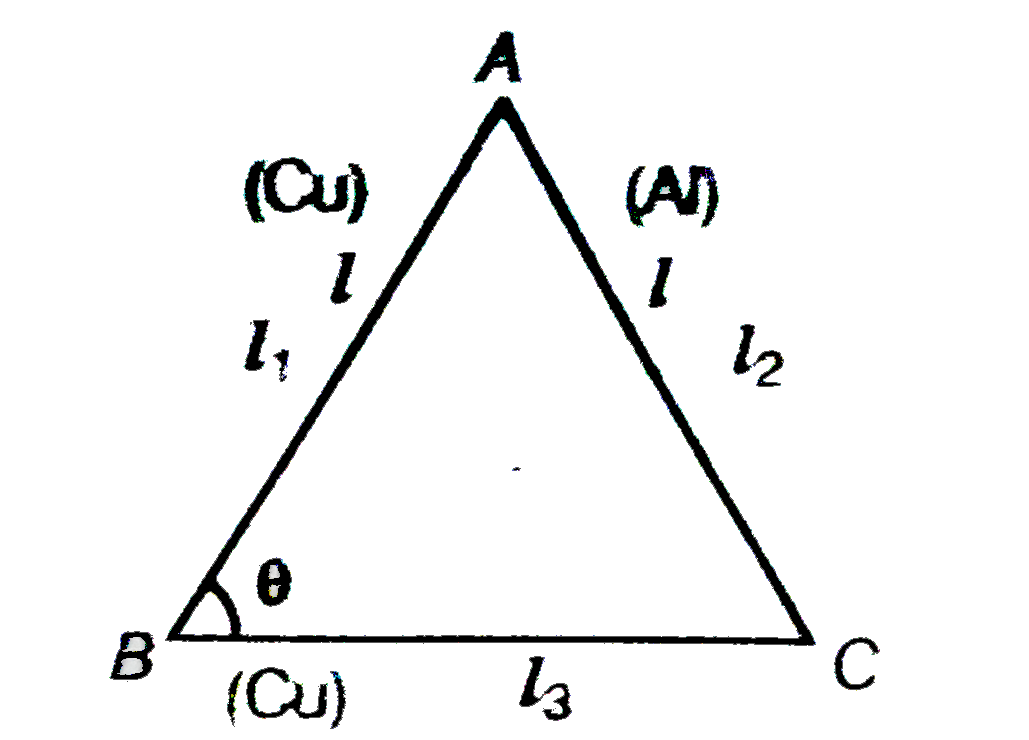
sinθdθ=2α1Δt(1−cosθ)−α2Δt
Putting θ=60∘ (for equilateral triangle)
dθ×sin60∘=2α1Δt(1−cos60∘)−α2Δt
=2α1Δt×12−α2Δt=(α1−α2)Δt
⇒ dθ=change in the angle ∠ABC
=(α1−α2)ΔTsin60∘=2(α1−α2)ΔT√3 (∵Δt=ΔT given)
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LONG SHORT ANSWER TYPE QUESTION
16 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Long Answer Type Questions
3 Videos
- MOTION IN A PLANEBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Multiple Choice Questions
37 Videos
Similar Questions
A meter scale calibarated for temperature T is used to measure the length of a steel rod. Length of steel rod measured by metal scale at temperature 2T is L. Length of the rod measured by metal scale at temperature T is [αs:Coefficient of linear expansion of metal scale, αR : Coefficient of linear expansion of steel rod]
View SolutionThe moment of inertia of a rod about its perpendicular bisector is l, when temperature of rod is increased by ΔT, the increase in the moment of inertia of the rod about same axis (γ = temperature coefficient of volume expansion)
View Solution
Knowledge Check
The moment of inertia of a rod about its perpendicular bisector is I . When the temperature of the rod is increased by ΔT , the increase in the moment of inertia of the rod about the same axis is (Here , α is the coefficient of linear expansion of the rod )
AαIΔTB2αIΔTCαIΔT2D2IΔTαAt 50∘C , a brass rod has a length 50 cm and a diameter 2 mm . It is joined to a steel rod of the same length and diameter at the same temperature . The change in the length of the composite rod when it is heated to 250∘C is (Coefficient of linear expansion of brass = 2.0×10−5∘C−1 , coefficient of linear expansion of steel = 1.2×10−5∘C−1)
A0.28 cmB0.30 cmC0.32 cmD0.34 cm
When the temperature of a rod increases from t to r+Δt, its moment of inertia increases from I to I+ΔI. If α is the value of ΔI/I is
View SolutionA compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temeprature increass by ΔT. The net change in its length is zero. Let l be the length of the rod, A its area of cross-section, Y its Young's modulus, and α its coefficient of linear expansion. Then, F is equal to
View SolutionA compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temeprature increass by ΔT. The net change in its length is zero. Let l be the length of the rod, A its area of cross-section, Y its Young's modulus, and α its coefficient of linear expansion. Then, F is equal to
View SolutionTwo metallic rods of length l and 3l have coefficient of linear expansion α and 3α respectively. The coefficient of linear expansion ofr their series combinations, is
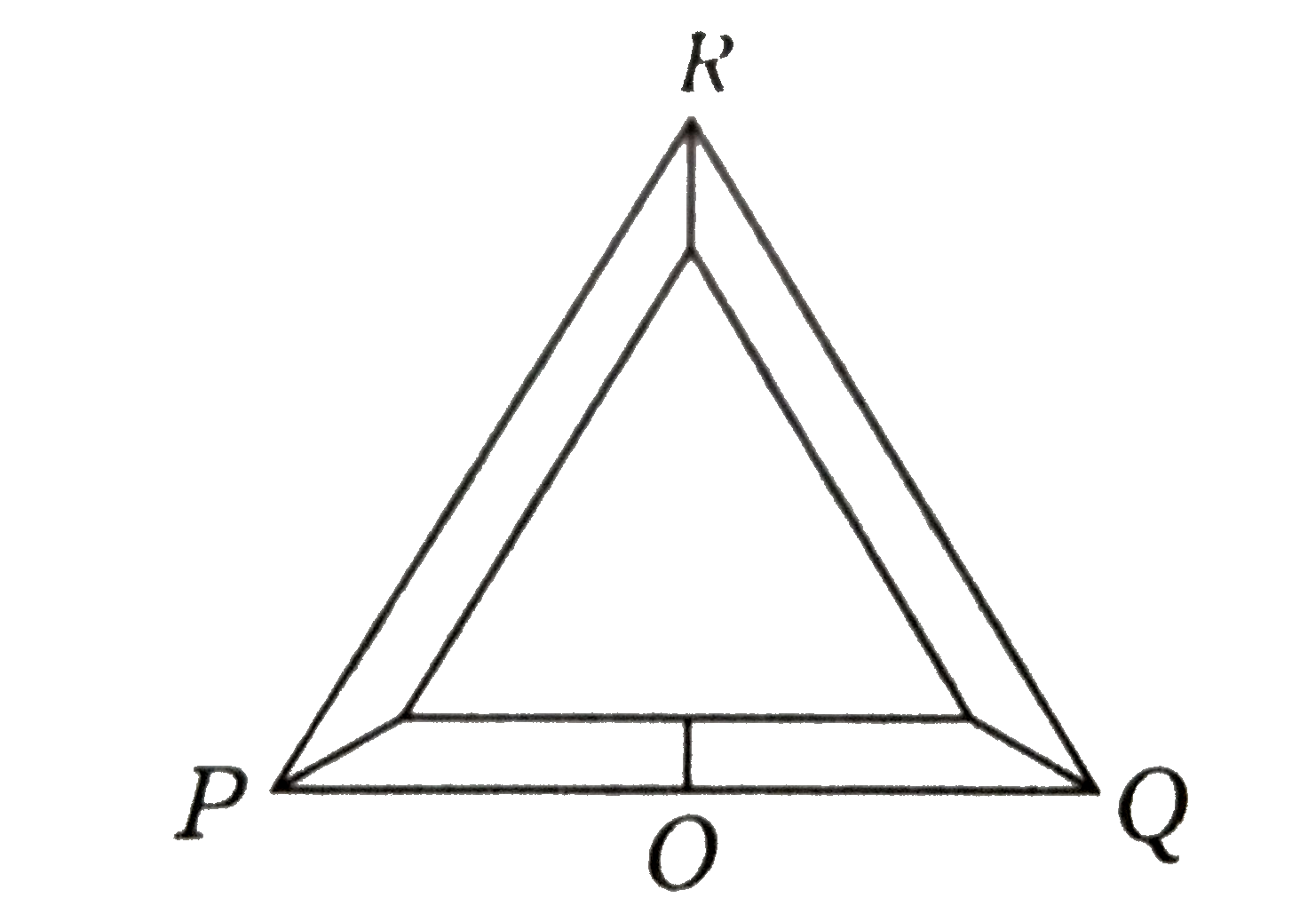
View SolutionThree rods of equal length are joined to from an equilateral triangle PQR.O is the mid-point of PQ. Distance OR remains same for small change in temperature. Coefficient of linear expansion for PR and RQ is same i.e., α2 but for PQ is α1. Then
View SolutionOn heating the metal rod of length 2m from 0°C to 50°C, its length is increased by 0.1 cm. the coeffecient of linear expansion of metal rod is
View Solution
