An O16 nucleus is spherical and has a radius R and a volume V=43πR3.According to the empirical observation, the volume of the .54X128 nucleus assumed to be sphericla is V' and radius is R'. Then
V'=8V
V'=2V
R'=2R
R'=8R
The correct Answer is:a,c
R=R0A1/3
For O16,R=R0(16)1/3
For .54X128,R'=R0(16)1/3
R'=R=0(128)1/3
R'=(12816)1/3R=2R
∴V'=43πR13=8V.
Topper's Solved these Questions
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICSCHAPTER - NUCLEAR PHYSICSEXERCISE - Linked Comprehension
29 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICSCHAPTER - NUCLEAR PHYSICSEXERCISE - Integer
6 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICSCHAPTER - NUCLEAR PHYSICSEXERCISE - Subjective
35 Videos
- Moving charges and magnetismBOOK - CENGAGE PHYSICSCHAPTER - Moving charges and magnetismEXERCISE - Question Bank
20 Videos
- NUCLEIBOOK - CENGAGE PHYSICSCHAPTER - NUCLEIEXERCISE - QUESTION BANK
59 Videos
Similar Questions
Knowledge Check
The volume of a sphere is given by V=43πr3 Make r as the subject of the formula.
A√3V4πB3V4πC(3Vπ)13D(3V4π)13The volume of a cone is V=13πr2h. Make r as the subject of the formula.
Ar=√3V2πhBr=√V3πhCr=√3VπhDr=√V3πhA sphere of radius r has the same volume as that of a cone with a circular base of radius r. Find the height of the cone.
A2rBr3C4rD(23)r
If a sphere of radius 2r has the same volume as that of a cone with circular base of radius r, then find the height of the cone.
View SolutionA cylinder is inscribed in a sphere of radius R. The volume V of the cylinder is written as v=f(x),wherex is the height of the cylinder
View SolutionUsing the method of integration, show that the volume of a right circular cone of base radius r and height h is V=13πr2h.
View SolutionThe nuclear charge (Ze) is non uniformlly distribute with in a nucleus of radius r. The charge density ρ(r) (charge per unit volume) is dependent only on the radial distance r form the centre of the nucleus s shown in figure. The electric field is only along the radial direction.
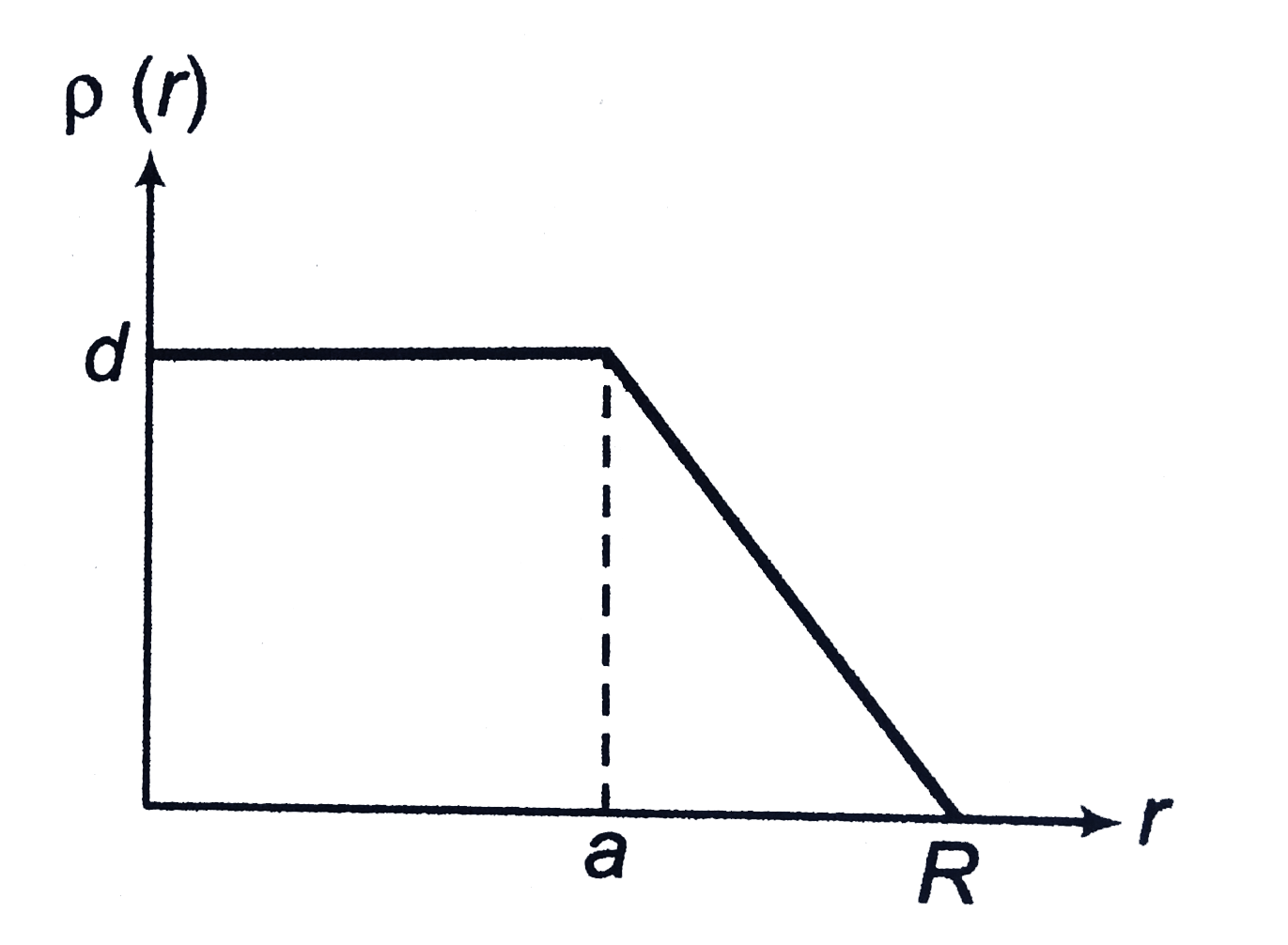
The electric field at r=R is
View SolutionThe volume of a sphere is given by V=43πr3 Make r as the subject of the formula.
View Solution
