Assertion: If we see along the axis of a chaged ring, the magnitudeof electric field is minimum at centre and magnitude of electridc potential is maximum.
Reason: Electric field is a vector quantity while electric potential is scalar.
If both Assertion and Reason are true but Reason is the correct explanation of Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true, but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:B
To solve the given question, we need to analyze both the assertion and the reason provided.
1. Understanding the Assertion:
The assertion states that when observing along the axis of a charged ring, the electric field is minimum at the center, while the electric potential is maximum at that point.
2. Electric Potential Calculation:
The electric potential V at a point along the axis of a charged ring can be expressed as:
VP=14πϵ0Q√r2+x2
where Q is the total charge on the ring, r is the radius of the ring, and x is the distance from the center of the ring along the axis.
3. Finding Maximum Potential:
At the center of the ring, when x=0:
VP=14πϵ0Qr
This shows that the potential is maximum at the center of the ring since moving away from the center (increasing x) will increase the denominator and thus decrease VP.
4. Electric Field Calculation:
The electric field E at the center of the charged ring can be analyzed by considering the contributions from each infinitesimal charge element on the ring. Due to symmetry, the horizontal components of the electric field from opposite charges will cancel out.
5. Finding Minimum Electric Field:
At the center of the ring, the net electric field E is:
E=0
This indicates that the electric field is indeed minimum (zero) at the center of the ring.
6. Conclusion on Assertion:
Since the electric field is minimum (zero) at the center and the electric potential is maximum at that point, the assertion is correct.
7. Understanding the Reason:
The reason states that the electric field is a vector quantity while the electric potential is a scalar quantity. This is a true statement.
8. Evaluating the Reason as Explanation:
However, while the reason is correct, it does not directly explain why the electric field is minimum and the potential is maximum at the center of the ring. The cancellation of electric field vectors due to symmetry is the key point, which is not addressed by the reason.
9. Final Evaluation:
Therefore, both the assertion and reason are correct, but the reason does not correctly explain the assertion.
Final Answer:
The correct option is that the assertion is true, the reason is true, but the reason is not the correct explanation of the assertion.
---
The assertion states that when observing along the axis of a charged ring, the electric field is minimum at the center, while the electric potential is maximum at that point.
The electric potential V at a point along the axis of a charged ring can be expressed as:
VP=14πϵ0Q√r2+x2
where Q is the total charge on the ring, r is the radius of the ring, and x is the distance from the center of the ring along the axis.
At the center of the ring, when x=0:
VP=14πϵ0Qr
This shows that the potential is maximum at the center of the ring since moving away from the center (increasing x) will increase the denominator and thus decrease VP.
The electric field E at the center of the charged ring can be analyzed by considering the contributions from each infinitesimal charge element on the ring. Due to symmetry, the horizontal components of the electric field from opposite charges will cancel out.
At the center of the ring, the net electric field E is:
E=0
This indicates that the electric field is indeed minimum (zero) at the center of the ring.
Since the electric field is minimum (zero) at the center and the electric potential is maximum at that point, the assertion is correct.
The reason states that the electric field is a vector quantity while the electric potential is a scalar quantity. This is a true statement.
However, while the reason is correct, it does not directly explain why the electric field is minimum and the potential is maximum at the center of the ring. The cancellation of electric field vectors due to symmetry is the key point, which is not addressed by the reason.
Therefore, both the assertion and reason are correct, but the reason does not correctly explain the assertion.
The correct option is that the assertion is true, the reason is true, but the reason is not the correct explanation of the assertion.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Objective
82 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.7
2 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
Assertion Due to two charges electric field and electric potential cannot be zero at some point simultaneously.
Reason Field is a vector quantity and electric potential is a scalar quantity
View SolutionAt what angle of electric dipole moment with uniform electric field, potential energy is maximum ?
View Solution
Knowledge Check
In case of linearly polarised light, the magnitude of the electric field vector
Ais parallel to the direction of propagationBdoes not change with timeCincreases linearly with timeDvaries perodically with time
Electric field lines are shown in figure. State whether the electric potential is greater at A or B.
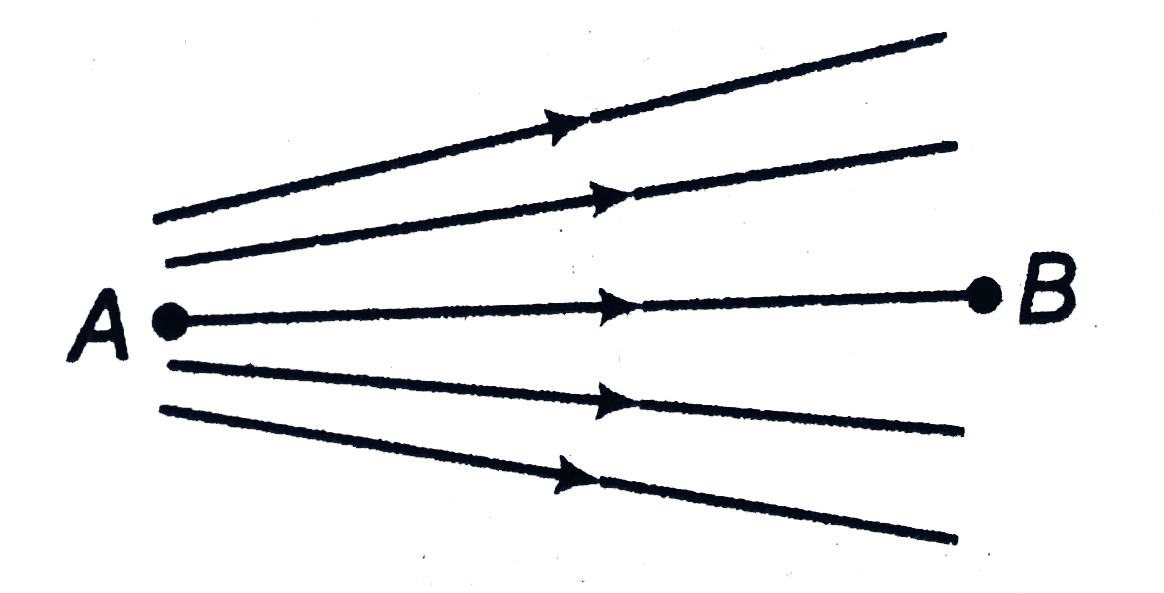
View SolutionAssertion : Due to two point charges electric field and electric potential can't be zero at some point simultaneously.
Reason : Field is a vector quantity and potential a scalar quantity.
View SolutionAssertion : Electric potential of earth is taken zero.
Reason : No electric field exists on earth surface.
View SolutionAlong the axis of a dipole, direction of electric field is always in the direction of electric dipole moment p. Is this statement true of false?
View SolutionAssertion: Electric field is aways directed perpendicular to an equipotential surface.
Reason: Equipotential surface is a surface on which at each point potential is same.
View SolutionIn a certain region of space, the electric field is zero. From this, we can conclude that the electric potential in this region is:
View SolutionThe electric field and the electric potential at a point are E and V respectively.
View Solution
