By using a concave mirror, image of a tree is focused on a screen. The distance between the screen and the mirror is _____
equal to radius of curvature of the mirror.
equal to half the radius of curvature of the spherical mirror.
equal to twice the focal length of the mirror.
equal to one fourth the focal length of the mirror.
The correct Answer is:B
To solve the question about the distance between the screen and the concave mirror when the image of a tree is focused on the screen, we can follow these steps:
1. Understanding the Object Position:
- The question states that the image of a tree is focused on a screen using a concave mirror. Since a tree is a large object located far away, we can consider it as being at infinity.
Hint: Remember that when an object is at infinity, the light rays coming from it are parallel.
2. Behavior of Light Rays:
- When parallel light rays from an object at infinity strike a concave mirror, they converge at the focus (F) of the mirror after reflection.
Hint: Think about how parallel rays behave when they hit a concave mirror.
3. Image Formation:
- For a concave mirror, when the object is at infinity, the image is formed at the focus (F). This is a key property of concave mirrors.
Hint: Recall the relationship between the object position and the image position for concave mirrors.
4. Distance Between Screen and Mirror:
- Since the image of the tree is formed at the focus, the distance between the screen (where the image is focused) and the mirror is equal to the focal length (f) of the mirror.
Hint: The screen must be placed at the location where the image is formed, which is the focus in this case.
5. Relating Focal Length to Radius of Curvature:
- The focal length (f) of a concave mirror is related to its radius of curvature (R) by the formula:
f=R2
Hint: Remember this relationship to understand the significance of the focal length in relation to the radius of curvature.
6. Conclusion:
- Therefore, the distance between the screen and the mirror is equal to the focal length (f) of the mirror.
Final Answer:
The distance between the screen and the mirror is equal to the focal length (f) of the concave mirror.
- The question states that the image of a tree is focused on a screen using a concave mirror. Since a tree is a large object located far away, we can consider it as being at infinity.
- When parallel light rays from an object at infinity strike a concave mirror, they converge at the focus (F) of the mirror after reflection.
- For a concave mirror, when the object is at infinity, the image is formed at the focus (F). This is a key property of concave mirrors.
- Since the image of the tree is formed at the focus, the distance between the screen (where the image is focused) and the mirror is equal to the focal length (f) of the mirror.
- The focal length (f) of a concave mirror is related to its radius of curvature (R) by the formula:
f=R2
- Therefore, the distance between the screen and the mirror is equal to the focal length (f) of the mirror.
The distance between the screen and the mirror is equal to the focal length (f) of the concave mirror.
Topper's Solved these Questions
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - Concept Application (Level 2)
20 Videos
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - Concept Application (Level 3)
15 Videos
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - Concept Application (Level 1) Matching
1 Videos
- KINEMATICS BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - KINEMATICS EXERCISE - CONCEPT APPLICATION (Level 3) (Application Based Questions )
5 Videos
- MAGNETISM BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MAGNETISM EXERCISE - CONCEPT APPLICATION (Level 3)
4 Videos
Similar Questions
Two times magnified image is formed by a concave mirror when an object is placed 30 cm in front of the mirror. The distance of image from the mirror is __________.
View SolutionAn object is placed midway between a concave mirror of focal length f and a convex mirror of focal length f. The distance between the two mirrors is 6f. Trace the ray that is first incident on the concave mirror and then the convex mirror.
View Solution
Knowledge Check
Four students A, B, C and D performed the experiment to determine the focal length of a concave mirror by obtaining the image of a distant tree on a screen. They measured the distances between the screen and the mirror as shown in the diagrams given below :
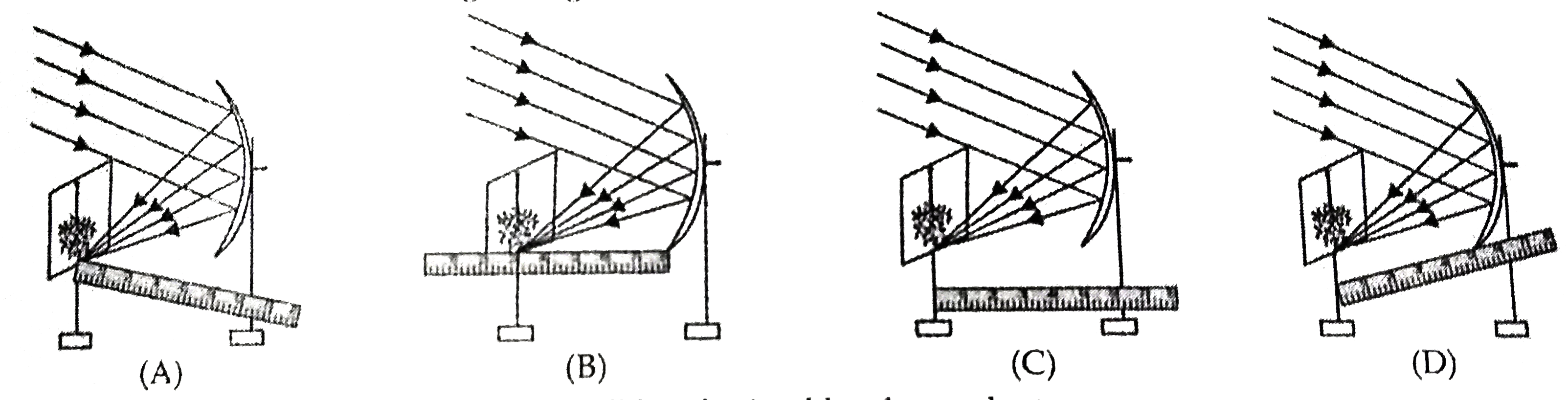
The correct value for focal length will be obtained by the student :AABBCCDDArrange the following steps in a sequential order to determine the focal length of a concave mirror by distant object method.
(A) Place a white screen in front of the mirror and adjust the position of the screen until a sharp images is formed.
(B) Focus the mirror towards a distant object.
(C) Mount the concave mirror on a mirror stand.
(D) Measure the distance between the screen and the mirror. This gives the focal length.AC A B DBC B A DCB A C DDA B D CArrange the following steps of an experiment in sequential order to calculate the focal length of a concave mirror when the object is at a small distance.
(A) Mount a concave mirror on the mirror stand and place an object in front of it at a certain distance.
(B) Adjust the position of the screen and the mirror until a bright and sharp image is obtained on the screen.
(C) Measure the distance between the object and the mirror. This distance gives the object distance u.
(D) Place the screen between the object and mirror but not in line with them.
(E) Measure the distance between the concave mirror and the screen. This gives the image distance v.
(F) The focal length of the given concave mirror can be found out using the mirror formula with proper signs.
(G) Repeat the experiment for different values of u and v and tabulate the results.AA D B C E G FBA C E B D G FCA B C D E F GDA B D E C G E
Try this
Material: Convex lens, screen, metre scale, stand for the lens etc.
Method:
Keeping the screen fixed, obtain a clear image of a distant object like a tree or a building with the help of the lens on the screen. Measure the distance between the screen and the lens with the help of the metre scale. Now tum the other side of the lens towards the screen. Again obtain a clear image of the distant object on the screen by moving the lens forward or backward. Measure the distance between the screen and the lens again.
What will happen if you use a concave lens in this experiment?
View SolutionAn object is placed at a distance u from a concave mirror and its real image is received on a screen placed at a distance of v from the mirror. If f is the focal length of the mirror, then the graph between 1/v versus 1/u is
View SolutionA concave mirror forms an image of the sun at a distance of 12 cm from it.
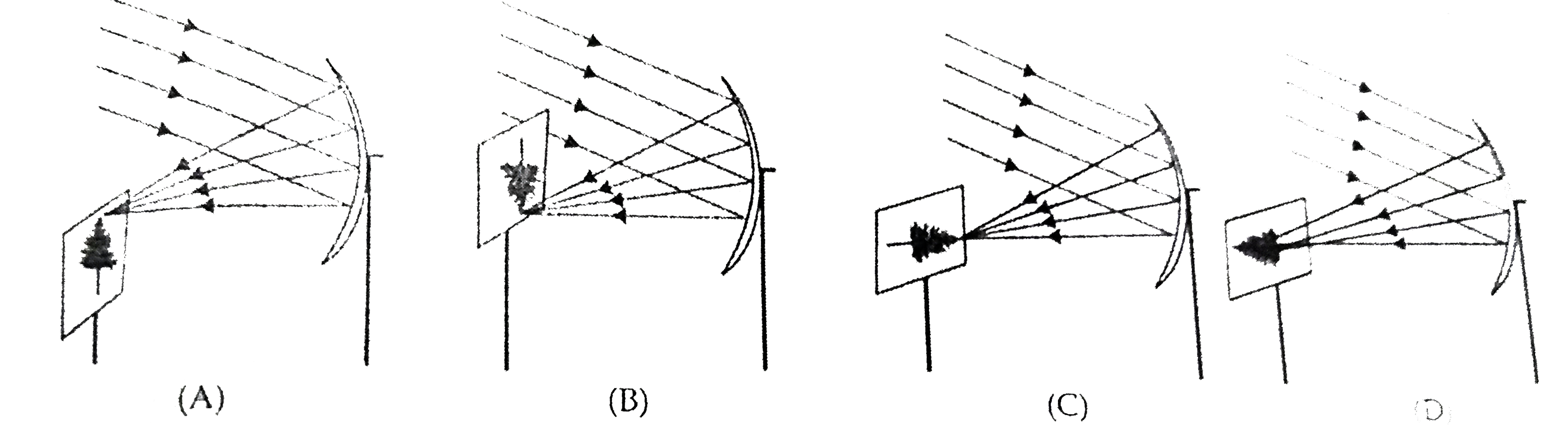
View SolutionThe parallel rays form the top of a distant tree are incident on a concave mirror forming its image on a screen.
The diagram which shows the image of the tree on the screen correctly is :
View SolutionA teacher gives a convex lens and a concave mirror of focal length of about 20 cm each to his student and asks him to find their focal lengths by obtaining the image of a distant object.The student uses a distant tree as the object and obtains its sharp image, one by one, on a screen. The distances d1 and d2 between the lens/mirror and the screen in the two cases and the nature of their respective sharp images are likely to be :
View Solution
