Calculate the gravitational field intensity and potential at the centre of the base of a solid hemisphere of mass m, radius R
We consider the shaded elemetnal disc of radius Rsinθ and thickness Rdθ
its mass
dM=M23πR3π(Rsinθ)2(Rdθsinθ)
or dM=3M2sin3θdθ
Field due to this plate at O,
dE=2GdM(1−cosθ)(Rsinθ)2 (see field due to a uniform disc)
or dE=3GMsinθ(1−cosθ)dθR2
Therefore, E=∫π20dE=∫π20=3GMsinθ(1=cosθ)R2dθ
=3GMR2[−cosθ+cos2θ2](p)i20
or E=3GM2R2
Now potential due to the element under consider at the cente of the baase of the hemisphere
dV=−2GdM/r(cosecθ−cotθ)
(See potential due to a circular plate)
or dV=−3GMsin3θ(cosecθ−cotθ)dθ(Rsinθ)
Therefore, V=−3GMR∫π20(sinθ−cosθsinθ)dθ
=−3GMR[−cosθ+cos2θ2]π20
or V=−3GM2R
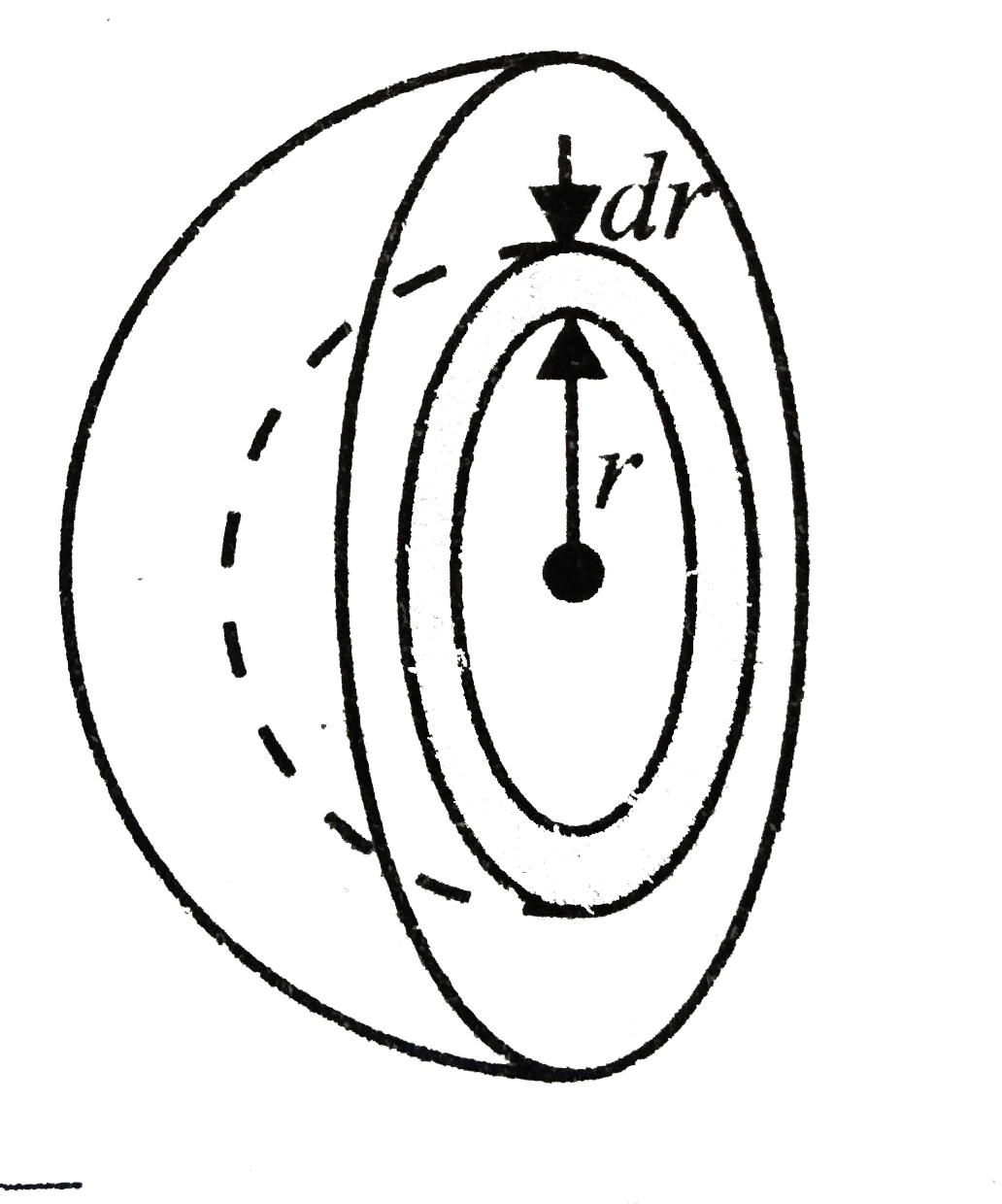
Alternative method: Consider a hemispherical shell of radius r and thicknes dr
It mass
dm=M23πR3(2π2dr) or dm=3Mr2drR3
Since all points of this hemispherical shell are at the same distnce r from O hence potential at O is
dV=−Gdmr=−3GMrdrR3
∴V=∫R0dV=−3GM2R
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Exercise 6.113videos
GRAVITATION
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Exercise 6.215videos
GRAVITATION
- BOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE1videos
FLUID MECHANICS
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer9videos
KINEMATICS-1
Similar Questions
Calculate the gravitational field intensity at the centre of the base of a hollow hemisphere of mass M and radius R. (Assume the base of hemisphere to be open)
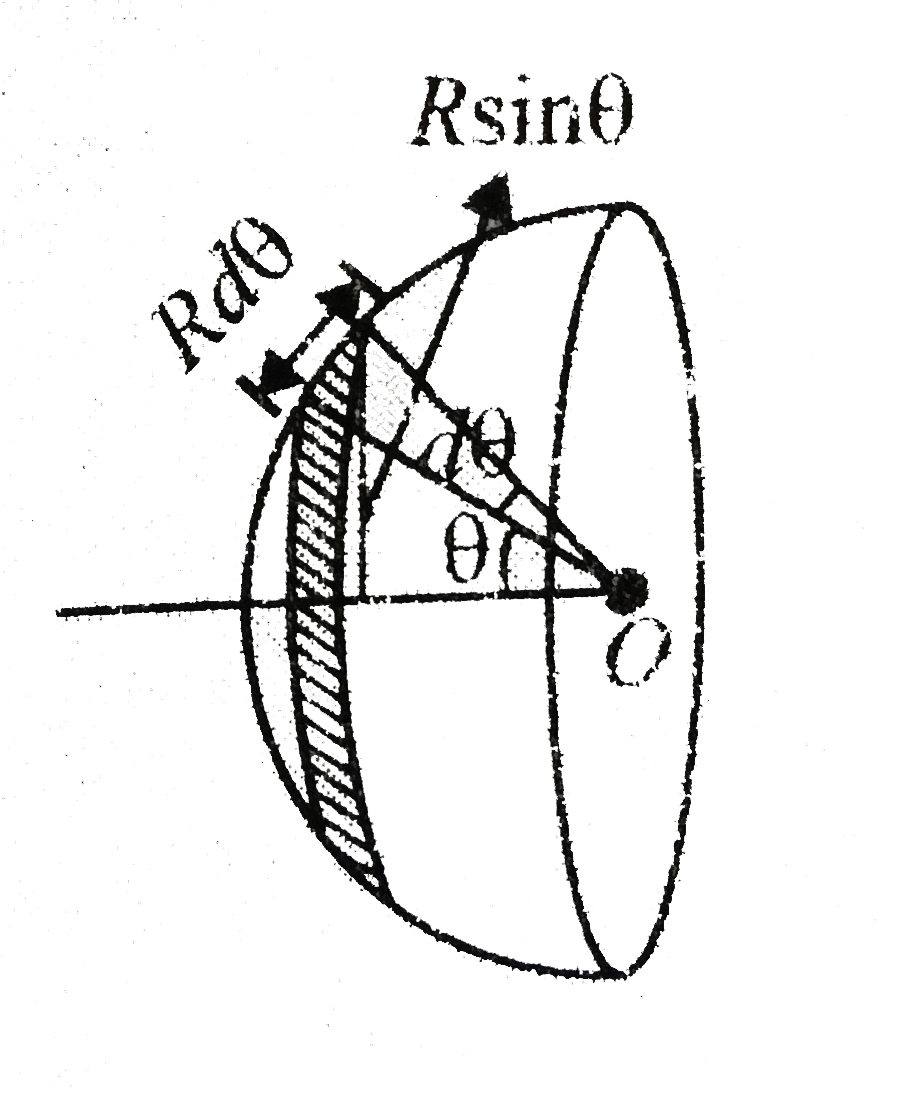 View Solution
View SolutionCalculate the gravitational field intensity at the centre of the base of a hollow hemisphere of mass M and radius R. (Assume the base of hemisphere to be open)
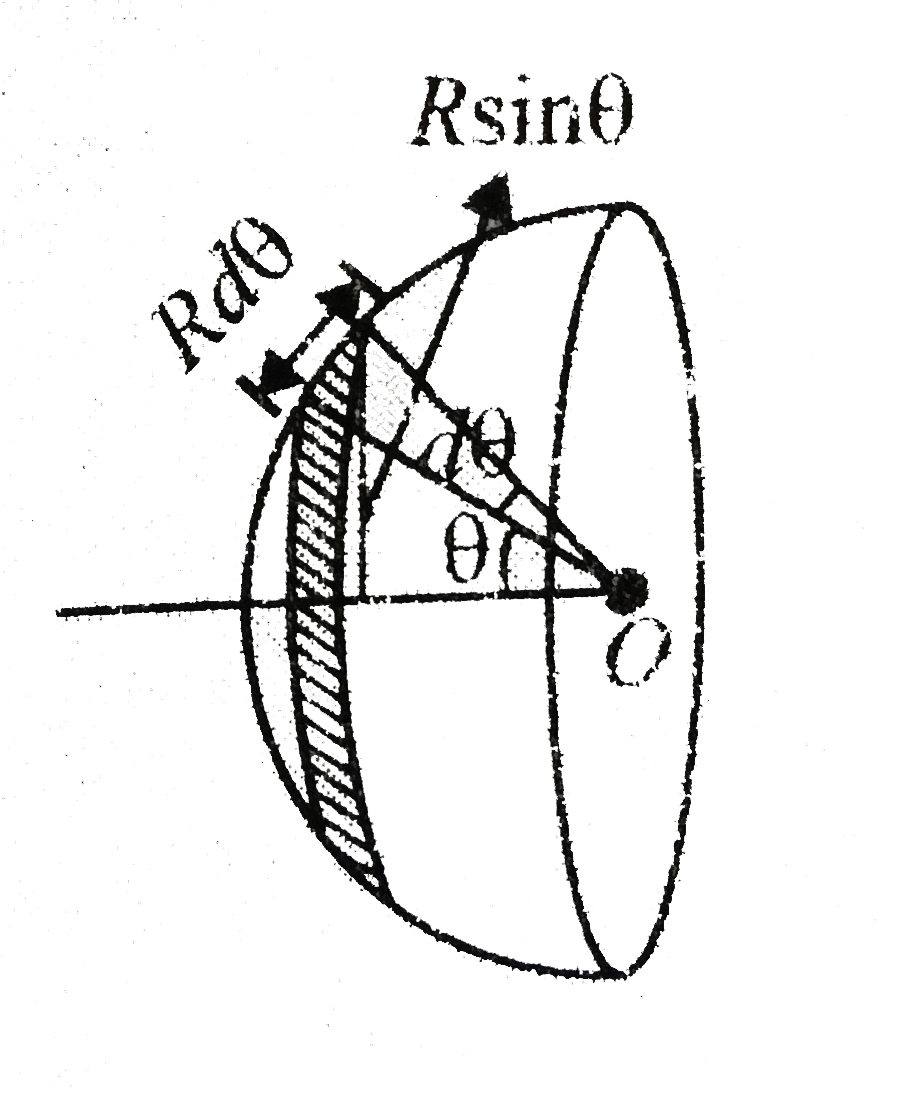 View Solution
View SolutionCalculate the gravitational potential at the centre of base of a solid hemisphere of mass M, radius R.
View SolutionThe centre of gravity of a solid hemisphere of radius a is
View SolutionThe centre of gravity of a solid hemisphere of radius a is : (A)
View SolutionDetermine the centre of mass of a uniform hemisphere of radius R.
View SolutionDetermine the centre of mass of a uniform hemisphere of radius R.
View SolutionCalculate gravitational field intensity at a distance x on the axis from centre of a uniform disc of mass M and radius R .
View SolutionDetermine the position of the centre of mass of a hemisphere of radius R.
View SolutionFind the intensity of gravitational field at a point lying at a distance x from the centre on the axis of a ring of radius a and mass M.
View Solution

