Charge over a nonconducting ring is distributed so that the linear charge density varies as λ=λ0sinθ. What is the direction of force on a charge q0 placed at the center?
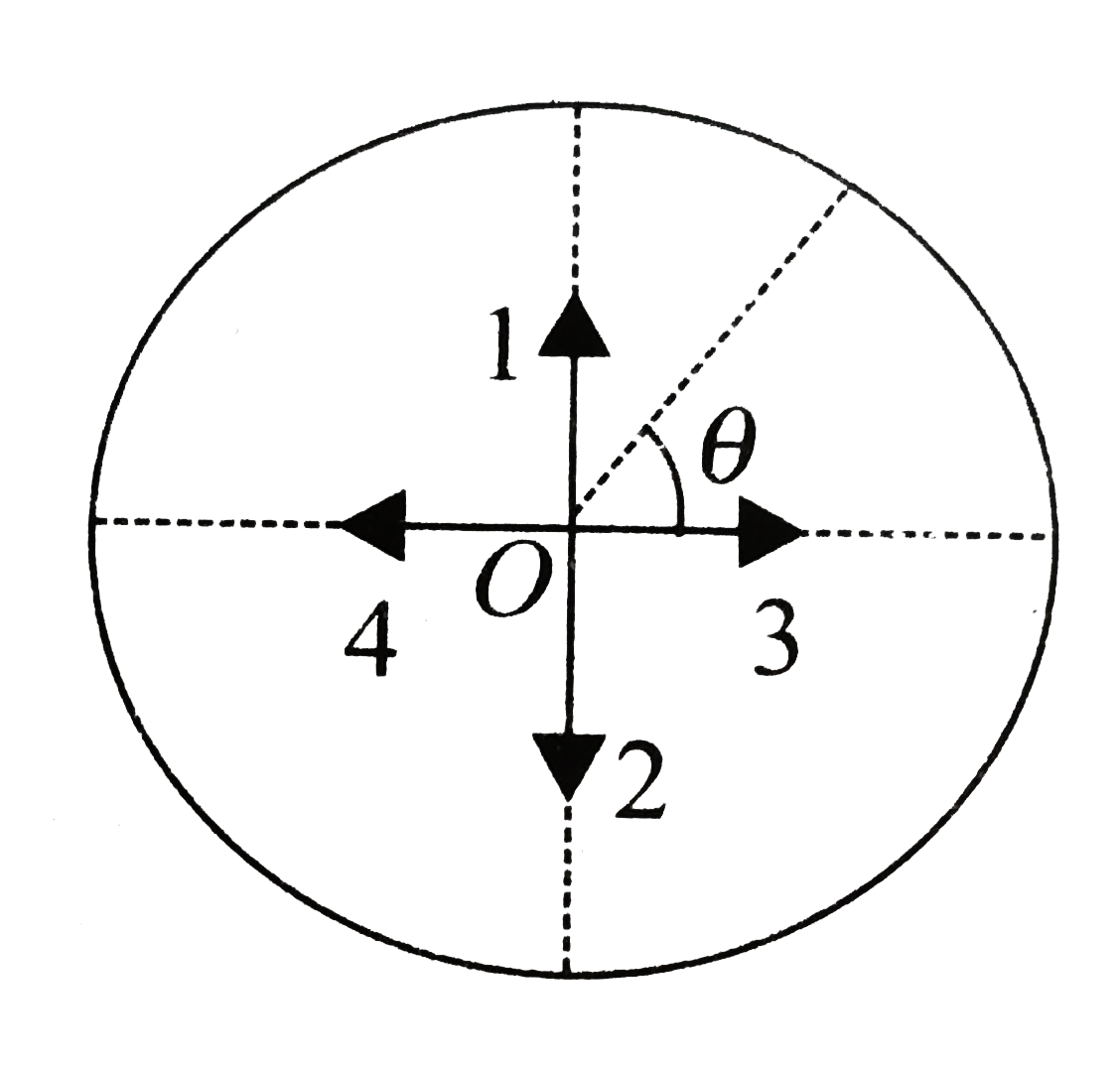
along 1 if q0 is positive and 2 if q0 is negative.
along 2 if q0 is positive and 1 if q0 is negative.
along 3 if q0 is positive and 4 if q0 is negative.
along 4 if q0 is positive and 3 if q0 is negative.
The correct Answer is:B
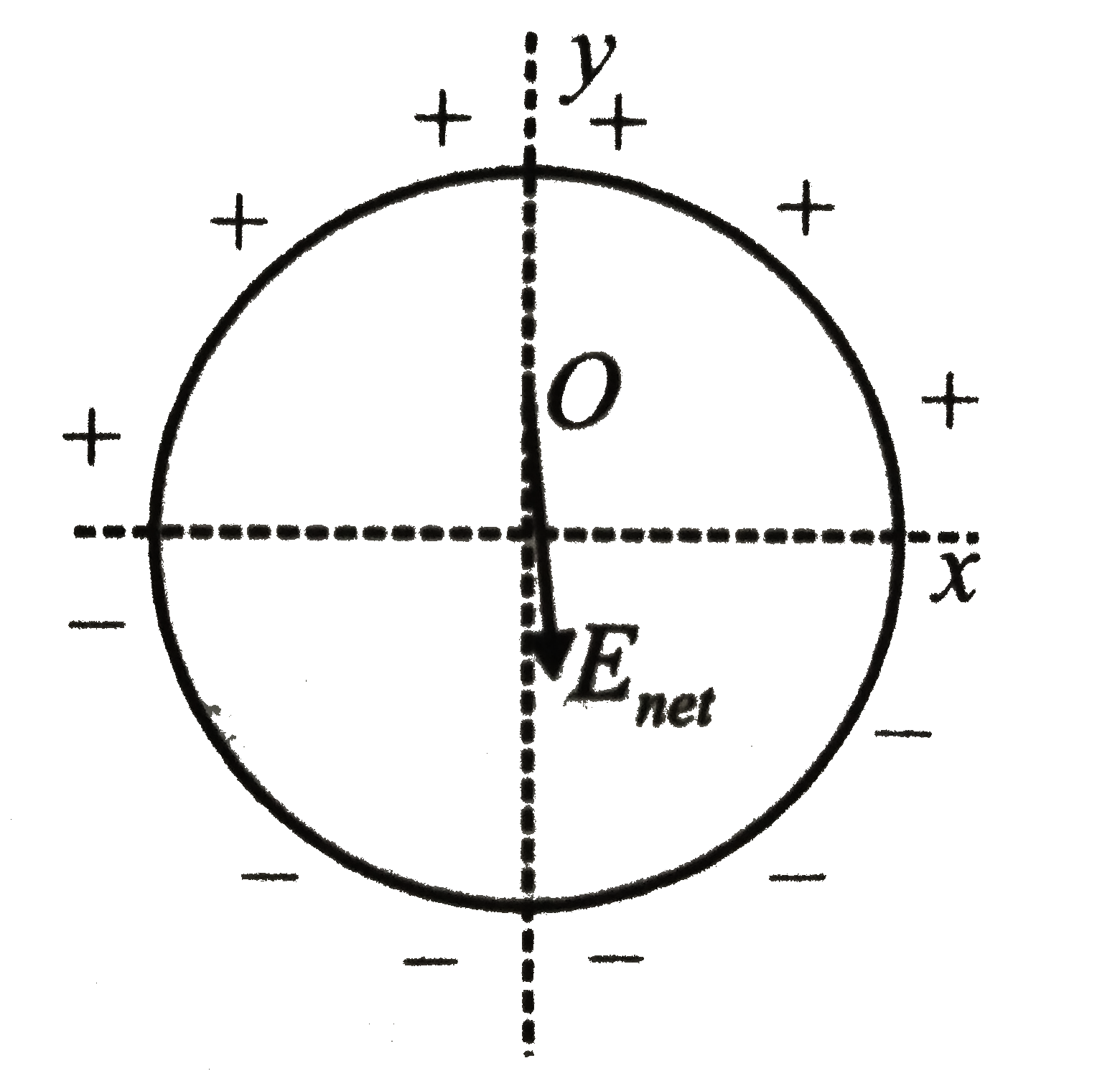
For θ≤θ<2π,λ is negative and symmmetrically distributed about y−axix.
Hence direction of electric Beld Ene at O due to charge distribution is in downward direction, If q0 is
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - Assertion and Reason Type
8 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - Comprehension Type
94 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Multiple Correct Answer type
2 Videos
- MISCELLANEOUS VOLUME 5BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Integer
12 Videos
Similar Questions
The dimensional formula of linear charge density λ is
View SolutionA non conducting ring of radius R1 is charged such that the linear charge density is λ1cos2θ where θ is the polar angle. If the radius is increased to R2 keeping the charge constant, the linear charge density is changed to λ2cos2θ. The relation connecting R1, R2λ1 and λ2 will be
View SolutionIf linear charge density of a wire as shown in the figure is λ

View SolutionTwo mutually perpendicular long straight conductors carrying uniformly distributed charges of linear charges densities λ1 and λ2 are positon at a distance a from each other. How does the interaction between the rods depends on a ?

View SolutionA thin non-conducting ring or radius a has a linear charge density λ=λ0sinϕ. A uniform electric field E0ˆi+E0ˆj exist in the region . .Net torque acting on ring is given as :
View SolutionA half ring of radius r has a linear charge density λ.The potential at the centre of the half ring is
View SolutionA thin nonconducting ring of radius R has a linear charge density λ=λ0cosφ, where λ0 is a constant , ϕ is the azimuthal angle. Find the magnitude of the electric field strength
(a) at the centre of the ring ,
(b) on the axis of the ring as a function of the distance x from its centre. Investegation the obtained function at x>>R.
View SolutionA ring of radius R carries a non - uniform charge of linear density λ=λ0cosθ sec in the figure) Magnitude of the net dipolement of the ring is :

View SolutionIntensity of electric field at a point at a perpendicular distance .r. from an infinite line charge, having linear charge density .λ. is given by :
View SolutionA long charged cylinder of linear charge density λ is surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders ?
View Solution
