Consider a sinusoidal travelling wave shown in figure. The wave velocity is +40cm/s.
Find
(a) the frequency
(b) the phase difference between points 2.5cm apart
( c ) how long it takes for the phase at a given position to (pi//3)
(d) the velocity of a particle at P at the instant shown. 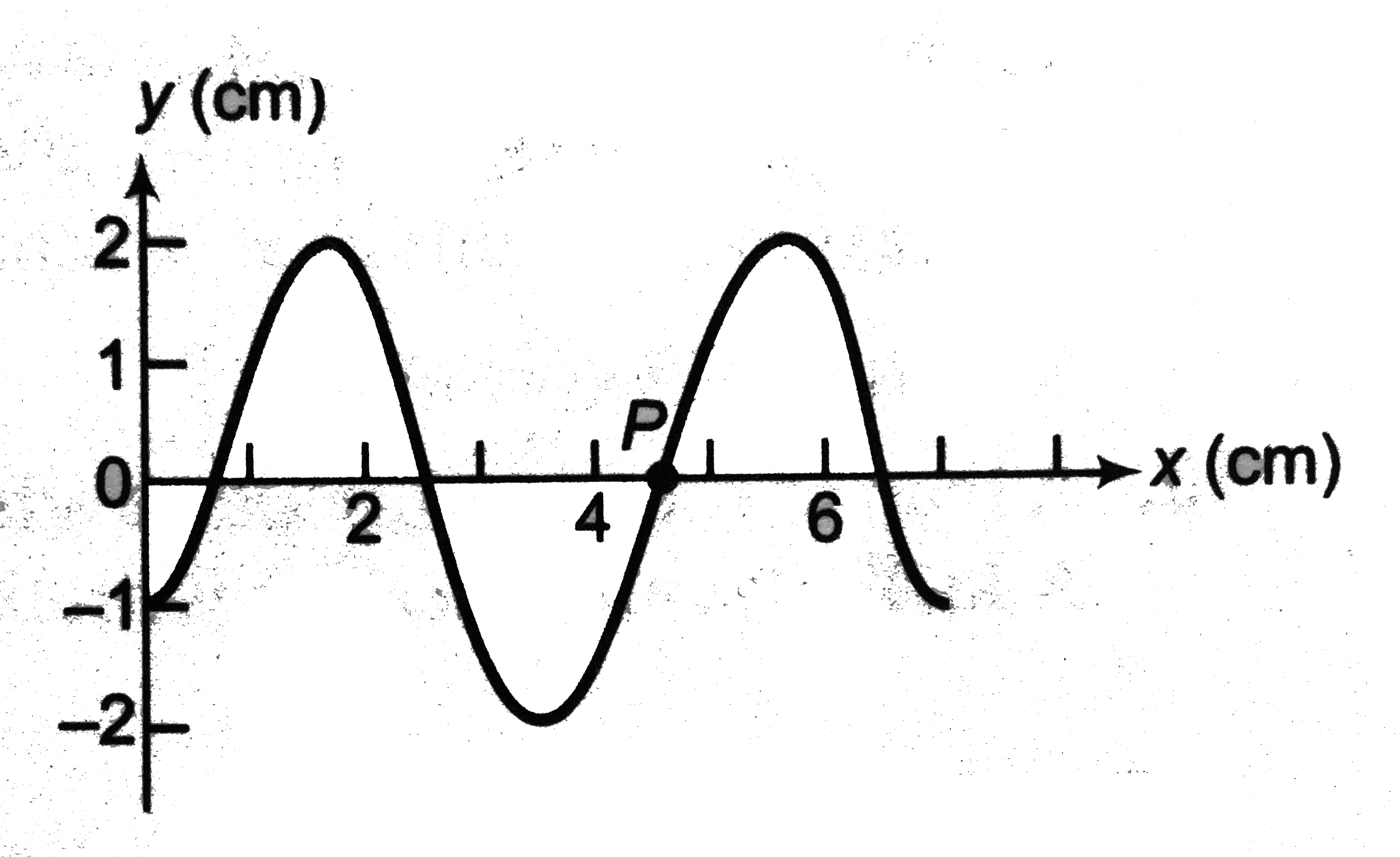
20Hz
30Hz
25Hz
10Hz
λ=2(4.5−2.5)=4cm,v=nλ
⇒n=404=10Hz
ϕ=2πλΔx=5π4rad⇒ϕ=2πTΔt
Δt=ϕ2πn=π/32π10=160s
velocity of p should be maximum, as it is at mean position.
vp=ωA=2πfA=2π×10×2100
=1.26m/s
this velocity should be −ve, because slope at p is +ve.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Integer9videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Assertion-Reasoning6videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct9videos
TRANSMISSION OF HEAT
- BOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
Consider a sinusoidal travelling wave shown in figure. The wave velocity is +40cm/s.
Find
(a) the frequency
(b) the phase difference between points 2.5cm apart
( c ) how long it takes for the phase at a given position to
(d) the velocity of a particle at P at the instant shown. View Solution
View SolutionBlock 5 shown in figure moves to the right with a constant velocity of 30 cm/s. Find :
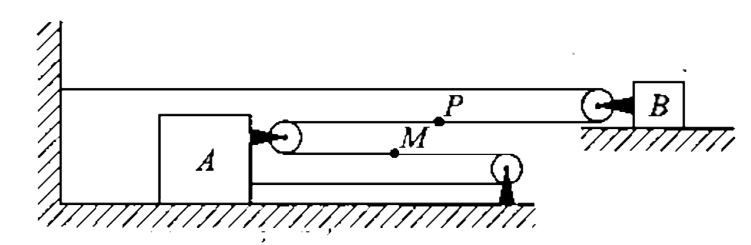
(a) The velocity of block A.
(b) The velocity of the point P of the string.
(c) The velocity of the point M of the string.
(d) The relative velocity of the point P of the string with respect to the block A.View Solution
Knowledge Check
- Question 1 - Select One
Small amplitude progressive wave in a stretched string has a speed of 100cm/s and frequency 100 Hz. The phase difference between two points 2.75 cm apart on the string in radians, is
A0B11π/2Cπ/4D3π/8 - Question 2 - Select One
A transverse wave along a stretched string has a speed of 30 m/s and a frequency of 250 Hz. Then the phase difference between two points on the string 10 cm apart at the same instant is
A0Bπ/2 radC5π/3D8π/3 - Question 3 - Select One
A progressive wave moves with a velocity of 36 m/s in a medium with a freqency of 200Hz. The phase difference between two particles separeted by a distance of 1cm is
A40∘B20radCπ9 radD(π9)∘
A wave of frequency 500Hz has a wave velocity of 350m/s.
(a) Find the distance between two points which are 60∘ out of phase.
(b) Find the phase difference between two displacement at a certain point at time 10−3s apart.View SolutionA transverse progressive wave on a stretched string has a velocity of 10ms−1 and a frequency of 100 Hz . The phase difference between two particles of the string which are 2.5cm apart will be
View SolutionIn a string the speed of wave is 10 m/s and its frequency is 100 Hz. The value of the phase difference at a distance 2.5 cm will be:
View SolutionThe phase difference between two points separated by 0.8 m in a wave of frequency 120 Hz is 0.5π . The wave velocity is
View SolutionThe phase difference between two particles in a medium separated by a distance x is π/6 .If the frequency of the oscillation is 50 Hz and the velocity of propagation of the wave is 100 m/s then x =
View Solution

