Define angle of friction. The inclination θ of a rough plane is increased gradually. The ply on the plane just comes into motion when inclination θ becomes 30∘. Find coefficient of friction. If the inclination is further increased to 45∘ then find acceleration of the body along the plane. (g = 10 m.s−2)
Here, angle of repose 30∘
∴ Coefficient of friction, μ=tan30∘=1√3
If the angle of inclinataion is 45∘, the acceleration of the body,
a=g(sin45∘−μ.cos45∘)=10×1√2(1−μ.)
10√2(1−1√3)≈2.99m.s−2[∵μ.≈μ]
Topper's Solved these Questions
- FRICTION BOOK - CHHAYA PUBLICATIONCHAPTER - FRICTION EXERCISE - EXAMINATION ARCHIVE with solutions ( JEE Main )
2 Videos
- FIRST AND SECOND LAW OF THERMODYNAMICSBOOK - CHHAYA PUBLICATIONCHAPTER - FIRST AND SECOND LAW OF THERMODYNAMICSEXERCISE - CBSE SCANNER
18 Videos
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - CBSE SCANNER
1 Videos
Similar Questions
Knowledge Check
A block of mass 5 kg is placed on a rough inclined plane. The inclination of the plane is gradually increased till the block just begins to slide down. The inclination of the plane is than 3 in 5. The coefficient of friction between the block and the plane is (Take, g=10m/s2)
A35B34C45D23A body is placed on a rough inclined plane of inclination θ. As the angle θ is the increased from 0∘ to 90∘, the contact force between the block and the plane
 Aremains constantBdecreases.Cfirst decreases then increasesDfirst increases then decrease.
Aremains constantBdecreases.Cfirst decreases then increasesDfirst increases then decrease.A block of weight w rests on a horizontal plane. A force P inclined at an angle theta to the plane is applied to the block until it is on the of motion. Find the value of coefficient of friction.
 Apsinθ(psinθ−ω)Bpcosθ(ω−psinθ)Cpcosθ(psinθ−ω)Dpsinθ(ω−psinθ)
Apsinθ(psinθ−ω)Bpcosθ(ω−psinθ)Cpcosθ(psinθ−ω)Dpsinθ(ω−psinθ)
A 20kg box is gently placed on a rough inclined plane of inclination 30∘ with horizontal. The coefficient of sliding friction between the box and the plane is 0.5.Find the acceleration of the box down the incline.
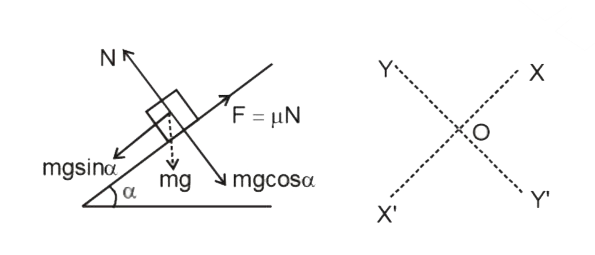
View SolutionA block of mass m is at rest on a rough inclined plane of angle of inclination θ. If coefficient of friction between the block and the inclined plane is μ, then the minimum value of force along the plane required to move the block on the plane is
View SolutionA block of mass m is at rest on a rough inclined plane of angle of inclination θ. If coefficient of friction between the block and the inclined plane is μ, then the minimum value of force along the plane required to move the block on the plane is
View SolutionA block of mass 5 kg is placed on a rough inclined plane. The inclination of the plane is gradually increased till the block just begins to slide down. The inclination of the plane is than 3 in 5. The coefficient of friction between the block and the plane is (Take, g=10m/s2)
View SolutionA body is placed on a rough inclined plane of inclination θ. As the angle θ is the increased from 0∘ to 90∘, the contact force between the block and the plane

View Solution

