δμ+δW=0is valid for
adiabatic process
isothermal process
isobaric process
isochoric process
To solve the question δu+δW=0, we need to analyze the terms involved and their implications in thermodynamics.
1. Understanding the Terms:
- Here, δu represents the change in internal energy of the system.
- δW represents the work done by the system.
2. Applying the First Law of Thermodynamics:
- The first law of thermodynamics states that the change in internal energy of a system is equal to the heat added to the system minus the work done by the system:
\(
\Delta U = Q - W
\)
- Rearranging this gives:
\(
Q = \Delta U + W
\)
3. Interpreting the Given Equation:
- The equation δu+δW=0 can be rearranged to:
\(
\Delta U = -\Delta W
\)
- This implies that the change in internal energy is equal to the negative of the work done by the system.
4. Identifying Conditions:
- For the equation to hold true, the heat transfer Q must be zero. This is because if there is no heat added to or removed from the system, all the energy changes must be accounted for by the work done.
5. Conclusion:
- The condition where Q=0 corresponds to an adiabatic process. In an adiabatic process, there is no heat exchange with the surroundings, and thus any change in internal energy is solely due to work done.
Final Answer:
The equation δu+δW=0 is valid for adiabatic processes.
---
- Here, δu represents the change in internal energy of the system.
- δW represents the work done by the system.
- The first law of thermodynamics states that the change in internal energy of a system is equal to the heat added to the system minus the work done by the system:
\(
\Delta U = Q - W
\)
- Rearranging this gives:
\(
Q = \Delta U + W
\)
- The equation δu+δW=0 can be rearranged to:
\(
\Delta U = -\Delta W
\)
- This implies that the change in internal energy is equal to the negative of the work done by the system.
- For the equation to hold true, the heat transfer Q must be zero. This is because if there is no heat added to or removed from the system, all the energy changes must be accounted for by the work done.
- The condition where Q=0 corresponds to an adiabatic process. In an adiabatic process, there is no heat exchange with the surroundings, and thus any change in internal energy is solely due to work done.
The equation δu+δW=0 is valid for adiabatic processes.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Under which of the following conditions is the relation, ΔH=ΔE+PΔV valid for a system :-
AConstant pressureBConstant temperatureCConstant temperature and pressureDConstant temperature, pressure and composition - Question 2 - Select One
A porcess is nonspontaneous at evey temperature if
(i) ΔH>0,ΔS=0
(ii)ΔH<0,ΔS>0
(iii) ΔH>0,ΔS<0
(iv) ΔH=0,ΔS<0A(i),(ii),(iii)B(ii),(iii),(iv)C(i),(ii),(iii),(iv)D(i),(iii),(iv) - Question 3 - Select One
A student records ΔQ,ΔU and ΔW for a thermodynamic cycle A→B→C→A. Certain entries are missing. Find correct entry in following options.
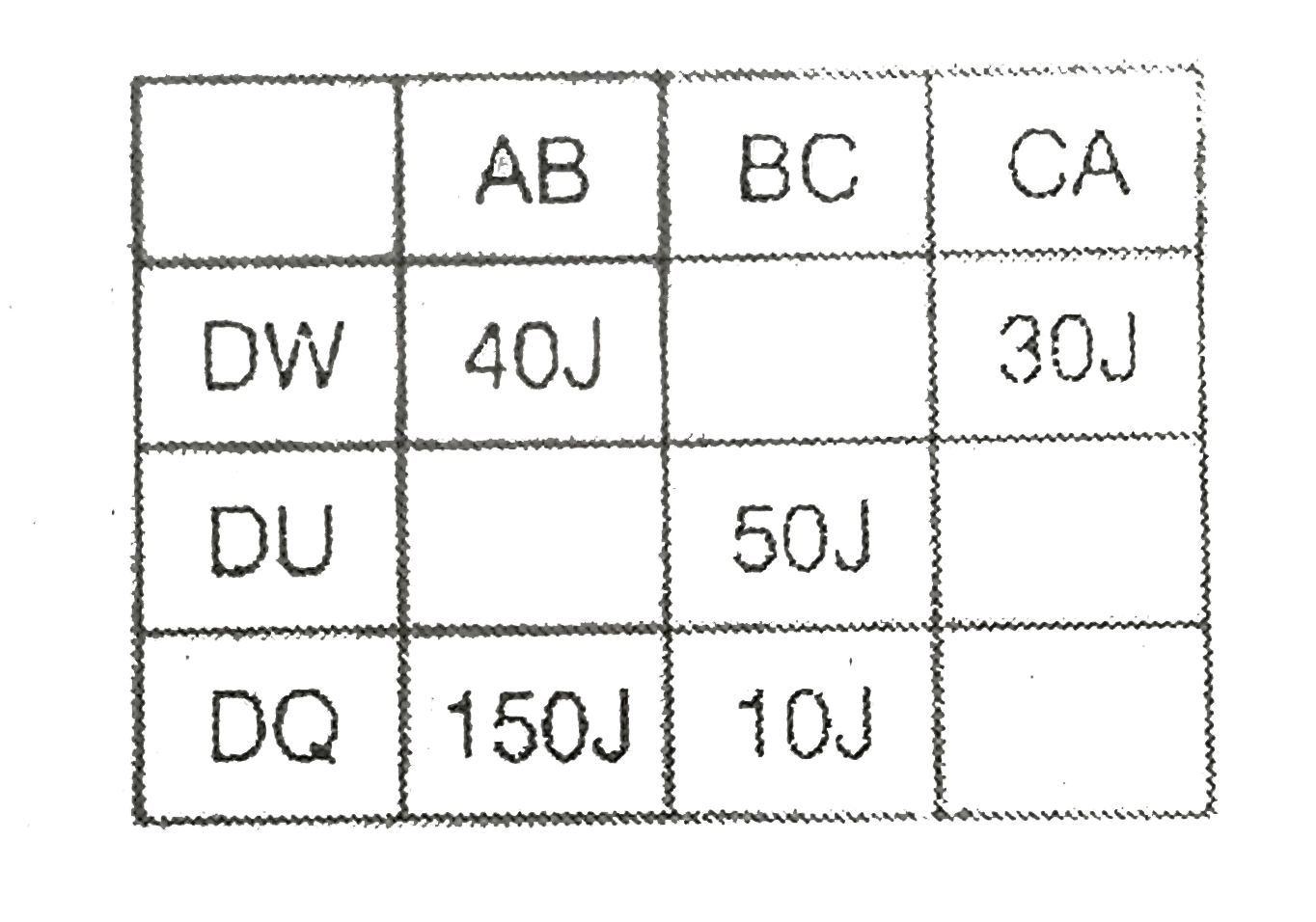 AWBC=−70JBΔQCA=130JCΔUAB=190JDΔUCA=−160J
AWBC=−70JBΔQCA=130JCΔUAB=190JDΔUCA=−160J
Calculate w,q,ΔH and ΔU for an ideal gas expanding in vaccum
adiabaticallyView SolutionFor a cyclic process,the condition is (A) ΔU=0 only (B) ΔH=0 only (C) ΔU>0 and ΔH>0 (D) both ΔU=0 and ΔH=0
View SolutionAn ideal gas undergoes the process 1→2 as shown in the figure, the heat supplied and work done in the process is ΔQandΔW respectively. The ratio ΔQ:ΔW is
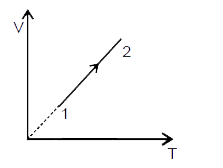 View Solution
View SolutionLet (ΔWa)and(ΔWb) be the work done by the system A and B respectively in the previous question.
View SolutionFour monochromatic light waves are represented as follows:
P:E=E0sinωt
Q:E=2E0sin(ωt+δ)
R:E=E0sin2ωt
S:E=2E0sin(2ωt+δ)
Sustained interference pattern is obtained due to superposition ofView Solution

