Electric field lines are a pictorial representation of the electric field around charges.
A. State Gauss's Law in Electrostatics.
B. Using this law derive an expression for the electric field intensity due to a uniformly charged thin spherical shell at a point.
i. Outside the shell ii. Inside the shell
C. Suppose that you are in a cave deep withing the earth. Are you safe from thunder and lighting? Why? Solution in Tamil
Step by step video & image solution for Electric field lines are a pictorial representation of the electric field around charges. A. State Gauss's Law in Electrostatics. B. Using this law derive an expression for the electric field intensity due to a uniformly charged thin spherical shell at a point. i. Outside the shell ii. Inside the shell C. Suppose that you are in a cave deep withing the earth. Are you safe from thunder and lighting? Why? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Competitive Exam Corner
20 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Evaluation Questions And Answers
12 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - NEW JOYTHI PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - COMPETITIVE EXAM CORNER
15 Videos
- ELECTROMAGNETIC INDUCTION BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - COMPETITIVE EXAM CORNER
13 Videos
Similar Questions
Two charged spherical conductors of radii R1andR2 are connected by a wire. Then the ratio of surface charge densitites of the spheres σ1/σ2 is
View SolutionA glass rod rubbed with silk is brought close to two uncharged spheres in contact with each other inducing charges on them as shown in figure.
a. What happens when the spheres are slightly separated.
b. the glass rod is subsequently removed.
c. the spheres are separated far apart.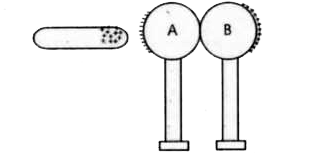
View SolutionIf 'm' is the mass of an electron and 'c' is the speed of light, the ratio of the wavelength of a photon of energy E to that of the electron of the same energy is
View SolutionA 50 Hz ac current of peak value 2 A flows through one of the pair of coils. If the mutual inductance between the pair of coils is 150 mH, then the peak value of voltage induced in the second coil is
View SolutionApplying Gauss's law derive the expression for electric intensity due to a charged conducting spherical shell at (i) a point outside the shell (ii) a point on the surface of the shell and (iii) a point inside the shell.
View SolutionUsing Gauss's law in electrostatics, obtain an expression for electric field due to a uniformly charged thin spherical shell at a point (i) Outside the shell and (ii) Inside the shell
View SolutionUsing Gauss's law in electrostatics, obtain an expression for electric field due to a uniformly charged thin spherical shell at a point
(i) Outside the shell and
(ii) Inside the shell
View SolutionFind expression for electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionState Gauss's theorem. Obtain an expression for elactric field at any point outside a charged spherical hollow conductor (shell).
View SolutionState Gauss's theorem. Obtain an expression for elactric field at any point outside a charged spherical hollow conductor (shell).
View SolutionState Gauss's theorem in electrostatics. Apply this theorem to derive an expression for electric field intensity at a point outside a uniformly charged thin spherical shell.
View SolutionState Gauss's theorem with the help of diagram, derive an expression for the electric field intensity due to uniformly charged thin spherical shell at a point outside
View SolutionState Gauss's theorem with the help of diagram, derive an expression for the electric field intensity due to uniformly charged thin spherical shell at a point inside
View SolutionState Gauss's theorem with the help of diagram, derive an expression for the electric field intensity due to uniformly charged thin spherical shell at a point on the surface of the spherical shell.
View SolutionDerive an expression for electric field due to a uniformly charged spherical shell. i) at a point outside the shell
View Solution
