Force of attraction between the plates of a parallel plate capacitor is
q2ε0AK
q22ε0AK
q22ε0A
q22ε0A2K
The correct Answer is:B
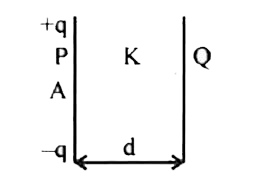
Consider a parallel plate capacitor, of area A, plate separation d and filled with a medium of dielectric constant K.
The electric field near a non-conducting plane sheet (P) having uniform surface charge density σ is given by
E=σ2ε0K
∴ The force on the plate Q due to the charged plate P is
F=qE=q⋅(σ2ε0K)=q2ε0K(qA) ∴σ=qA
∴F=q22ε0AK
Second Method:
The energy of a parallel plate capacitor
U=12q2C=q2d2⋅KAε0 ∵C=KAε0d
∴If the plate separation is increased by Δx, then increases in energy ΔU=12q2ΔxKAε0 . . . (1)
[Except Δx all other quantities remain constant]
If F is the force between the plates, thenn the work done in increasing the plate separation by Δx is
dW=Fdx . . (2)
but ΔU=dW ∴From (1) and (2)
∴Fdx=12q2ΔxKAε0 ∴F=12q2KAε0
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MARVEL PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - TEST YOUR GRASP
20 Videos
- ELECTRONS AND PHOTONS BOOK - MARVEL PUBLICATIONCHAPTER - ELECTRONS AND PHOTONS EXERCISE - TEST YOUR GRASP - 17
15 Videos
- GRAVITATION BOOK - MARVEL PUBLICATIONCHAPTER - GRAVITATION EXERCISE - TEST YOUR GRASP -2
20 Videos
Similar Questions
separation between the plates of a parallel-plate capacitor is 2 mm and the area of its plates is 5cm2. If the capacitor is charged such that it has 0.01J energy stored in it, the electrostatic force of attraction between its plates is _______N.
View SolutionThe distance between the plates of a parallel plate capacitor is 'd'. The capacitance of the capacitor gets doubled when a metal plate of thicknessd2 is placed between the plates.
View Solution
Knowledge Check
A parallel plate air capacitor has capacity C distance of separation between plates is d and potential difference V is applied between the plates force of attraction between the plates of the parallel plate air capacitor is
AC2V22d2BC2V22dCCV22dDCV2dThe separation between the plates of a charged parallel-plate capacitor is increased. The force between the plates
AincreasesBdecreasesCremains sameDfirst increases then decreasesThe expression for the capacity of a capacitor formed by placing a compound dieletric between the plates of a parallel plate capacitor, as shown in figure , will be (Given area of plate = A)
 Aε0A(d1K1+d2K2+d3K3)Bε0A(d1K1+d2K2+d3K3)Cε0A(K1K2K3)(d1d2d3)Dε0(AK1d1+AK2d2+AK3d3)
Aε0A(d1K1+d2K2+d3K3)Bε0A(d1K1+d2K2+d3K3)Cε0A(K1K2K3)(d1d2d3)Dε0(AK1d1+AK2d2+AK3d3)
The' distance between the plates of a parallel plate capacitor is d. A metal plate of thickness d/2 is placed between the plates. What will e its effect on the capacitance.
View SolutionThe distance between the plates fo a parallel plate capacitor is d. A metal plate of thickness d/2 is placed between the plates, what will be the new capacity ?
View SolutionThe expression for the capacity of the capacitor formed by compound dielectric placed between the plates of a parallel plate capacitor as shown in figure will be
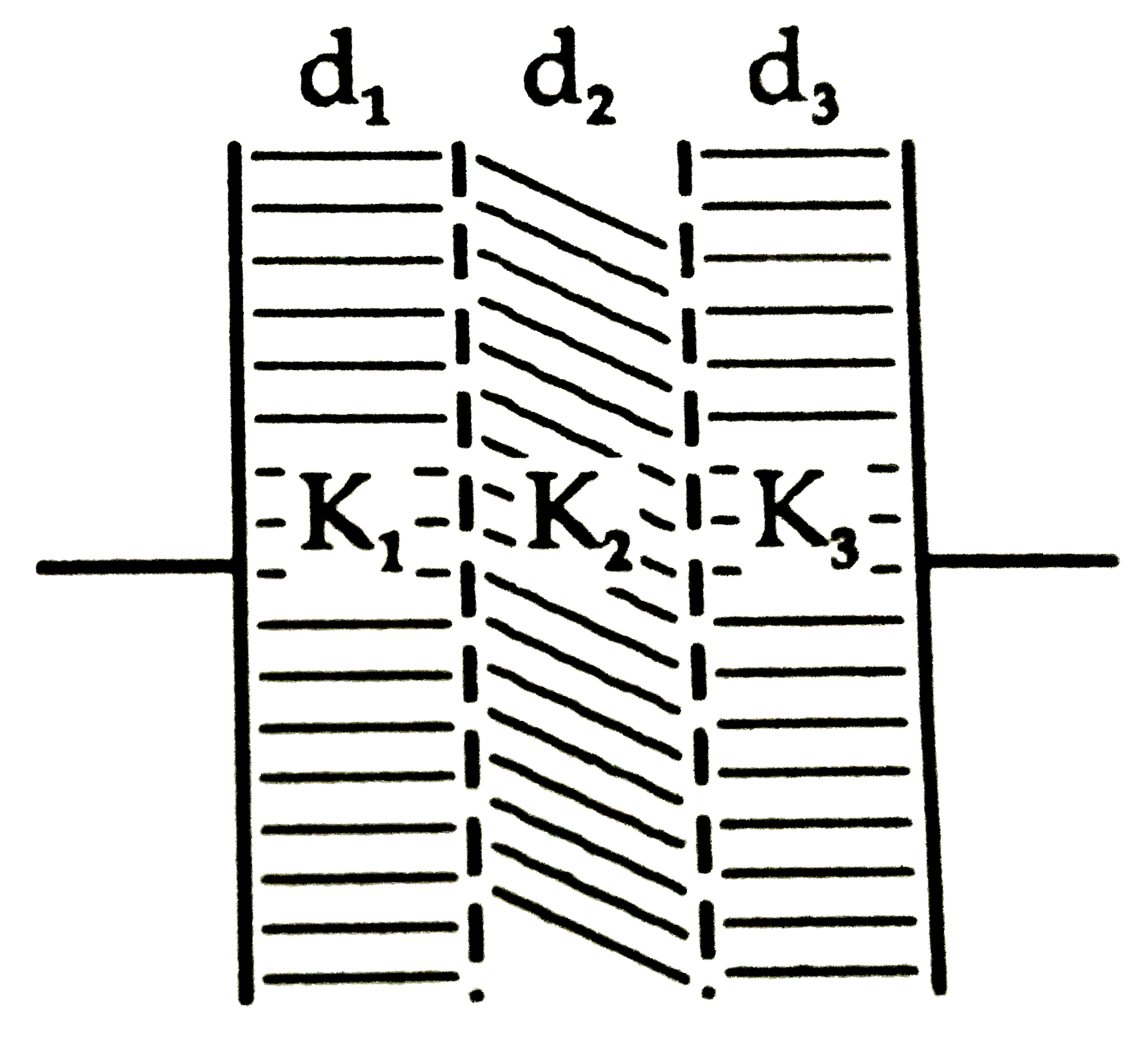
View SolutionThe expression for the capacity of the capacitor formed by compound dielectric placed between the plates of a parallel plate capacitor as shown in figure, will be (area of plate =A)

View SolutionA dielectric slab is placed between the plates of a parallel plate capacitor. Its capacitance
View Solution
