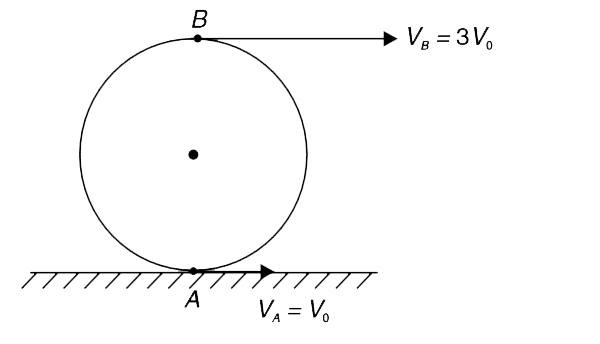
(i) A solid sphere of radius R is released on a rough horizontal surface with its top point having thrice the velocity of its bottom point A (VA=V0) as shown in figure. Calculate the linear velocity of the centre of the sphere when it starts pure rolling.
(ii) Solid sphere of radius R is placed on a rough horizontal surface with its centre having velocity V0 towards right and its angular velocity being ω0 (in anticlockwise sense). Find the required relationship between V0 and ω0 so that -
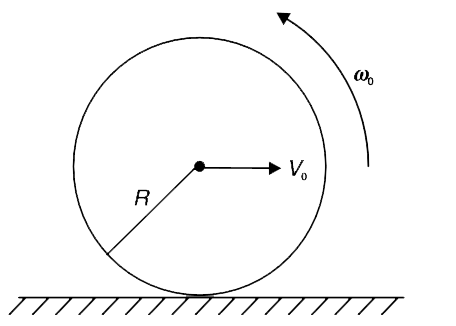
(a) the slipping ceases before the sphere loses all its linear momentum.
(b) the sphere comes to a permanent rest after some time.
(c) the velocity of centre becomes zero before the spinning ceases.
The correct Answer is:(i) 12V07
(ii) (a) V0>25ω0R
(b) V0=25ω0R
(c) V0<25ω0R
Step by step video, text & image solution for (i) A solid sphere of radius R is released on a rough horizontal surface with its top point having thrice the velocity of its bottom point A (V_(A) = V_(0)) as shown in figure. Calculate the linear velocity of the centre of the sphere when it starts pure rolling. (ii) Solid sphere of radius R is placed on a rough horizontal surface with its centre having velocity V_(0) towards right and its angular velocity being omega_(0) (in anticlockwise sense). Find the required relationship between V_(0) and omega_(0) so that - (a) the slipping ceases before the sphere loses all its linear momentum. (b) the sphere comes to a permanent rest after some time. (c) the velocity of centre becomes zero before the spinning ceases. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
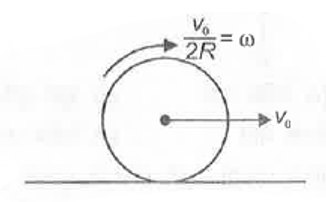
A solid sphere has combined linear and rotation motion as shown in figure. The velocity of its centre of mass when it starts pure rolling is (R = radius of sphere)
 View Solution
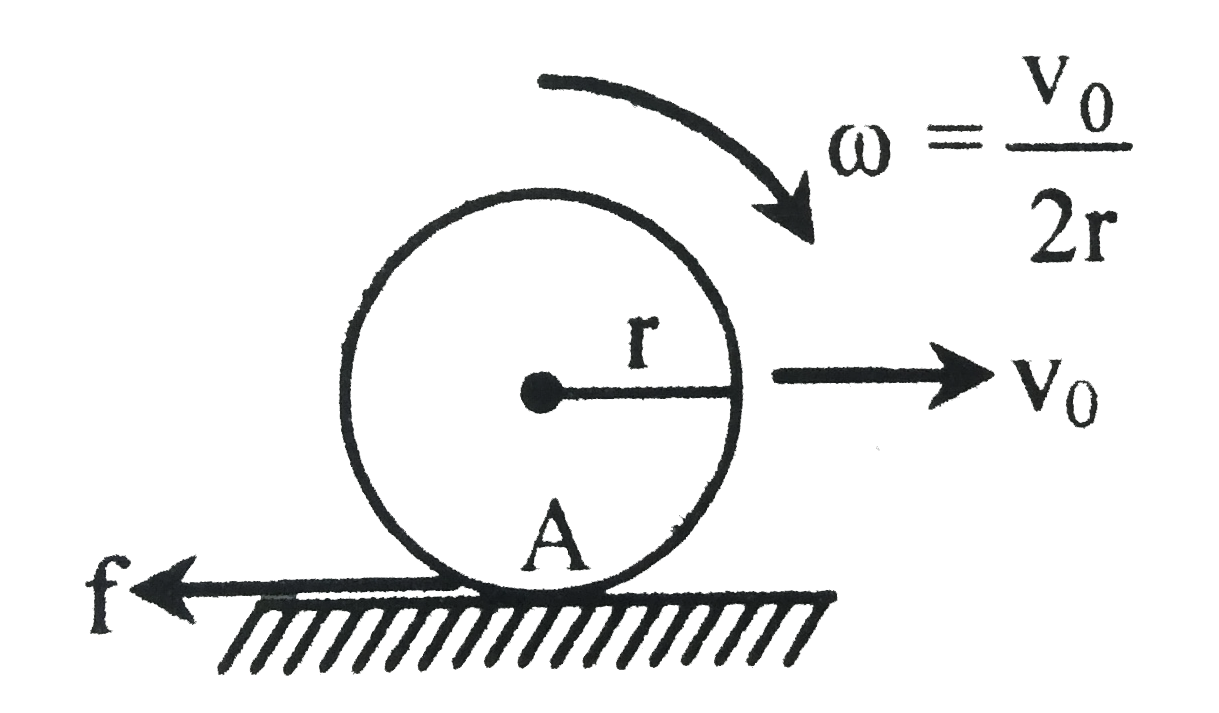
View SolutionA sphere of mass M and radius r shown in figure slips on a rough horizontal plane. At some instant it has translational velocity V0 and rotational velocity about the centre v02r. Find the translational velocity after the sphere starts pure rolling.
 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One
A sphere of radius R is rolling on a rough horizontal surface. The magnitude of velocity of A with respect to ground will be
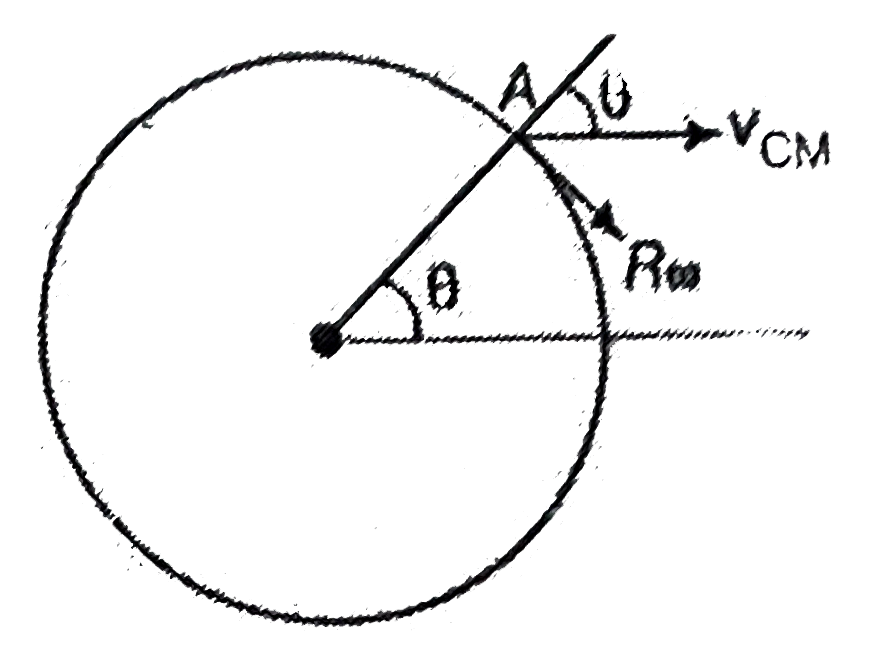 A√2vCMB2VCMsinθC√2vCM√1+sinθDNo sufficient information
A√2vCMB2VCMsinθC√2vCM√1+sinθDNo sufficient information - Question 2 - Select One
A solid sphere of radius r r is rolling on a horizontal surface. The ratio between the rotational kinetic energy and total energy.
A57B27C12D17 - Question 3 - Select One
A solid sphere is set into motion on a rough horizontal surface with a linear velocity v in the forward direction and an angular velocity v/R in the anticlockwise direction as shown in the figure. Find the linear speed of the sphere, when it stops rotating.
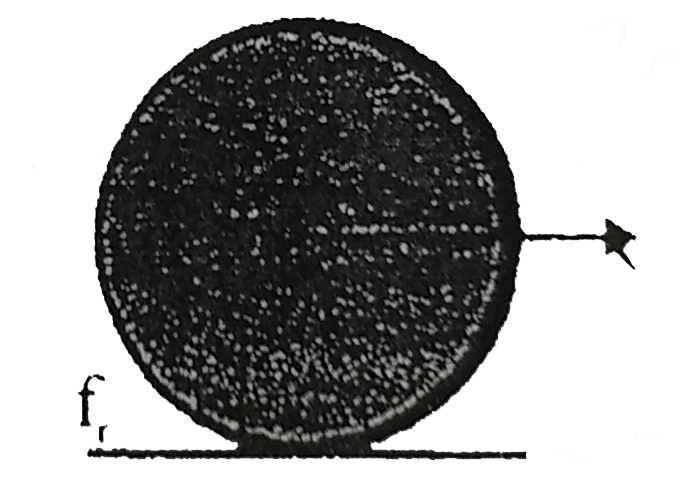 Av5Bv7C3v5D3v7
Av5Bv7C3v5D3v7
A sphere of mass M and radius r shown in figure slips on a rough horizontal plane. At some instant it has translational velocity V0 and rotational velocity about the centre v02r. Find the translational velocity after the sphere starts pure rolling.
 .View Solution
.View SolutionA uniform sphere of mass m and radius R is placed on a rough horizontal surface. The sphere is struck horizontally at a heighth from the floor. Match the following:
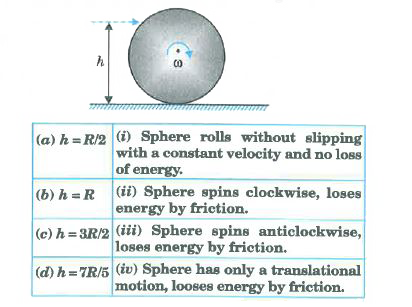 View Solution
View SolutionA sharp vertical impulse J is given to a uniform solid sphere of mass m and radius R place on a rough horizontal surface. Choose the correct options
 View Solution
View SolutionA uniform circular disc of radius r is placed on a rough horizontal surface and given a linear velocity v0 and angular velocity ω0 as shown. The disc comes to rest after moving some distance to the right. It follows that
 View Solution
View SolutionA solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
View Solution

