(i) Draw the plot of amplitude versus $ω$ for an amplitude modulated were whose carrier wave $(ω_{c})$ is carrying two modulating signals, $ω_{1}$ and $ω_{2}$ $(ω_{2} > ω_{1})$ .
(ii) Is the plot symmetrical about $ω_{c}$ ? Comment especially about plot in region $ω < ω_{c}$ .
(iii) Extrapolate and predict the problems one can expect if more waves are to be modulated.
(iv) Suggest solutions to the above problem. In the process can one understand another advantage of modulation in terms of bandwidth?

Video Solution

Text Solution

Verified by Experts

(i) Let the two modulating signals $A_{m_{1}} {sin ω}_{m_{1}} t$ and $A_{m_{2}} {sin ω}_{m_{2}} t$ be superimposed on carrier signal
$A_{c} s i m ω_{c} t$ . The signal produced is $x (t) = A_{m_{1}} {sin ω}_{m_{1}} t + A_{m_{2}} {sin ω}_{m_{2}} t + A_{c} {sin ω}_{c} t$
To produce amplitude modulated wave the signal $x (t)$ is passed through a square law device which produces
an output given by
$y (t) = B [A_{m_{1}} {sin ω}_{m_{1}} + A_{m_{2}} {sin ω}_{m_{2}} t + A_{c} {sin ω}_{c} t] + C {[A_{m_{1}} {sin ω}_{m_{1}} t + A_{m_{2}} {sin ω}_{m_{2}} t + A_{c} s i m ω_{c} t]}_{m_{1}}^{2}$
$B = [A_{m_{1}} {sin ω}_{m_{1}} t + A_{m_{2}} {sin ω}_{m_{2}} t + A_{c} {sin ω}_{c} t] + C [{(A_{m_{1}} {sin ω}_{m_{1}} t + A_{m_{2}} {sin ω}_{m_{2}} t)}_{m_{1}}^{2} + A_{c}^{2} {sin}^{2} ω_{c} t$
$+ 2 A_{c} {sin ω}_{c} t (A_{m_{1}} {sin ω}_{m_{1}} t + A_{m_{2}} {sin ω}_{m_{2}} t)]$
$= B [A_{m_{1}} {sin ω}_{m_{1}} t + A_{m_{2}} {sin ω}_{m_{2}} t + A_{c} {sin ω}_{c} t]$
$+ C [A_{m_{1}}^{2} {sin}^{2} ω_{m_{1}} t + A_{m_{2}}^{2} {sin}^{2} ω_{m_{2}} t + 2 A_{m_{1}} A_{m_{2}} {sin ω}_{m_{1}} t {sin ω}_{m_{2}} t$
$+ A_{c}^{2} s i m^{2} ω_{c} t + 2 A_{c} (A_{m_{1}} {sin ω}_{m_{1}} t {sin ω}_{c} t + A_{m_{2}} {sin ω}_{m_{2}} t {sin ω}_{c} t)]$
$= B [A_{m_{1}} {sin ω}_{m_{1}} t + A_{m_{2}} {sin ω}_{m_{2}} t + A_{c} {sin ω}_{c} t]$
$+ C [A_{m_{1}}^{2} {sin}^{2} ω_{m_{1}} t + A_{m_{2}}^{2} {sin}^{2} ω_{m_{2}} t + A_{m_{1}} A_{m_{2}} {cos (ω_{m_{2}} - ω_{m_{1}}) t - cos (ω_{m_{2}} + ω_{m_{1}})} + A_{c}^{2} {sin}^{2} ω_{c} t$
$+ A_{c} A_{m_{1}} {cos (ω_{c} - ω_{m_{1}}) t - cos (ω_{c} + ω_{m_{1}}) t} + A_{c} A_{m_{2}} {cos (ω_{c} - ω_{m_{2}}) t - cos (ω_{c} + ω_{m_{2}}) t}]$
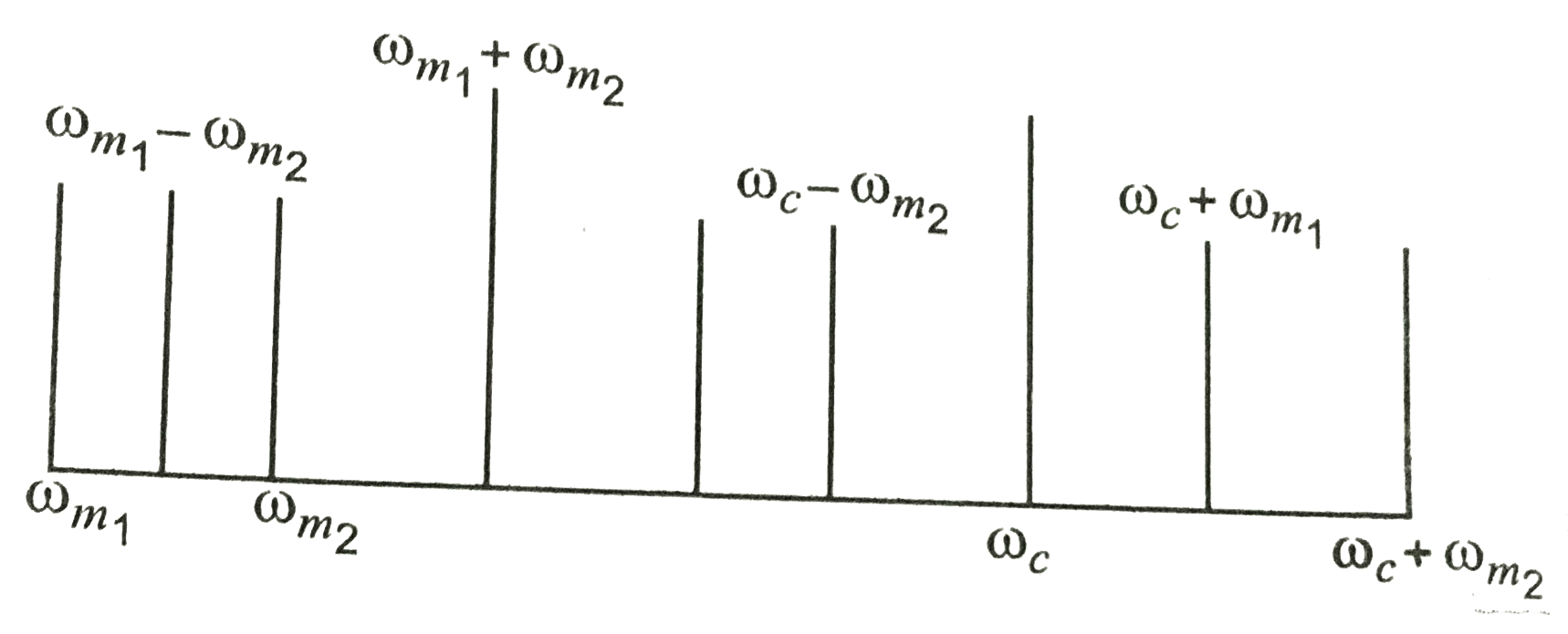
In the above amplitude modulated waves, the frequencies present are
$ω_{m_{1}}, ω_{m_{2}}, ω_{c}, (ω_{m_{2}} - ω_{m_{1}}), (ω_{m_{2}} + ω_{m_{1}}), (ω_{c} - ω_{m_{1}}), (ω_{c} + ω_{m_{1}}), (ω_{c} - ω_{m_{2}})$ and $(ω_{c} + ω_{m_{2}})$
The plot of amplitude versus $ω$ is shown in
figure
(ii) From figure , we note that frequency
spectrum is not symmetrical about $ω_{c}$ .
Crowding of spectrum is present for $ω < ω_{c}$ .
(iii) if more waves are to be modulated then
there will be more crowding in the modulating
signal in the region $ω < ω_{c}$ . That will result
more chances of mixing of signals.
(iv) To accommodate more signals, we should increase band width and frequency of carrier waves $ω_{c}$ . This
shows that large carrier frequency enables to carry more information (i.e., more $ω_{m}$ ) and the same will
inturn increase band width.

Updated on:21/07/2023