If a point moves along a circle with constant speed, prove that its angular speed about any point on the circle is half of that about the centre.
2ωc=ω0
ωc=ω0
ωc=2ω0
ωc=(12)ω0
The correct Answer is:C
Let, O be a point on a circle and P be the position of the particle at any time T , such that
∠POA=θ . Then, ∠PCA=20
Here, C is the centre of the circle.
Angular velocity of P about O is ω0=dθdt
and angular velocity of P about C is,
ωc=ddt(2θ)=2dθdt
or ωc=2ω0
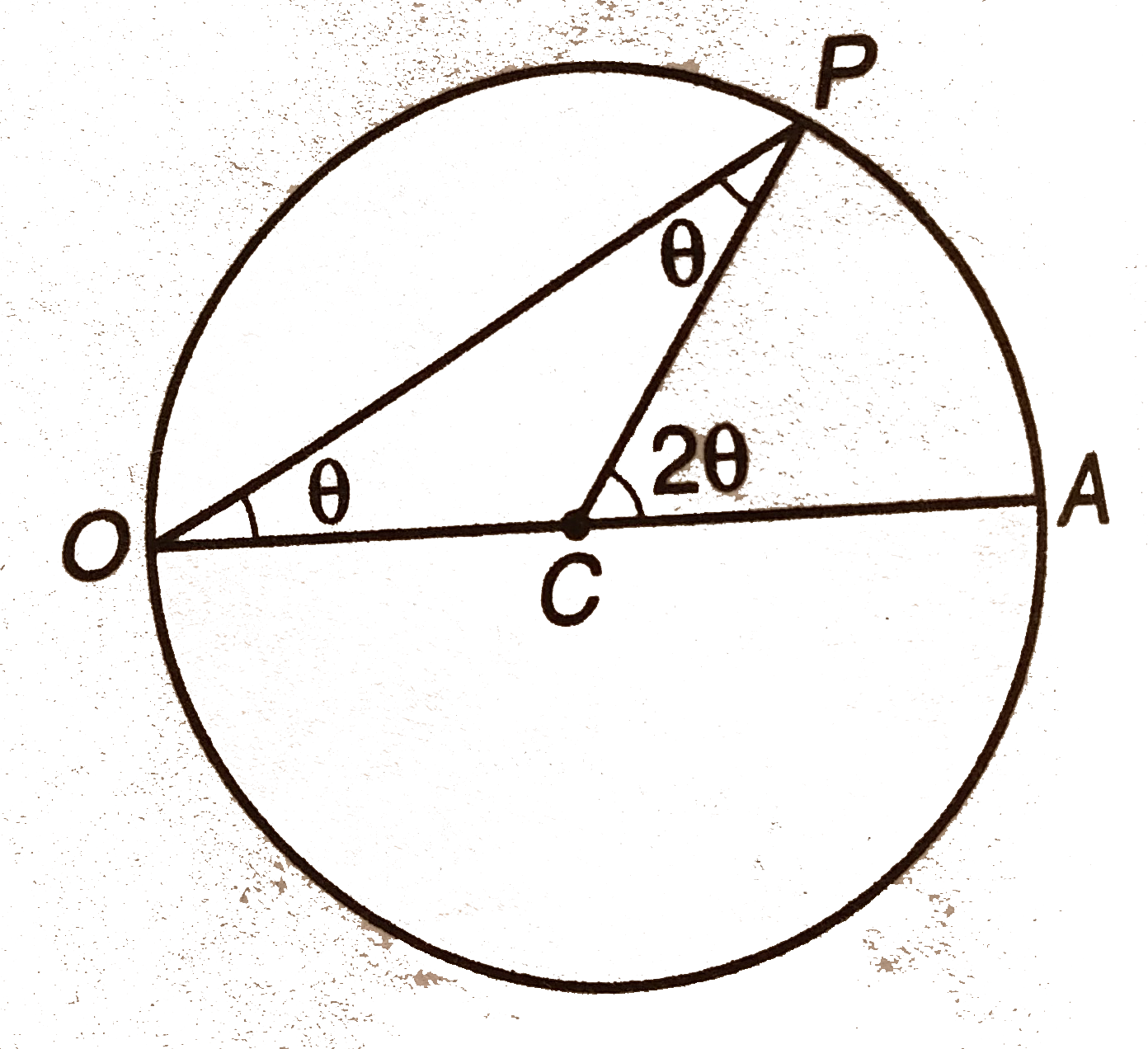
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Exercise 10.1
7 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Exercise 10.2
7 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Solved Examples
2 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
A horse runs along a circle with a speed of 20km/h . A lantern is at the centre of the circle. A fence is along the tangent to the circle at the point at which the horse starts. Find the speed with which the shadow of the horse moves along the fence at the moment when it covers 1/8 of the circle in km/h.
View SolutionA particle moves in a circle with constant angular velocity ω about a point P on its circumference. The angular velocity of the particle about the centre C of the circle is
View Solution
Knowledge Check
If the body is moving in a circle of radius r with a constant speed v , its angular velocity is
Av2/rBvrCv/rDr/vAssertion : A body is moving along a circle with a constant speed. Its angular momentum about the centre of the circle remains constant
Reason : In this situation, a constant non-zero torque acts on the body.AIf both Assertion and Reason are correct and Reason is the correct explanation of AssertionBIf both Assertion and Reason are true but Reason is not the correct explanation of AssertionCIf Assertion is true but Reason is fasleDIf Assertion is false but Reason is trueA particle is moving in a circle with a constant speed. Its moving is
APeriodicBOscillatoryCSimple harmonicDboth 'a' and 'b'
If a particle covers half the circle of radius R with constant speed then
View SolutionIf a body moves with a constant speed in a circle
View SolutionA particle is moving along a straight line with increasing speed. Its angular momentum about a fixed point on this line :
View SolutionA body is moving along circle at constant speed Which of the following statement is true?
View SolutionIf a particle is moving along a circle of radius 3 m with a constant speed 9m/s, then it covers a quarter of the circle in time of
View Solution
