If a point source is placed at a distance of 18 cm from the pole of a concave mirror, its image is formed at a distance of 9 cm from the mirror. A glass slab of thickness 6 cm is placed between the point source and the mirror such that the parallel faces of the glass slab remains perpendicular to the principal axis of the mirror. If the refractive index of glass is 1.5 what will be the displacement of the image?
Let P be the position of the object and in the absence of the glass slab, Q be the position of image formed by the concave mirror [Fig. 2.23].
Here,
If the slab of thickness 6 cm is placed between the point source and the concave mirror, apparent displacement of the point source will take place towards the mirror. The rays coming from P appear to come from P. after refraction. Apparent displacement of P.
So in the second case, object distance u = -(18 - 2) = - 16cm, f = - 6 cm , v = ?
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - REFRACTION OF LIGHTEXERCISE - HIGHER ORDER THINKING SKILL (HOTS QUESTION)
25 Videos
- REFRACTION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - REFRACTION OF LIGHTEXERCISE - SECTION RELATED QUESTION
18 Videos
- REFLECTION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - REFLECTION OF LIGHTEXERCISE - INTEGER ANSWER TYPE
3 Videos
- SEMICONDUCTORS AND ELECTRONICS BOOK - CHHAYA PUBLICATIONCHAPTER - SEMICONDUCTORS AND ELECTRONICS EXERCISE - CBSE SCANNER
31 Videos
Similar Questions
An object is placed at a distance of 30 cm from a concave mirror and its real image is formed at a distance of 30 cm from the mirror. The focal length of the mirror is
View SolutionA point object is placed at a distance of 10 cm and its real image is formed at a distance of 20 cm from a concave mirror. If the object is moved by 0.1 cm towards the mirror, the image will shift by about
View Solution
Knowledge Check
An object is placed at a distance of 30 cm from a concave mirror and its real image is formed at a distance of 30 cm from the mirror. The focal length of the mirror is
A15 cmB45 cmC30 cmD20 cmAn object is placed at a distance of 20 cm from the pole of a concave mirror of focal length 10 cm. The distance of the image formed is
A+20cmB+10CmC−20cmD−10cmAn object is placed at a distance of 15 cm from a concave mirror and its real image is formed at 30 cm in front of that mirror. The focal length of the mirror is
A+20 cmB−20 cmC−10 cmD+10 cm
A point object is placed on principal axis of a concave mirror of radius of curvature 20cm at a distance 31cm from poll of the mirror. A glass slab of thikness 3cm and refractive index 1.5 is placed between object and mirror as shown in the figure. Find the distance (in cm) of final image formed by the system from the mirror.
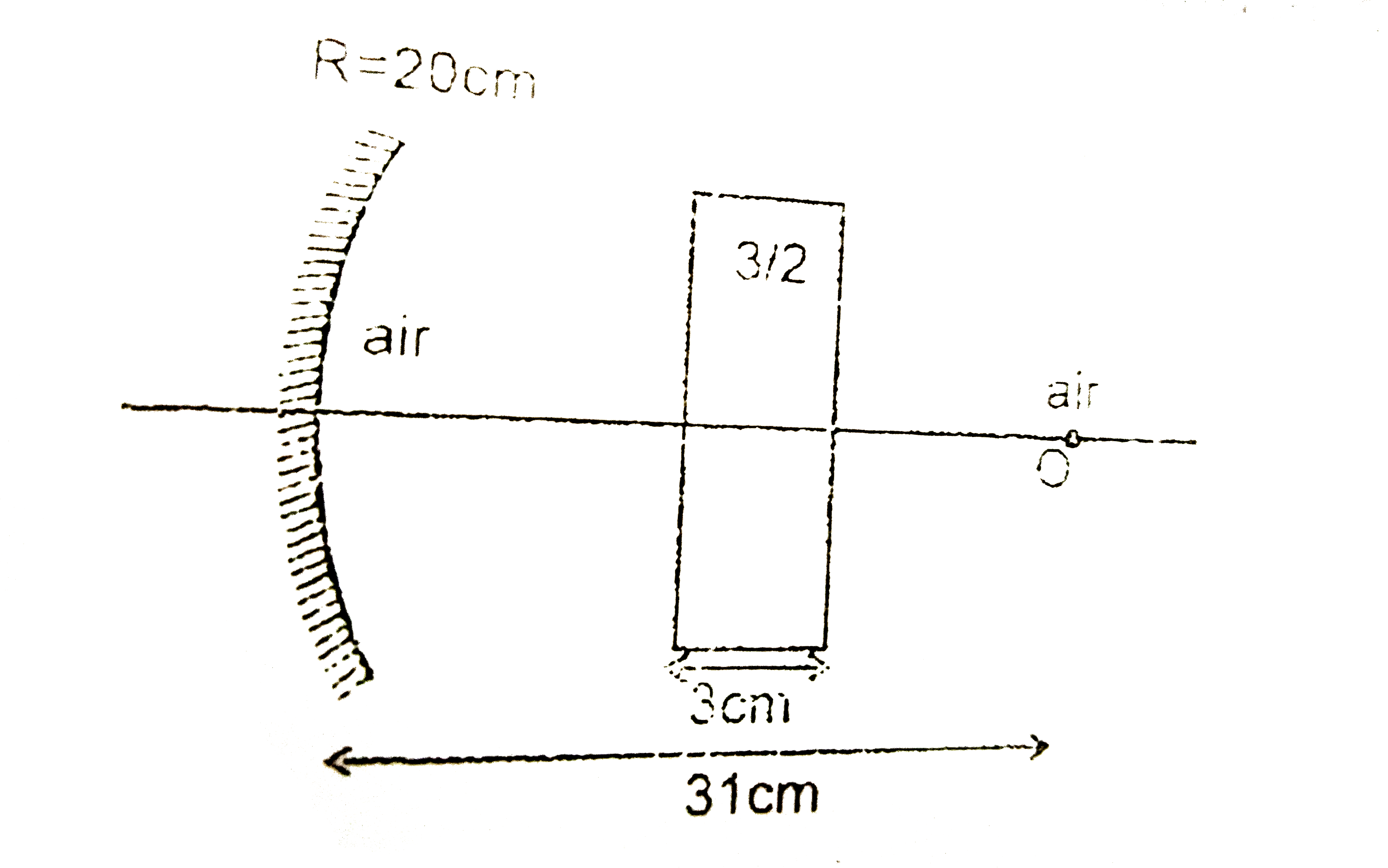
View SolutionA point object is placed on principal axis of a concave mirror of radius of curvature 20cm at a distance 31cm from poll of the mirror. A glass slab of thikness 3cm and refractive index 1.5 is placed between object and mirror as shown in the figure. Find the distance (in cm) of final image formed by the system from the mirror.

View SolutionAn object is placed at 21 cm in front of a concave mirror of radius of a curvature 10 cm. A glass slab of thickness 3 cm and u 15 is then placed close to the mirror in the space between the object and the mirror. The position of final image formed is
View SolutionAn object is placed on the principle axis of a concave mirror of focal length 10cm at a distance of 21cm from it. A glass slab is placed between the mirror and the object as shown in figure.
Find the distance of final image formed by the mirror.

View SolutionA point object is placed at a distance of 10 cm and its real image is formed at a distance of 20 cm from a concave mirror. If the object is moved by 0.1 cm towards the mirror, the image will shift by about
View Solution
