If an object is placed at A(OA>f), where f is the focal length of the lens, the image is formed at B. A perpendicular is erected at O and C is chosen on it such that the angle (∠BCA) is a right angle. Then, the value of f will be 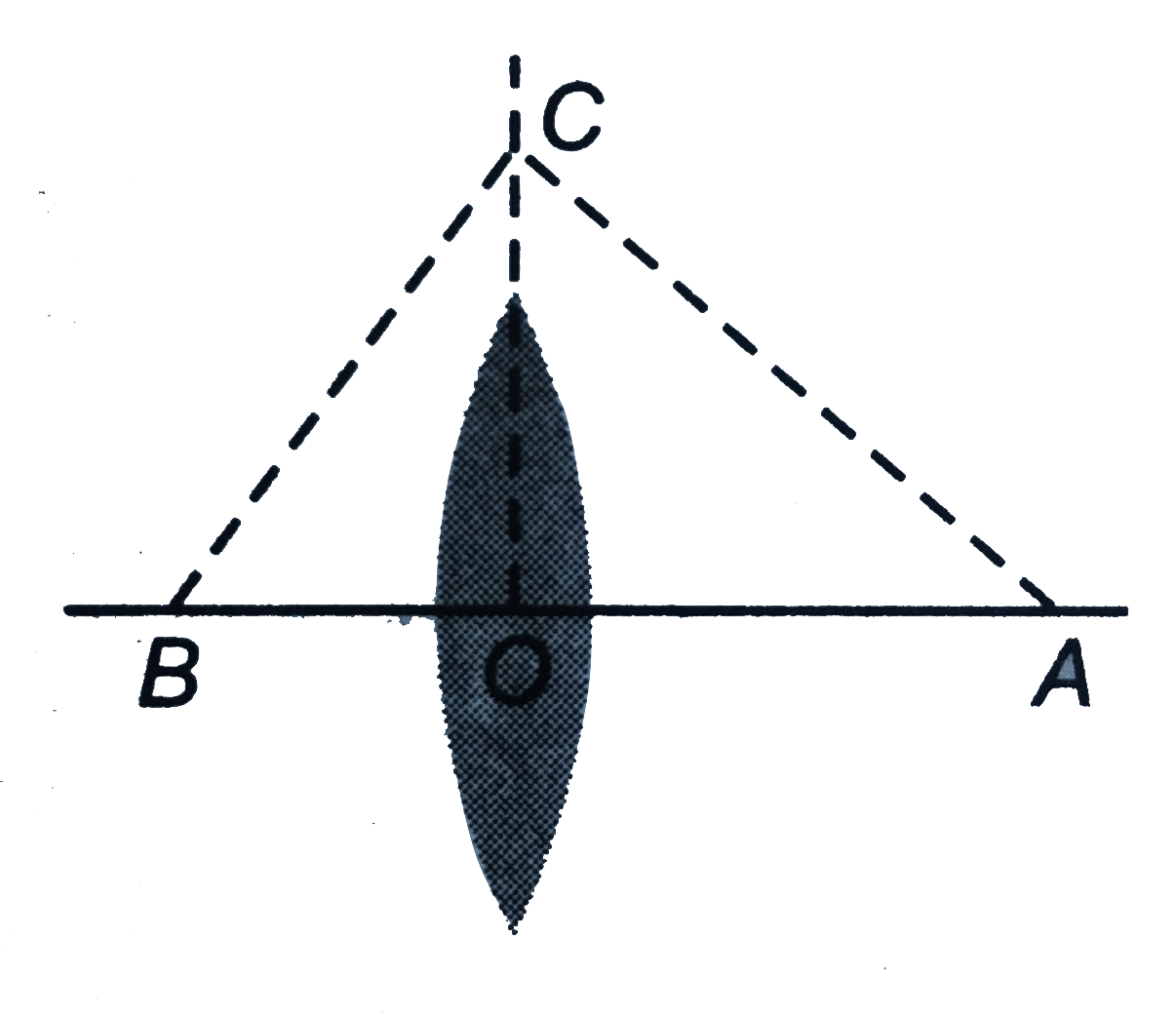
ABOC2
(AC)(BC)OC
OC2AB
(OC)(AB)AC+BC
The correct Answer is:C
1f=1OB−1−OA=1OB+1OA
f=(OA)(OB)AB+OB
∴f=(OA)(OB)AB….(i)
Now, AB2=AC2+BC2
or (OA+OB)2=AC2+BC2
or OA2+OB2+2(OA)(OB)=AC2+BC2
∴(AC2−OC2)+(BC2−OC2)
+2(OA)(OB)=AC2+BC2
Solving, we get
(OA)(OB)=OC2
Substituting in Eq. (i), we get
f=OC2AB
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Single Correct Option
3 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - more than one correct option
1 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Subjective Questions
8 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
Similar Questions
An object is placed at A(OA>f). Here, f is the focal length of the lens. The image is formed at B. A perpendicular is erected at O and C is chosen such that ∠BCA=90∘. Let OA = a, OB = b and OC = c. Then the value of f is
View SolutionThe power of lens is P=1f where f is focal length of the lens. The dimensions of power of lens is
View SolutionThe power of lens is P=1f' where f is focal length of the lens. The dimensions of power of lens is
View SolutionAn object is placed at a distance of f2 from a convex lens of focal length f. The image will be
View SolutionAn object is placed at a distance f in front of a concave lens of focal length f. Locate its image.
View SolutionAn object is placed at f/2 away from first focus of a convex lens where f is the focal length of the lens. Its image is formed at a distance 3f/2 in a slab of refractive index 3/2 , from the face of the slab facing the lens. Find the distance of this face of the slab from the second focus of the lens.
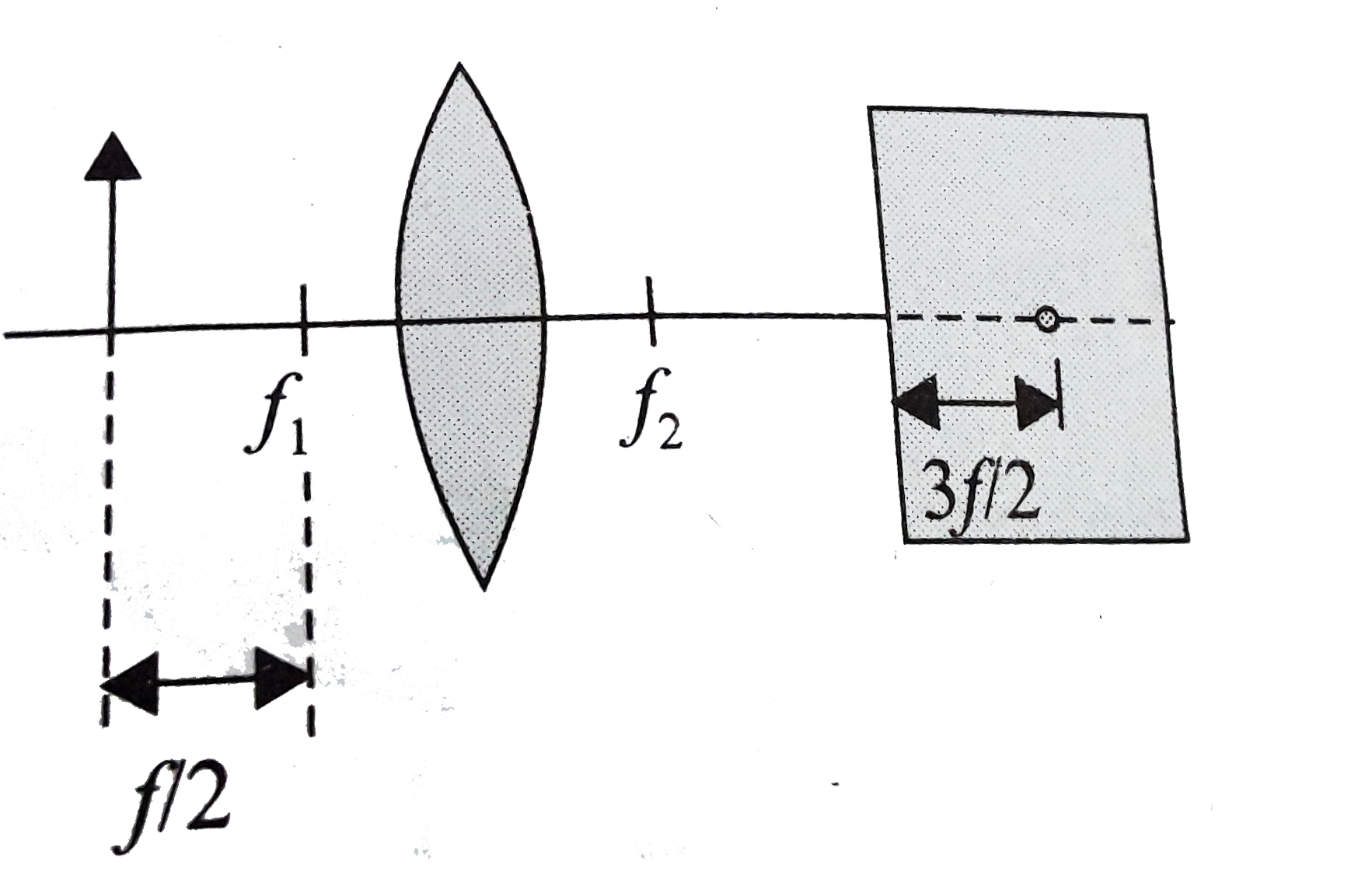
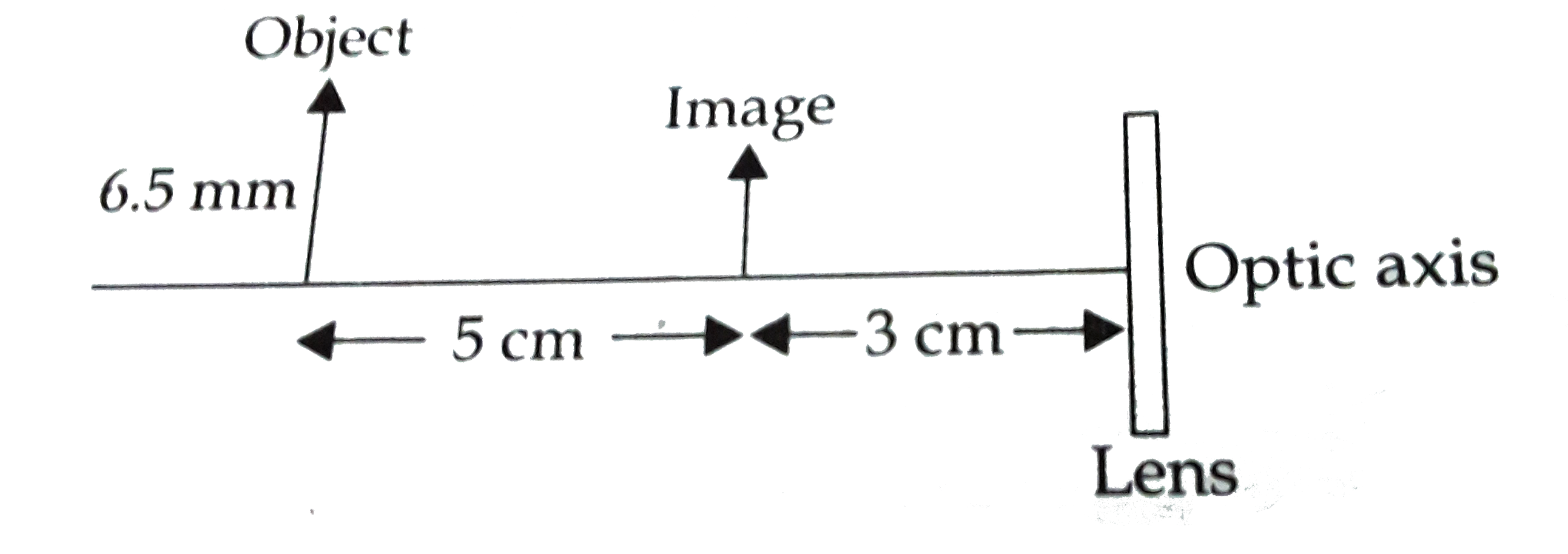
View SolutionShown an object and its image formed by a thin lens. Then the nature and focal length of lens is
View SolutionAn object is placed at a distance f in the front of a convex mirror. If focal length of the mirror is f, then distance of image from pole of the mirror is
View SolutionA linear object is placed along the axis of a mirror as shown in figure. If 'f' is the focal length of the mirror then the length of image is-

View SolutionAn object is placed at a distance of f/2 from a convex lens. The image will be
View Solution
