On a horizontal rought rood , value of coefficient of friction μ=0.4.Find the minimum time in which a distance of 400m can be covered. The car start from rest and finally comes to rest.
Maximum friction on horizontal rough road ,fmax=μmg
∴ Maximum acceleration or retardation of the car may be
amax or a=fmaxm=μmgm=μg
=0.4×10=4m/s2
Let , the rest car acceleration and retards for time 't' with 4m/s2
Then, 12at2+12at2=400m
or at2=400m or 4t2=400
or t=10s
Therefore the minimum time is 20s(10s of acceleration and 10sof retardation).
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Miscellaneous Examples9videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Exercise 8.110videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
- BOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective18videos
LAWS OF THERMODYNAMICS
Similar Questions
A car starts from rest on a 2−km long bridge. The coefficient of friction is 0.5. Find the minimum time to cross the bridge.
View SolutionA block of mass m moving with a velocity v0 on a rough horizontal surface from x=0 coefficient of friction is given by μ=μ0=ax. Find the kinetic energy of the block as function of x before it comes to rest.
View Solution
Knowledge Check
- Question 1 - Select One
A car starts from rest to cover a distance s. the coefficient of friction between the road and the tyres is μ. The minimum time in which the car can cover the distance is proportional to
AμB√μC1μD1√μ - Question 2 - Select One
A small collar of mass m is given an intial velocity of magnitude v0 on the horizontal circular track fabricated from a slender rod. If the coefficient of kinetic friction is μK, determine the distance travelled before the collar comes to rest. (Recognize that the friction force depends on the net normal force).
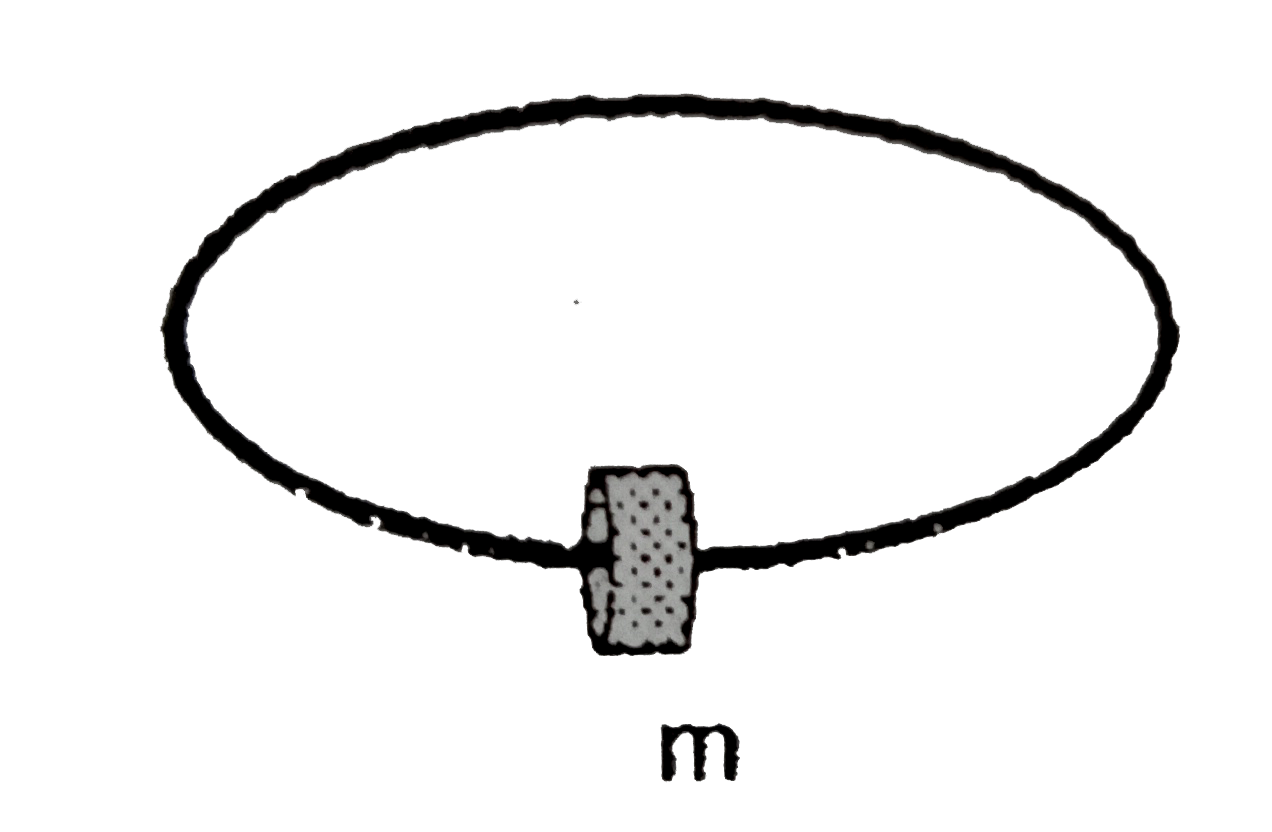 Arμkln⎡⎢ ⎢⎣v20+√v40+r2g2rg⎤⎥ ⎥⎦Br4μkln⎡⎢ ⎢⎣v20+√v40+r2g2rg⎤⎥ ⎥⎦C2rμkln⎡⎢ ⎢⎣v20+√v40+r2g2rg⎤⎥ ⎥⎦Dr2μkln⎡⎢ ⎢⎣v20+√v40+r2g2rg⎤⎥ ⎥⎦
Arμkln⎡⎢ ⎢⎣v20+√v40+r2g2rg⎤⎥ ⎥⎦Br4μkln⎡⎢ ⎢⎣v20+√v40+r2g2rg⎤⎥ ⎥⎦C2rμkln⎡⎢ ⎢⎣v20+√v40+r2g2rg⎤⎥ ⎥⎦Dr2μkln⎡⎢ ⎢⎣v20+√v40+r2g2rg⎤⎥ ⎥⎦ - Question 3 - Select One
A car, starting from rest, has a constant acceleration for a time interval t1 during which it covers a distance In the next time interval the car has a constant retardation and comes to rest after covering a distance in time Which of the following relations is correct?
Aa1a2=s1S2=t1t2Ba1a2=s2s1=t1t2Ca1a2=s1s2=t2t1Da1a2=s2s1=t2t1
A block of mass m rests on a horizontal floor for which coefficient of friction is μ . Find the magnitude and the direction of force that should be applied to just move the block so that the applied force is minimum.
View SolutionThe coefficient of friction between the tyres and road is 0.4. The minimum distance covered before attaining a speed of 8 ms−1 starting from rest is nearly (take, g=10 ms−2)
View SolutionThe coefficient of friction between the tyres and road is 0.4. The minimum distance covered before attaining a speed of 8ms−1 starting from rest is nearly ( take g=10ms−2)
View SolutionTwo block mandM tied together with an inextensible string are placed at rest on a rought horizontal surface with coefficient of friction μ The block m is pulled with a variable force F at a varying angle θ with the horizontal .The value of θ at which the least value of F is required to move the blocks given by
 View Solution
View SolutionA ball is dropped from a height of 3m. If coefficient of restitution between the surface and ball is 0.5, then the total distance covered by the before it comes to rest is
View Solution

