Particle A is moving along X-axis. At time t = 0, it has velocity of 10ms−1 and acceleration −4ms−2. Particle B has velocity of 20ms−1 and acceleration −2ms−2. Initially both the particles are at origion. At time t = 2 distance between the particles are at origin. At time t = 2 s distance between the particles is
24 m
36 m
20 m
42 m
The correct Answer is:A
To solve the problem, we need to find the positions of both particles A and B at time t=2 seconds and then calculate the distance between them.
Step 1: Calculate the position of Particle A at t=2 seconds
The formula for the position s of a particle under uniform acceleration is given by:
s=ut+12at2
For Particle A:
- Initial velocity uA=10m/s
- Acceleration aA=−4m/s2
- Time t=2s
Substituting the values into the formula:
sA=10⋅2+12⋅(−4)⋅(22)
Calculating this step-by-step:
1. Calculate 10⋅2=20
2. Calculate (22)=4
3. Calculate 12⋅(−4)⋅4=−8
4. Combine the results: sA=20−8=12m
Step 2: Calculate the position of Particle B at t=2 seconds
For Particle B:
- Initial velocity uB=20m/s
- Acceleration aB=−2m/s2
- Time t=2s
Using the same formula:
sB=20⋅2+12⋅(−2)⋅(22)
Calculating this step-by-step:
1. Calculate 20⋅2=40
2. Calculate (22)=4
3. Calculate 12⋅(−2)⋅4=−4
4. Combine the results: sB=40−4=36m
Step 3: Calculate the distance between the two particles
Now that we have the positions of both particles:
- Position of Particle A: sA=12m
- Position of Particle B: sB=36m
The distance between the two particles is:
Distance=sB−sA=36−12=24m
Final Answer
The distance between the particles at t=2 seconds is 24m.
---
The formula for the position s of a particle under uniform acceleration is given by:
- Initial velocity uA=10m/s
- Acceleration aA=−4m/s2
- Time t=2s
2. Calculate (22)=4
3. Calculate 12⋅(−4)⋅4=−8
4. Combine the results: sA=20−8=12m
For Particle B:
- Initial velocity uB=20m/s
- Acceleration aB=−2m/s2
- Time t=2s
2. Calculate (22)=4
3. Calculate 12⋅(−2)⋅4=−4
4. Combine the results: sB=40−4=36m
Now that we have the positions of both particles:
- Position of Particle B: sB=36m
The distance between the particles at t=2 seconds is 24m.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (B) Meical entrance special format questions (Assertion and reason)19videos
MOTION IN A PLANE
- BOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (B) Meical entrance special format questions (Mathch the columns)6videos
MOTION IN A PLANE
- BOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Check point 3.715videos
MOTION IN A PLANE
- BOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - Medical entrances gallery19videos
MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective10videos
PROJECTILE MOTION
Similar Questions
A particle has initial velocity 4i+4jms−1 and an acceleration −0.4ims−2, at what time will its speed bbe 5ms−1?
View SolutionTwo particles are moving along x-axis. Particle-1 is 40m behind Particle-2. Particle-1 starts with velocity12m/s and acceleration 4m/s2 both in positive x-direction. Particle-2 starts with velocity 4m/s and acceleration 12m/s2 also in positive x-direction. Find
(a) the time when distance between them is minimum.
(b) the minimum distacne between them.View SolutionA particle is moving east wards with a velocity of 15ms−1. In a time of 10 s, the velocity changes to 15ms−1 northwards. Average acceleration during this time is (in ms−2)
View SolutionThe acceleration of a particle is increasing linearly with time t as bt. The particle starts from the origin with an initial velocity v0. The distance travelled by the particle in time t will be
View SolutionA particle is moving along a circle and its linear velocity is 0.5ms when its angular velocity is 2.5rads−1. Its tangential acceleration is 0.2ms−2 then its angular acceleration is
View SolutionThe velocity of particle A is 0.1 ms−1 and that of particle B is 0.05 ms−1 if the mass of the particle B is five times that of particle A the ratio of De Broglie wavelengths associtated with the particles A and B is
View SolutionThe acceleratin of a particle increases linearly with time t as 6t. If the initial velocity of the particles is zero and the particle starts from the origin, then the distance traveled by the particle in time t will be
View SolutionIf a particle starts moving with initial velocity u=1ms−1 and acceleration a=2ms−2, the veloctiy of the particle at any time is given by v=u+at=1+2t. Plot the velocity-time graph of the particle.
View SolutionThe acceleration time graph of a particle is shown in the Fig. 2 (CF). 11. At time t=0s velocity of the particle is 8ms−1. Its velocity t=10s is.
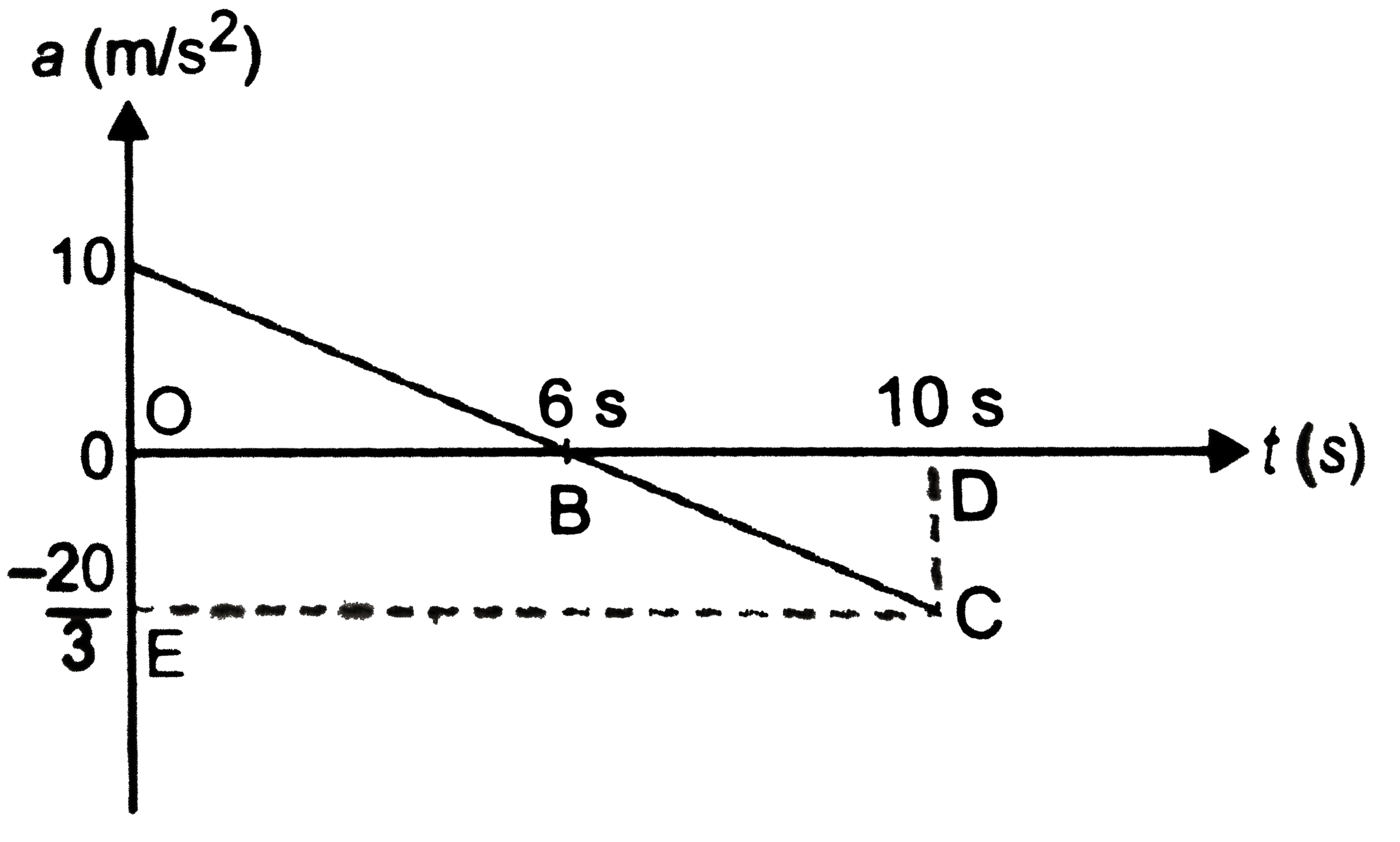 .View Solution
.View SolutionVelocity and acceleration of a particle at some instant of time are v=(3ˆi+4ˆj)ms−1 and a=−(6ˆi+8ˆj)ms−2 respectively. At the same instant particle is at origin. Maximum x-coordinate of particle will be
View Solution
