Prove that
Refractive index=real depthapparent depth
To prove that the refractive index (μ) is equal to the ratio of real depth to apparent depth, we can follow these steps:
1. Understanding the Setup:
- Consider two mediums: Medium 1 (with refractive index μ1) and Medium 2 (with refractive index μ2).
- An object is placed at a depth (real depth) 'h' in Medium 1, and an observer is looking at it from Medium 2.
2. Defining Depths:
- Let the real depth (the actual distance from the object to the surface) be represented as 'h'.
- Let the apparent depth (the depth at which the object appears to be when viewed from Medium 2) be represented as 'h''.
3. Drawing the Ray Diagram:
- Draw a ray from the observer's eye to the object. This ray will strike the surface separating the two mediums.
- At the point of incidence, let the angle of incidence be 'i' and the angle of refraction be 'r'.
4. Applying Snell's Law:
- According to Snell's Law:
μ2⋅sin(i)=μ1⋅sin(r)
5. Using Small Angle Approximation:
- For small angles, we can use the approximation:
sin(i)≈tan(i)andsin(r)≈tan(r)
- Therefore, we can rewrite Snell's Law as:
μ2⋅tan(i)=μ1⋅tan(r)
6. Relating Tangents to Depths:
- The tangent of angle 'i' can be expressed as:
tan(i)=xh
- The tangent of angle 'r' can be expressed as:
tan(r)=xh′
- Here, 'x' is the horizontal distance from the observer to the point directly above the object.
7. Substituting in Snell's Law:
- Substitute the expressions for tan(i) and tan(r) into Snell's Law:
μ2⋅xh=μ1⋅xh′
8. Cancelling x:
- Since 'x' is common on both sides, we can cancel it out (assuming x ≠ 0):
μ2⋅1h=μ1⋅1h′
9. Rearranging the Equation:
- Rearranging gives:
μ2μ1=hh′
10. Conclusion:
- If we take Medium 1 as air (where μ1 = 1), we have:
μ2=hh′
- Thus, we conclude that:
Refractive Index(μ)=Real DepthApparent Depth
- Consider two mediums: Medium 1 (with refractive index μ1) and Medium 2 (with refractive index μ2).
- An object is placed at a depth (real depth) 'h' in Medium 1, and an observer is looking at it from Medium 2.
- Let the real depth (the actual distance from the object to the surface) be represented as 'h'.
- Let the apparent depth (the depth at which the object appears to be when viewed from Medium 2) be represented as 'h''.
- Draw a ray from the observer's eye to the object. This ray will strike the surface separating the two mediums.
- At the point of incidence, let the angle of incidence be 'i' and the angle of refraction be 'r'.
- According to Snell's Law:
μ2⋅sin(i)=μ1⋅sin(r)
- For small angles, we can use the approximation:
sin(i)≈tan(i)andsin(r)≈tan(r)
- Therefore, we can rewrite Snell's Law as:
μ2⋅tan(i)=μ1⋅tan(r)
- The tangent of angle 'i' can be expressed as:
tan(i)=xh
- The tangent of angle 'r' can be expressed as:
tan(r)=xh′
- Here, 'x' is the horizontal distance from the observer to the point directly above the object.
- Substitute the expressions for tan(i) and tan(r) into Snell's Law:
μ2⋅xh=μ1⋅xh′
- Since 'x' is common on both sides, we can cancel it out (assuming x ≠ 0):
μ2⋅1h=μ1⋅1h′
- Rearranging gives:
μ2μ1=hh′
- If we take Medium 1 as air (where μ1 = 1), we have:
μ2=hh′
- Thus, we conclude that:
Refractive Index(μ)=Real DepthApparent Depth
Topper's Solved these Questions
- REFRACTION OF LIGHT AT PLANE SURFACESBOOK - ICSECHAPTER - REFRACTION OF LIGHT AT PLANE SURFACESEXERCISE - EXERCISE-4(C) MULTIPLE CHOICE TYPE
2 Videos
- REFRACTION OF LIGHT AT PLANE SURFACESBOOK - ICSECHAPTER - REFRACTION OF LIGHT AT PLANE SURFACESEXERCISE - EXERCISE-4(C) NUMERICALS
3 Videos
- REFRACTION OF LIGHT AT PLANE SURFACESBOOK - ICSECHAPTER - REFRACTION OF LIGHT AT PLANE SURFACESEXERCISE - EXERCISE-4(B) NUMERICALS
2 Videos
- REFRACTION OF LIGHTBOOK - ICSECHAPTER - REFRACTION OF LIGHTEXERCISE - DIAGRAM BASED MCQ:
5 Videos
- REFRACTION THROUGH A LENS BOOK - ICSECHAPTER - REFRACTION THROUGH A LENS EXERCISE - EXERCISE-5(D) (MULTIPLE CHOICE TYPE)
2 Videos
Similar Questions
A vessel of depth 2d cm is half filled with a liquid of refractive index μ1 and the upper half with a liquid of refractive index μ2. The apparent depth of the vessel seen perpendicularly is
View SolutionA small object is placed at the centre of the bottom of a cylindrical vessel of radius 3 cm and height 4 cm filled completely with water. Consider the ray leaving the vessel through a corner. Suppose this ray and the ray along the axis of the vessel are used to trace the image. Find the apparent depth of the image and the ratio of real depth to the apparent depth under the assumptions taken. Refractive index -of water = 1-33.
View Solution
Knowledge Check
A vesse of depth x is half filled with oil of refractive index μ1 and the other half is filled with water of refrative index μ2. The apparent depth of the vessel when viewed above is
Ax(μ1+μ2)2μ1μ2Bxμ1μ22(μ1+μ2)Cxμ1μ2(μ1+μ2)D2x(μ1+μ2)μ1μ2
A vessle of depth d is first filled to (23)rd of its depth with a liquid of refractive index 32 and the rest of the vessel is filled with a liquid of refractive index 43. What is the apparent depth of the inner surface of the bottom of the vessle.
View SolutionA vessle of depth d is first filled to (23)rd of its depth with a liquid of refractive index 32 and the rest of the vessel is filled with a liquid of refractive index 43. What is the apparent depth of the inner surface of the bottom of the vessle.
View SolutionA vessel is quarter filled with a liquid of refractive index μ. The remaining parts of the vessel is filled with an immiscible liquid of refractive index 3μ/2. The apparent depth of the vessel is 50% of the actual depth. The value of μ is
View SolutionA parallel sided block of glass of refractive index 1.5 which is 36 mm thick rests on the floor of a tank which is filled with water (refractive index = 4/3). The difference between apparent depth of floor at A and B when seen from vertically above is equal to

View SolutionA tank contains three layers of immiscible liquids. The first layer is of water with refractive index 4/3 and thickness 8cm. The second layer is of oil with refractive index 3/2 and thickness 9cm while the third layer is of glycerine with refractive index 2 and thickness 4cm. Find the apparent depth of the bottom of the container.
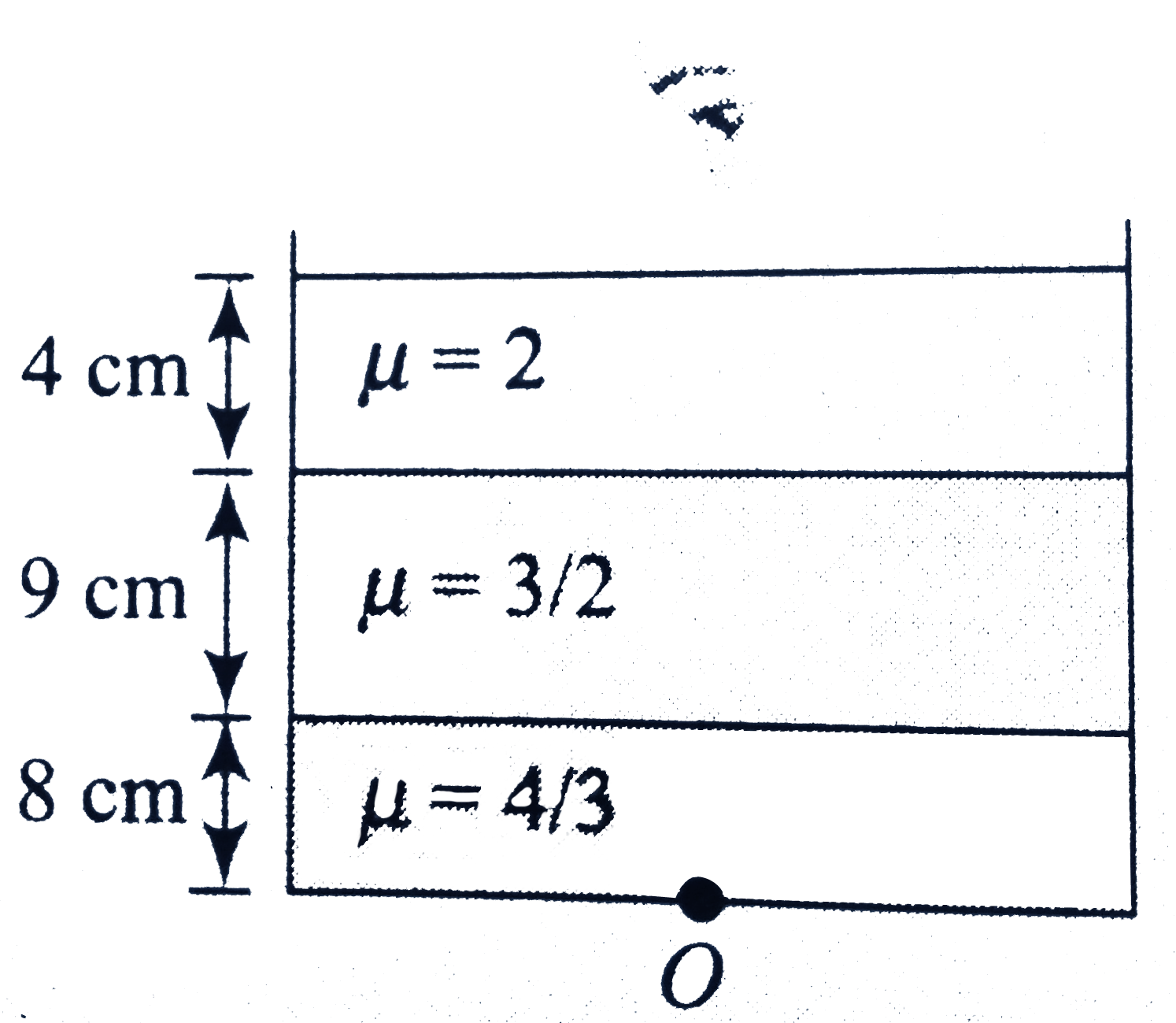
View SolutionIn a pond of water, a flame is held 2 m above the surface of the water. A fish is at depth of 4 m from the water surface. Refractive index of water is 43. The apparent height of the flame from the eyes of fish is -
View SolutionA travelling microscope is focussed on to a scratch on the bottom of a beaker. Water of refractive index 43 is poured in it. Then the microscope is to be lifted through 2 cm focus it again. Find the depth of water in the beaker.
View Solution
