Siddharth wanted to see his inverted image in a plane mirror. So, he stood between a concave mirror and a plane mirror facing each other as shown in the figure. Knowing the focal length of the concave mirror, he found the distance between him and his inverted virtual image, formed in the plane mirror. What is his answer?
To solve the problem, we need to determine the distance between Siddharth and his inverted virtual image formed in the plane mirror after the concave mirror has created an image of him. We will follow these steps:
Step 1: Identify the Focal Length and Object Distance
- The focal length f of the concave mirror is given as −2 meters (negative because it is a concave mirror).
- The object distance u (the distance from Siddharth to the concave mirror) is given as −3 meters (also negative, as per the sign convention).
Step 2: Use the Mirror Formula
The mirror formula is given by:
1f=1v+1u
Where:
- f = focal length
- v = image distance from the mirror
- u = object distance from the mirror
Step 3: Substitute the Values into the Formula
Substituting f=−2 m and u=−3 m into the formula:
1−2=1v+1−3
Step 4: Rearranging the Equation
Rearranging gives:
1v=1−2+13
Step 5: Find a Common Denominator
The common denominator for −2 and 3 is −6:
1v=−36+26=−16
Step 6: Solve for v
Taking the reciprocal gives:
v=−6 meters
This means the image formed by the concave mirror is 6 meters in front of the mirror, and since it is negative, it indicates that the image is real and inverted.
Step 7: Determine the Position of the Image in the Plane Mirror
The image formed by the concave mirror acts as an object for the plane mirror. The distance from Siddharth to the plane mirror is 3 meters (the distance from Siddharth to the concave mirror) plus 6 meters (the distance of the image from the concave mirror):
Total distance=3+6=9 meters
Step 8: Calculate the Image Distance from the Plane Mirror
In a plane mirror, the image distance is equal to the object distance. Therefore, the virtual image will be located 9 meters behind the plane mirror.
Step 9: Calculate the Total Distance Between Siddharth and His Inverted Image
The distance between Siddharth and his virtual image is the distance from Siddharth to the plane mirror plus the distance from the plane mirror to the virtual image:
Distance=3+9=12 meters
Final Answer
The distance between Siddharth and his inverted virtual image formed in the plane mirror is 12 meters.
---
- The focal length f of the concave mirror is given as −2 meters (negative because it is a concave mirror).
- The object distance u (the distance from Siddharth to the concave mirror) is given as −3 meters (also negative, as per the sign convention).
The mirror formula is given by:
1f=1v+1u
Where:
- f = focal length
- v = image distance from the mirror
- u = object distance from the mirror
Substituting f=−2 m and u=−3 m into the formula:
1−2=1v+1−3
Rearranging gives:
1v=1−2+13
The common denominator for −2 and 3 is −6:
1v=−36+26=−16
Taking the reciprocal gives:
v=−6 meters
This means the image formed by the concave mirror is 6 meters in front of the mirror, and since it is negative, it indicates that the image is real and inverted.
The image formed by the concave mirror acts as an object for the plane mirror. The distance from Siddharth to the plane mirror is 3 meters (the distance from Siddharth to the concave mirror) plus 6 meters (the distance of the image from the concave mirror):
Total distance=3+6=9 meters
In a plane mirror, the image distance is equal to the object distance. Therefore, the virtual image will be located 9 meters behind the plane mirror.
The distance between Siddharth and his virtual image is the distance from Siddharth to the plane mirror plus the distance from the plane mirror to the virtual image:
Distance=3+9=12 meters
The distance between Siddharth and his inverted virtual image formed in the plane mirror is 12 meters.
Topper's Solved these Questions
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - Concept Application (Level 3)
15 Videos
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - SOLVED EXAMPLE
43 Videos
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - Concept Application (Level 1)
30 Videos
- KINEMATICS BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - KINEMATICS EXERCISE - CONCEPT APPLICATION (Level 3) (Application Based Questions )
5 Videos
- MAGNETISM BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MAGNETISM EXERCISE - CONCEPT APPLICATION (Level 3)
4 Videos
Similar Questions
A concave mirror and a plane mirror are arranged facing each other and an object AB is placed between them as shown in the figure. What is the distance between the object and its inverted, virtual image formed in plane mirror?
View SolutionA convex mirror and a plane mirror are arranged facing an object as shown in the figure below. If there is no parallax between the images formed by the two mirrors, then find the radius of curvature of the convex mirror?
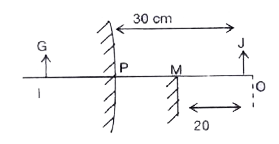
View Solution
Knowledge Check
The focal length of a concave mirror is 50cm. Where an object be placed so that its image is two times and inverted
A75 cmB72 cmC63 cmD50 cmFor the system as shown in the figure, the image formed by the concave mirror
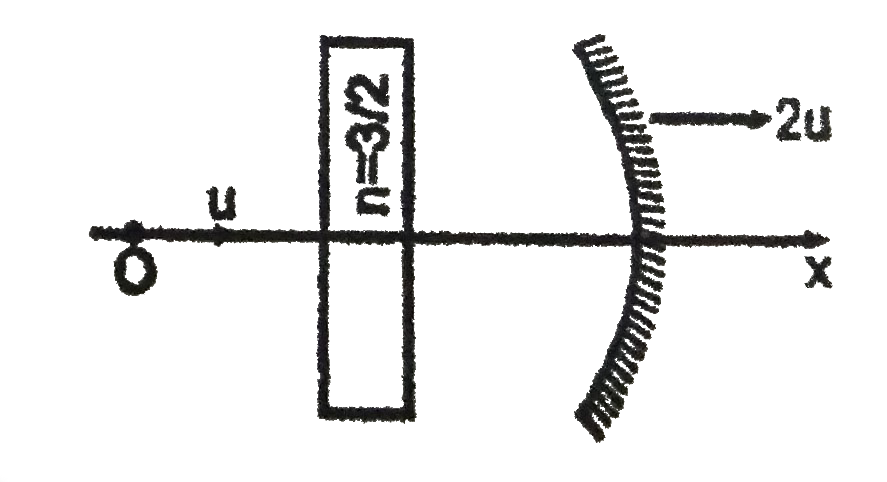 Awill have speed greater than the speed of the objectBmay have speed greater than the speed the objectCwill have speed less than the speed of the mirrorDmoves away from the mirror
Awill have speed greater than the speed of the objectBmay have speed greater than the speed the objectCwill have speed less than the speed of the mirrorDmoves away from the mirrorWe have a concave mirror of 10 cm focal length. Minimum distance between a real object and its real image formed by the mirror will be-
A10 cmB20 cmC40 cmD0
An object is placed between a plane mirror and a concave mirror of focal length 15 cm as shown in Figure . Find the position of the images after two reflections.

View SolutionIf you want to see an enlarged image of your face, state whether you will use a concave mirror or a convex mirror ?
View SolutionAssertion: A plane mirror can form a real and inverted image.
Reason : A virtual image is always formed behing the plane mirror.
View SolutionAssertion: A plane mirror can form a real and inverted image.
Reason : A virtual image is always formed behing the plane mirror.
View SolutionA convex mirror of length 1m and a plane mirror are facing each other, if the distance between them is 1m, their images are separated by
View Solution
