The center of mass is located at position i EX.5.4. Locate the center of mass of a uniform rod of mass M and length Solution in Tamil
Consider a uniform rod of mass M and length / whose one end coincides with the origin as shown in Figure. The rod is kept along the x axis. To find the center of mass of this rod, we choose an infinitesimally small mass dm of elemental length dr at a distance from the origin
λ is the linear mass density (ie, mass per unit length) of the rod. λ=Ml
The mass of small element (dm) is dm,Mldx
Now, we can write the center of mass equation for this mass distribution as,
XCM=∫xdxm∫dm
XCM=1∫0x(Mldx)M=1l1∫0xdx=1l[x22]l0=1l=(t22)
XCM=l2
As the l2 position is the geometric center of the rod, it is concluded that the center of mass

Topper's Solved these Questions
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODIESBOOK - FULL MARKSCHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODIESEXERCISE - TEXTUAL QUESTIONS SOLVED ( MULTIPLE CHOICE QUESTIONS:)
15 Videos
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODIESBOOK - FULL MARKSCHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODIESEXERCISE - TEXTUAL QUESTIONS SOLVED ( SHORT ANSWER QUESTIOS)
17 Videos
- LAW OF MOTION BOOK - FULL MARKSCHAPTER - LAW OF MOTION EXERCISE - ADDITIONAL QUESTIONS SOLVED (SHORT ANSWER QUESTIONS (3 MARKS & NUMERICALS))
16 Videos
- NATURE OF PHYSICAL WORLD AND MEASUREMENT BOOK - FULL MARKSCHAPTER - NATURE OF PHYSICAL WORLD AND MEASUREMENT EXERCISE - ADDITIONAL QUESTIONS SOLVED ( SHORT ANSWER QUESTIONS (2 MARK))
20 Videos
Similar Questions
The centre of mass of a system of particles does not depend upon
View SolutionDefine the center of mass of a body.
View SolutionA block of mass 500 g is at rest on a horizontal table. What steady force is required to give the block a velocity of 200cms−1 in 4 s?
View SolutionThe vernier scale of a travelling microscope has 50 divisions which coincide with 49 main scale divisions. If each main scale division is 0.5 mm. Calculate the minimum inaccuracy in the measurement of distance.
View SolutionLocate the center of mass.
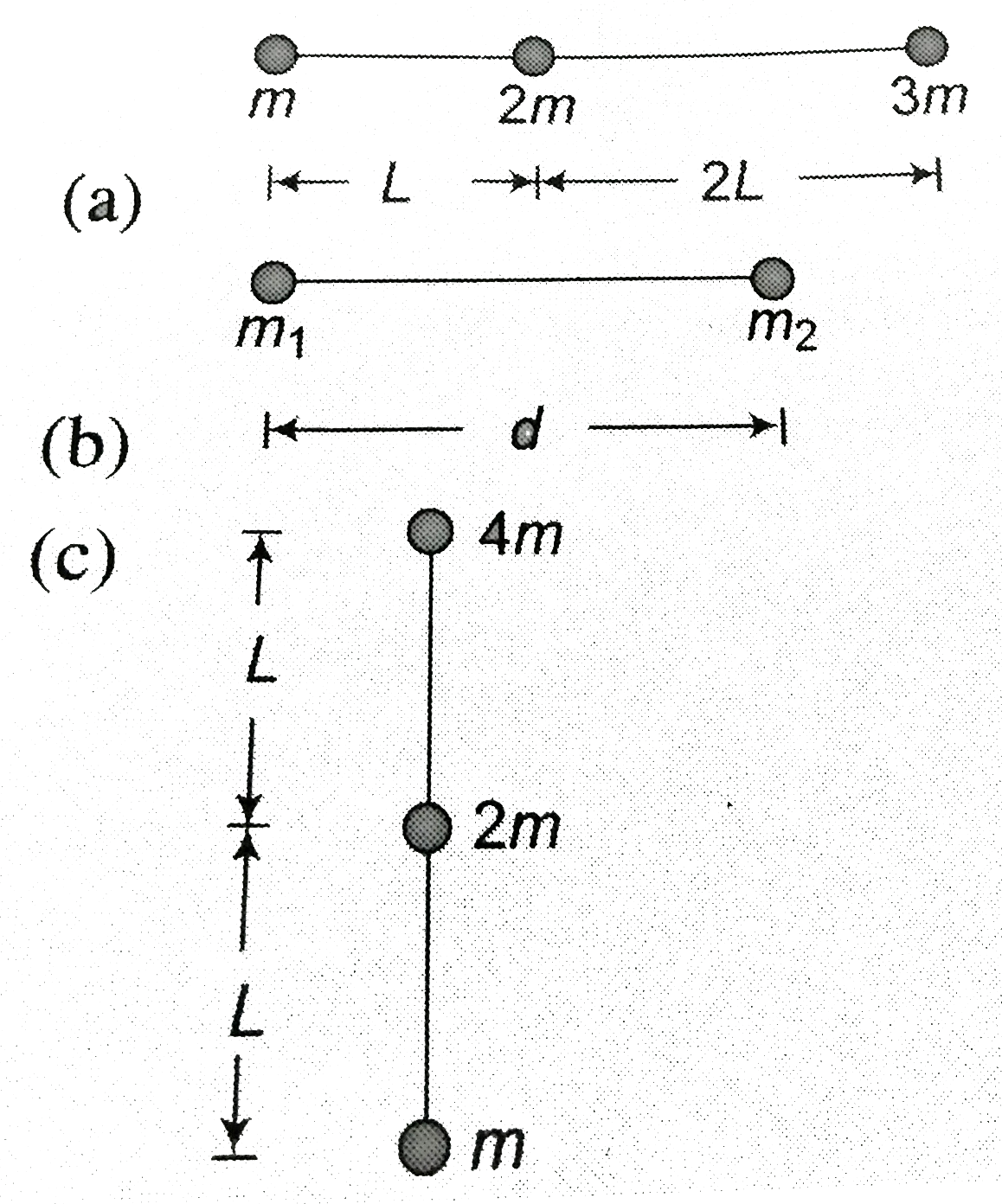
View SolutionMass center of a system of a segment of a uniform circular rod(arc)
Find location of mass center of a thin uniform rod bent into shape of an arc.
View SolutionMass Center of a sector of a uniform circular plate Find location of mass center of a sector of a thin uniform plate.
View SolutionA uniform rod of length 10meters and mass 20 kilograms is balanced on a fulcrum with a 40-kilogram mass on one end of the rod and a 20-kilogram mass on the other end, as shown. How far is the fulcrum located from the center of the rod?
View Solutionद्रव्यमान केन्द्र की परिभाषा दीजिए। L लम्बाई की एकसमान छड़ के द्रव्यमान केन्द्र की स्थिति का निगमन कीजिए ।
View SolutionThe center of mass for a uniform rod of mass M and length 12 i.e., 0.5 l lies at the
View SolutionThe center of mass for a uniform rod of mass M and length 12 i.e., 0.5 l lies at the
View SolutionM द्रव्यमान का एक कण त्रिज्या a तथा M द्रव्यमान के गोलीय कोष के केंद्र पर स्थित है । केंद्र से a2 दूरी पर किसी बिंदु का गुरुत्वीय विभव होगा -
View Solutionएक समान पतली छड़ के द्रव्यमान केन्द्र की स्थिति लिखिए।
View Solutionप्रक्कथन : किसी वस्तु के द्रव्यमान केंद्र की स्थिति वस्तु की आकृति तथा आकार पर निर्भर नहीं करती।
कारण : किसी वस्तु का द्रव्यमान केंद्र हमेशा वस्तु के केंद्र पर स्थित होता है।
View Solutionn भुजाओं वाले बहुभुज के शीर्षो पर समान द्रव्यमान (m) के (n-1) बिंदु द्रव्यमान स्थित है। बहुभुज के द्रव्यमान केंद्र से खाली शीर्ष का स्थिति सदिश α है द्रव्यमान केंद्र का धनात्मक सदिश होगा
View Solution
