The electric field in an electromagnetic wave is given by E(50N(C−1))sinω(t−xc). Find the energy contained in a cylinder of cross section 10 cm^2 and length 50 cm along the x- axis. Solution in Gujrati
Solution:
The energy density is
(uav)=((12(ɛ0)(E20))=(12×(8.85×(10−12)(C2)(N−1)(m−2))×((50NC−1)2)
=1.1×(10−8)Jm−3.
The volume of the cylinder is
V=10(cm2)×50cm=5×(10−4)(m3).
The energy contained in this volume is
U=(1.1×(10−8)Jm−3)×(5×(10−4)m3)
= 5.5 xx (10^(-12))J.
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Worked Out Examples5videos
ELECTROMAGNETIC WAVES
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Short Answer7videos
ELECTROMAGNETIC WAVES
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE9videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - GAUSS LAWEXERCISE - Exercises24videos
GAUSS LAW
Similar Questions
A parallel- plate capacitor with plate area A and separation between the plates d, is charged by a constant current i. Consider a plane surface of area A/2 parallel to the plates and drawn summetrically between the plates. Find the displacement current through this area.
04:29View SolutionIn a microwave oven, the food is kept in a plastic
container and the microwave is directed towards the
food. The food is cooked without melting or igniting the
plastic container. Explain.04:38View SolutionShown a metallic square frame of edga a in a vertical plane. A uniform magnetic field B exists in the space in a directon perpendicular to the plane of the figure. Two boys pull the opposite corners of the square ot deform it into a rhombus. They start pulling the corners t t=0 and sisplace the corners at a uniform speed u. (a) find the induced emf in the frame at the instant when the angles at these corners reduce to 60∘. (b) find the induced current in the frame at this instatnt if the total resistance of the frame is R. (c) Find the total charge which flows through a side of the frame by the time the square is deformed into a straight line.
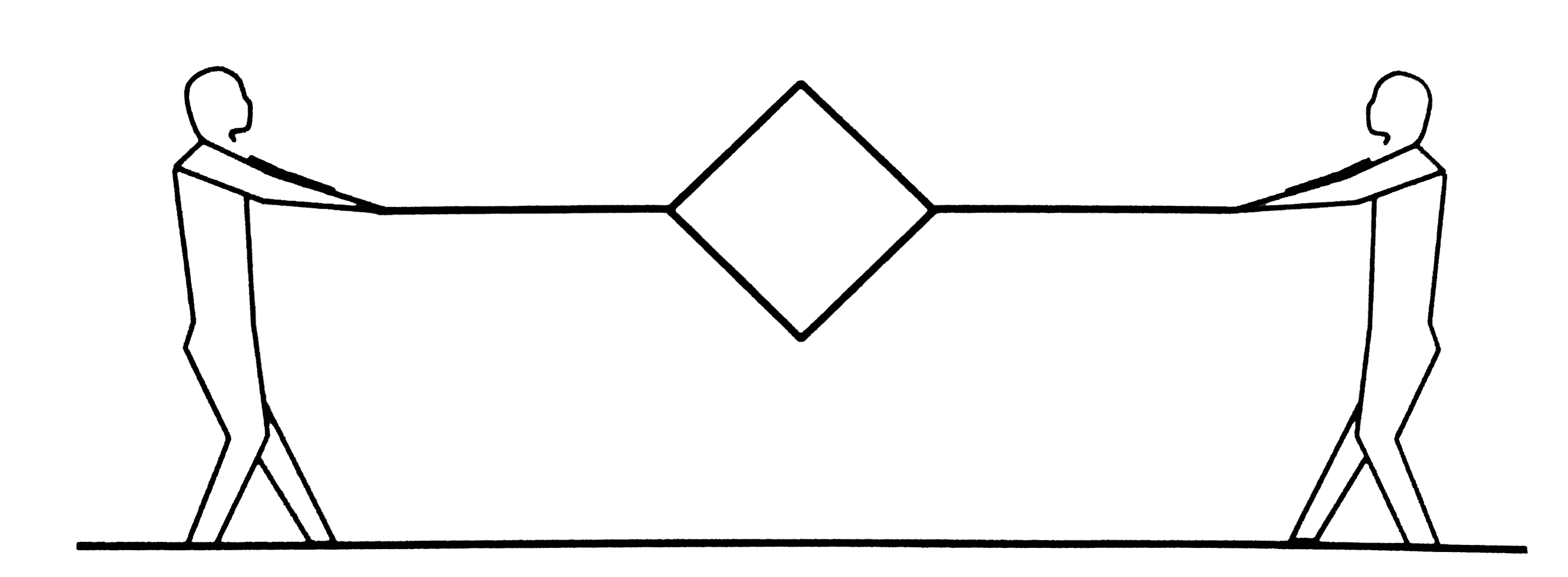 02:01View Solution
02:01View SolutionThe electric field in a region is given by →E=35E0→i+45E0→j with E0=2.0×103NC−1. Find the flux of this field through a recatngular surface of area 0.2m2 parallel to the y-z plane.
07:27View SolutionThe electric field in an electromagnetic wave is given by E(50N(C−1))sinω(t−xc). Find the energy contained in a cylinder of cross section 10 cm^2 and length 50 cm along the x- axis.
03:52View SolutionAn electric field in an e.m.wave is given by
E=200sin.2πλ(ct−x)NC−1.
Find the energy contained in a cylinder of crossection 20cm2 and length 40 cm along the x-axis.05:46View SolutionThe electric in an electromagetic wave is given by E=(100 N/C) sinomega(t-(X)/(C )) . If the energy contained in a cylinder of coss-section 10 cm^(2) and length 50 cm along the X-axis is 4.4xx10^(-8)J//m^(3) , then find intensity of the wave.
03:08View Solutionएक समतल वैद्युतचुम्बकीय तरंग में विद्युत क्षेत्र की समीकरण निम्न है।
E=50sin(ωt−kx) वोल्ट/मीटर
(i) दिक्स्थान में 10 सेमी2 परिच्छेद वाले उस बेलन में निहित। वैद्युतचुम्बकीय ऊर्जा ज्ञात कीजिये जिसकी लम्बाई 50 सेमी X-अक्ष के अनुदिश है।
(ii) तरंग की तीव्रता ज्ञात कीजिये।04:13View SolutionThe electric field in an electromagnetic wave is given by e = 50 sin ω(t−x/c)(inNC−1) Find the energy contained in a cylindrical space of cross-section 10 cm2 and length 100 cm along the x-axis. Also find the intensity of wave
03:14View SolutionElectric field intensity of an electromagnetic wave is represented as E= ( 100 N/C ) sin ( omega t - kx ) . Amount of energy stored in a cylindrical volume of cross section 10 cm^2 and length 100 cm , along X -axis is given by 0.443 xx 10^(-2x ) Joules . what is the value of x ?
09:42View SolutionThe electric field in an electromagnetic wave is given by E=(50N/C)sin(omega t-kx) .The energy contained in a cylinder of cross section 1m^(2) and length 25cm along the x axis is nearly
02:22View SolutionFind the energy contained in a cyllinder of cross - section 10cm2 and length 50 along x - axis, if E=50sinω(t−x/C) be the electric field in an electromagnetic wave
05:04View SolutionThe electric field of an electromagnetic wave is given by E=(50NC−1)sinω(t−x/c).
The energy contained in a cylinder of cross section 10cm2and length l /10 along the x-axis is 5.5×10−12J. The vlaue of l is03:24View SolutionAn electromagnetic wave of electric field E=10 sin (omega t-Kx)N//C is incident normal to the cross - sectional area of a cylinder of 10 cm^(2) and having length 100 cm, lying along X - axis. Find (a) the energy density, (b) energy contained in the cylinder, (c ) the intensity of the wave, (d) momentum transferred to the cross - sectional area of the cylinder in 1 s, considering total absorption, (e ) radiation pressure. [epsilon_(0)=8.854xx10^(12)C^(2)N^(-1)m^(-2), c=3xx10^(8)ms^(-1)]
02:25View SolutionThe electric field in an electromagnetic wave is given by E(50NC−1)sinω(t−xc). Find the energy contained in a cylinder of cross section 10 cm^2 and length 50 cm along the x- axis.
View SolutionThe electromagnetic field of electromagnetic wave is as follows E =(50 N//C) sin omega (t-x//c) x-direction 10 "सेमी"^(-2) What will be the energy contained in the cross-cut and 50 cm length cylinders?
View Solution

