The figure below is a representation of a simple harmonic progressive in the negative X-axis, at a given instant. The direction of the velocity of the particle at stage P in the figure is best represent by the arrow.
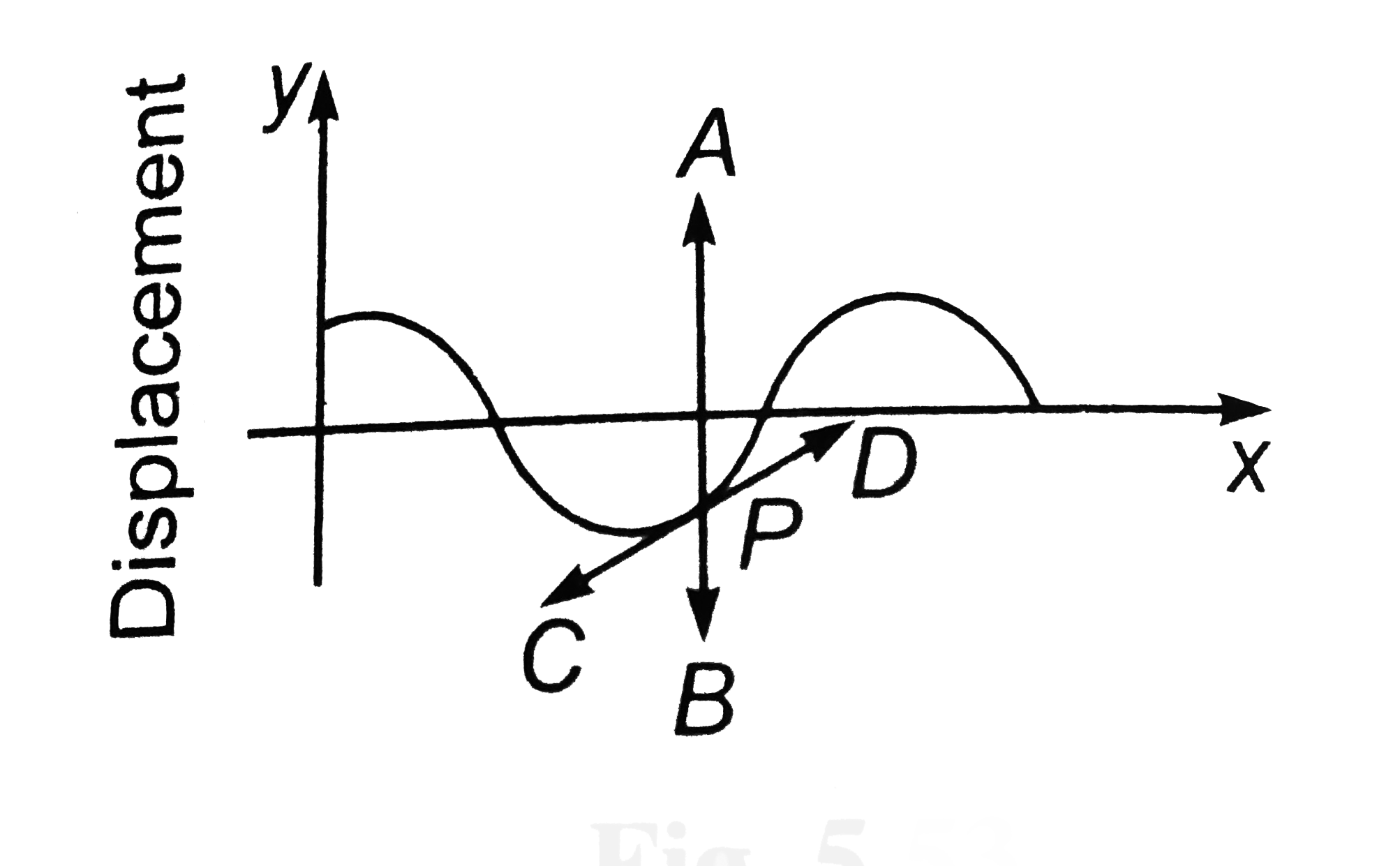
→PA
→PB
→PC
→PD
The correct Answer is:a
vp=−v(dydx)
here c is negative and dy/dx is positive at point P. Hence Vpis positive. So P is moving upward.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Single Correct Answer1videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Multiple Correct25videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Subjective16videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct9videos
TRANSMISSION OF HEAT
- BOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
A simple harmonic progressive wave is given by y=Asin(ωt−kx). What is (i) the particle velocity at a point x and time t (ii) the wave speed ?
View SolutionDerive an expression for a one - dimensional simple harmonic progressive wave travelling in the direction of the positive x - axis. Express it in terms of A,λ, v , t and x .
View Solution
Knowledge Check
- Question 1 - Select One
The equation of a simple harmonic progressive wave along the negative direction of X-axis is
Ay=asin2π[tT−xλ]By=asin(2πnt)Cy=asin2π[tT+xλ]Dy=acos2π[x] - Question 2 - Select One
The equation of a simple harmonic progressive wave along the positive direction of X axis is given by
Ay=asin2π[tT−xλ]By=asin(2πnt)Cy=asin2π[tT+xλ]Dy=acos(2πnt) - Question 3 - Select One
In a simple harmonic progressive wave, the maximum particle velocity is twice the wave velocity. If λ is the wavelength, then its amplitude is given by
AλπB2λπCλ2πD2πλ
The figure represents the instantaneous picture of a longitudinal harmonic wave travelling along the negative x-axis. Identify the correct statement related to the movement of the points shown in the figure.
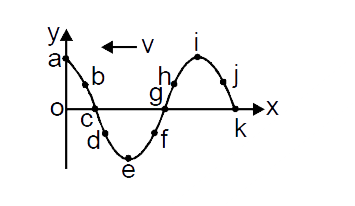
The stationary points areView SolutionThe equation of a simple harmonic progressive wave along the negative direction of X-axis is
View SolutionThe figure represents the instantaneous picture of a longitudinal harmonic wave travelling along the negative x-axis. Identify the correct statement related to the movement of the points shown in the figure.
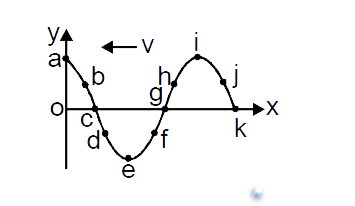
The points moving opposite to the direction of propagation areView SolutionThe figure represents the instantaneous picture of a longitudinal harmonic wave travelling along the negative x-axis. Identify the correct statement related to the movement of the points shown in the figure.
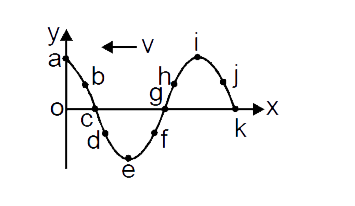
The points moving in the direction of wave areView SolutionA particle is moving parallel to x-axis as shown in the figure. The angular velocity of the particle about the origin is
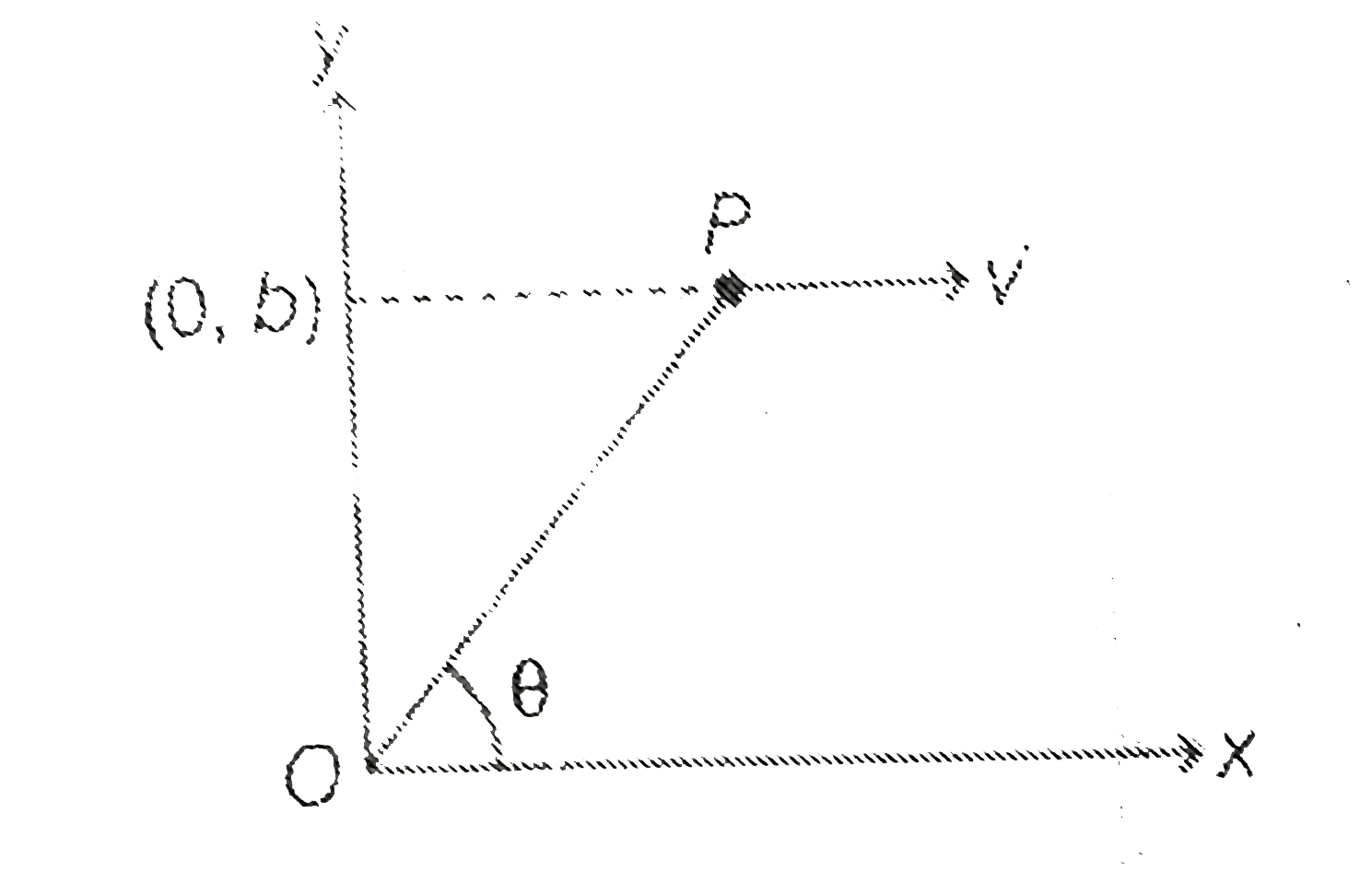 View Solution
View Solution

