The flat bottom of cylinder tank is silvered and water (μ=43) is filled in the tank upto a height h. A small bird is hovering at a height 3h from the bottom of the tank. When a small hole is opened near the bottom of the tank, the water level falls at the rate of 1 cm/s. The bird will perceive that his velocity of image is 1/x cm/sec (in downward directions) where x is
The correct Answer is:2
To solve the problem step by step, we will analyze the situation involving the bird, the water level, and the image formed by the silvered bottom of the tank.
Step 1: Understand the Setup
- The tank has a height of h filled with water (refractive index μ=43).
- The bird is hovering at a height of 3h from the bottom of the tank.
- The water level is falling at a rate of 1cms.
Step 2: Determine the Position of the Bird and Image
- The height of the water is h, and the bird is at 3h.
- The distance from the bird to the water surface is 3h−h=2h.
- The image of the bird will be formed below the water surface due to reflection from the silvered bottom.
Step 3: Calculate the Image Distance
- The distance of the image from the water surface can be calculated using the formula for the image distance in a spherical mirror:
\(
\frac{1}{f} = \frac{1}{v} + \frac{1}{u}
\)
Here, since the bottom is a plane mirror, the focal length f is infinite, and we can simplify the situation:
- The image distance v from the water surface is equal to the distance of the bird from the water surface, which is 2h.
Step 4: Consider the Effect of Falling Water Level
- As the water level falls at 1cms, the distance x2 (the distance from the water surface to the bottom of the tank) is decreasing.
- Let x1 be the distance from the bird to the water surface, which is 2h initially but will change as the water level falls.
Step 5: Relate the Rates of Change
- The rate of change of the water level (dx2dt) is −1cms (negative because it is falling).
- The distance x1 will also change as the water level falls, so we can express the distance from the bird to the image as:
\(
d = x1 + x2
\)
- The rate of change of the distance d with respect to time can be expressed as:
\(
\frac{dd}{dt} = \frac{dx1}{dt} + \frac{dx2}{dt}
\)
Step 6: Substitute the Rates
- Since dx2dt=−1cms, we need to find dx1dt.
- The distance x1 is initially 2h, and as the water level falls, x1 increases. Thus:
\(
\frac{dx1}{dt} = 1 cm/s
\)
- Therefore, substituting into the equation:
\(
\frac{dd}{dt} = 1 - 1 = 0
\)
Step 7: Calculate the Perceived Velocity of the Image
- The perceived velocity of the image by the bird is given by:
\(
\frac{d}{dt} \left( \frac{1}{x} \right) = \frac{1}{x^2} \cdot \frac{dd}{dt}
\)
- Since dddt is 0, the perceived velocity of the image is:
\(
\frac{d}{dt} \left( \frac{1}{x} \right) = 0
\)
Step 8: Find the Value of x
- The problem states that the bird perceives the velocity of the image as 1xcms.
- Since we have established that the perceived velocity is 0, we equate:
\(
\frac{1}{x} = 0 \Rightarrow x = 2
\)
Final Answer
The value of x is 2.
- The tank has a height of h filled with water (refractive index μ=43).
- The bird is hovering at a height of 3h from the bottom of the tank.
- The water level is falling at a rate of 1cms.
- The height of the water is h, and the bird is at 3h.
- The distance from the bird to the water surface is 3h−h=2h.
- The image of the bird will be formed below the water surface due to reflection from the silvered bottom.
- The distance of the image from the water surface can be calculated using the formula for the image distance in a spherical mirror:
\(
\frac{1}{f} = \frac{1}{v} + \frac{1}{u}
\)
Here, since the bottom is a plane mirror, the focal length f is infinite, and we can simplify the situation:
- The image distance v from the water surface is equal to the distance of the bird from the water surface, which is 2h.
- As the water level falls at 1cms, the distance x2 (the distance from the water surface to the bottom of the tank) is decreasing.
- Let x1 be the distance from the bird to the water surface, which is 2h initially but will change as the water level falls.
- The rate of change of the water level (dx2dt) is −1cms (negative because it is falling).
- The distance x1 will also change as the water level falls, so we can express the distance from the bird to the image as:
\(
d = x1 + x2
\)
- The rate of change of the distance d with respect to time can be expressed as:
\(
\frac{dd}{dt} = \frac{dx1}{dt} + \frac{dx2}{dt}
\)
- Since dx2dt=−1cms, we need to find dx1dt.
- The distance x1 is initially 2h, and as the water level falls, x1 increases. Thus:
\(
\frac{dx1}{dt} = 1 cm/s
\)
- Therefore, substituting into the equation:
\(
\frac{dd}{dt} = 1 - 1 = 0
\)
- The perceived velocity of the image by the bird is given by:
\(
\frac{d}{dt} \left( \frac{1}{x} \right) = \frac{1}{x^2} \cdot \frac{dd}{dt}
\)
- Since dddt is 0, the perceived velocity of the image is:
\(
\frac{d}{dt} \left( \frac{1}{x} \right) = 0
\)
- The problem states that the bird perceives the velocity of the image as 1xcms.
- Since we have established that the perceived velocity is 0, we equate:
\(
\frac{1}{x} = 0 \Rightarrow x = 2
\)
The value of x is 2.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - LECTURE SHEET (EXERCISE III LEVEL -I (MAIN) STRAIGHT OBJECTIVE TYPE QUESTIONS)7videos
GEOMETRICAL OPTICS
- BOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - LECTURE SHEET (EXERCISE III LEVEL -II (ADVAMCED) STRAIGHT OBJECTIVE TYPE QUESTIONS)12videos
GEOMETRICAL OPTICS
- BOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - LECTURE SHEET (EXERCISE II LEVEL -II (ADVANCED) MATRIX MATCHING TYPE QUESTIONS)1videos
GEOMETRICAL OPTICS
- BOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS10videos
ELEMENTS OF VECTORS
- BOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE106videos
LAWS OF MOTION
Similar Questions
The level of water in a tank is 5 m high . A hole of the area 10cm2 is made in the bottom of the tank . The rate of leakage of water from the hole is
View SolutionA tank is filled upto a height h with a liquid and is placed on a platform of height h from the ground. To get maximum range xm a small hole is punched at a distance of y from the free surface of the liquid. Then
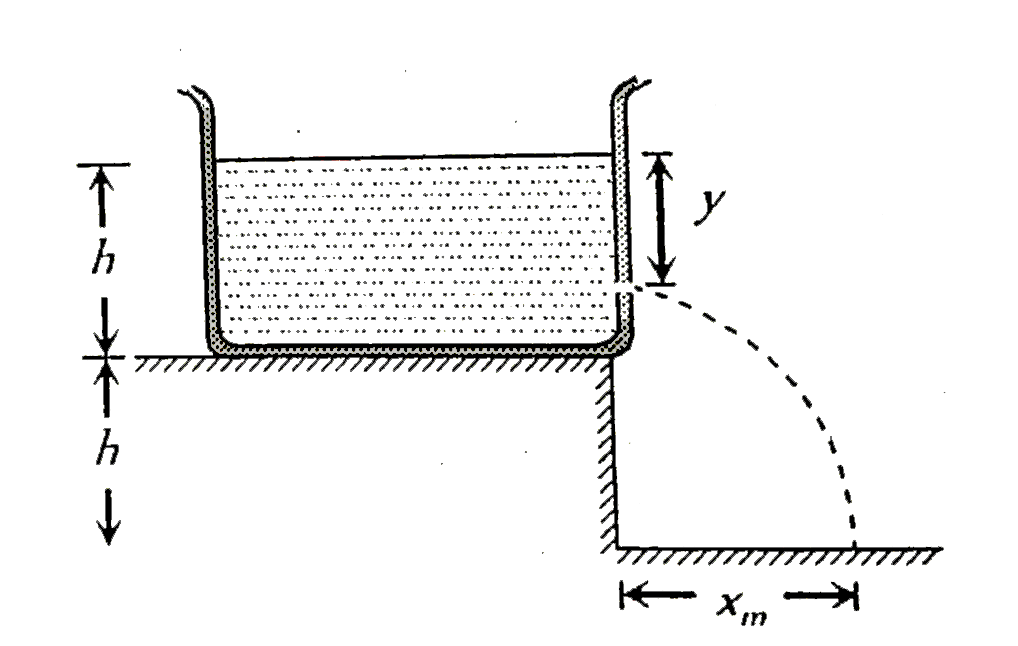 View Solution
View SolutionA rectangular tank is placed on a horizontal ground and is filled with water to a height H above the base. A small hole is made on one vertical side at a depth D below the level of the water in the tank. The distance x from the bottom of the tank at which the water jet from the tank will hit the ground is
View SolutionLiquid is filled in a container upto a height of H. A small hole is made at the bottom of the tank. Time taken to empty from H to H3 is t0. Find the time taken to empty tank from H3 to zero.
View SolutionA tank is filled up to a height 2H with a liquid and is placed on a platform of height H from the ground, the distance x from the ground where a small hole is punched to get the maximum range R is
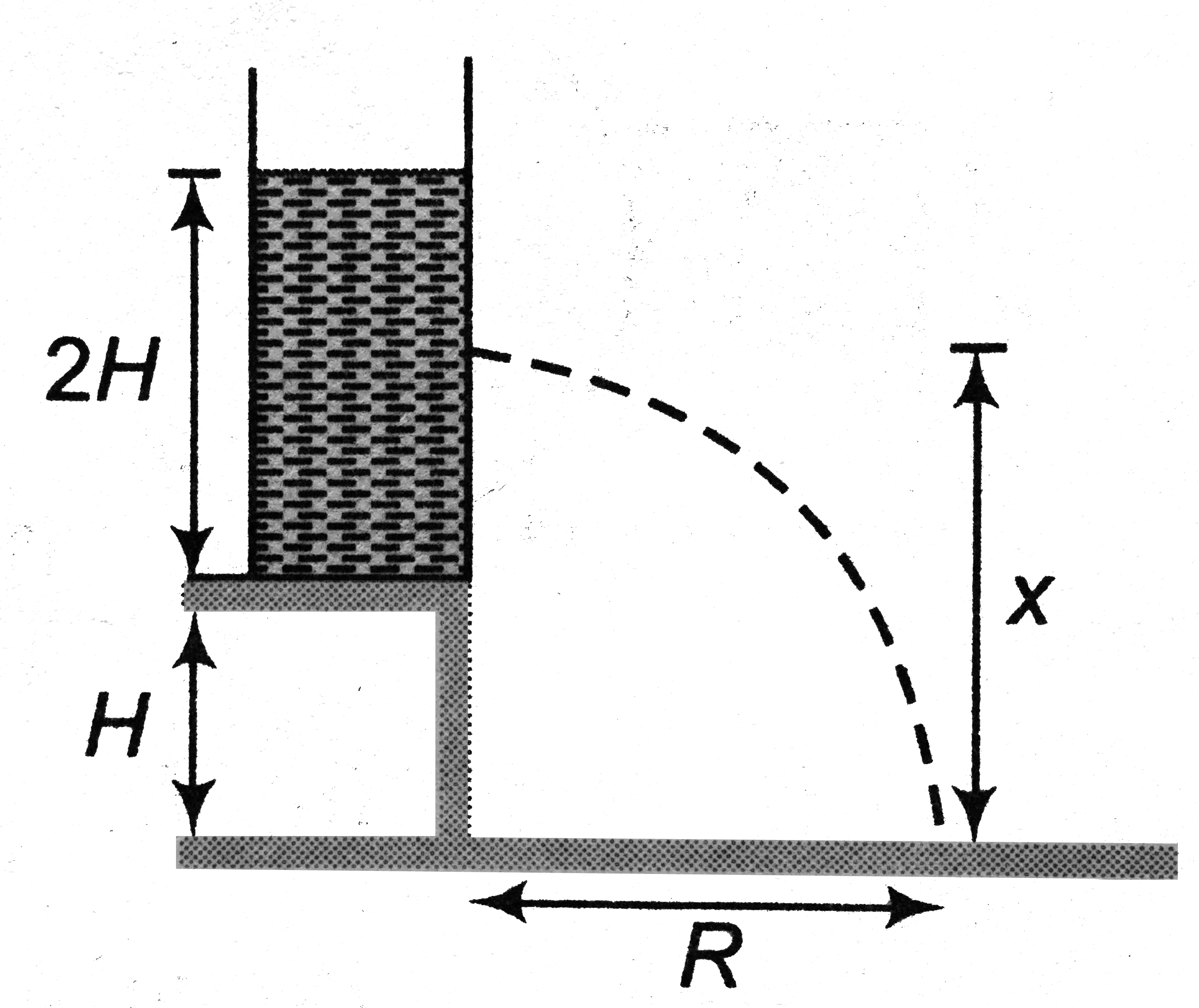 .View Solution
.View SolutionA tank is filled with a liquid upto a height H, A small hole is made at the bottom of this tank Let t1 be the time taken to empty first half of the tank and t2 time taken to empty rest half of the tank then find t1t2
View SolutionA tank is filled with a liquid upto a height H. A small hole is made at the bottom of this tank. Lett1 be the time taken to empty first half of the tank and t2 the time taken to empty rest half of the tank. Then find t1t2.
View SolutionA tank if filled with water upto height H. When a hole is made at a distance h below the level of water. What will be the horizontal range of water jet ?
View SolutionThere is a small hole near the botton of an open tank filled with liquid. The speed of the water ejected does not depend on
View SolutionA cylindrical tank of height H is open at the top end and it has a radius r. Water is filled in it up to a height of h. The time taken to empty the tank through a hole of radius r' in its bottom is
View Solution
