The focal length of plano-convex lens, the convex surface of which is silvered is $0.3 m$ , if $μ$ of the lens is $7 / 4$ , the radius of curvature of the convex surface is

$0.45 m$

$1.05 m$

$3 m$

$0.9 m$

Video Solution

Text Solution

Verified by Experts

(b) Here $P = 2 P_{L} + P_{M} = \frac{2}{f_{L}} - \frac{1}{f_{m}}$
$P = \frac{2}{f_{L}} + \frac{2}{R}$
Here $\frac{1}{f_{L}} = (μ - 1) (\frac{1}{r_{1}} - \frac{1}{r_{2}})) = (\frac{7}{4} - 1) (\frac{1}{\infty} + \frac{1}{R})$
$\frac{1}{f_{L}} = \frac{3}{4 R}$
$∴ P = - \frac{1}{F} = \frac{6}{4 R} + \frac{2}{R} + \frac{6 + 8}{4 R} = \frac{14}{4 R}$
or $- 10.3 = \frac{14}{4 R}$
$∴ R \frac{=_{14 \times 0.3}}{4} = - \frac{4.2}{4} = - 1.05$

Updated on:21/07/2023

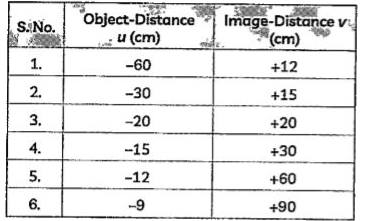
The focal length of the convex lens is
A $+ 10$ cm
B $+ 20 c m$
C $- 10 c m$
D $- 20 c m$
The focal length of a convex lens is 10 cm and its refractive index is 1.5. If the radius of curvature of one surface is 7.5 cm , the radius of curvature of the second surface will be
A7.5 cm
B15.0 cm
C75 cm
D5.0 cm
The focal length of a convex lens is 10 cm and its refractive index is 1.5 . If the radius of curvature of one surface is 7.5 cm, the radius of curvature of the second surface will be :
A $7.5 c m$
B $15 c m$
C $75 c m$
D $5 c m$