Question

The mass of hydrogen molecule is $3.32\times {10}^{-27}$ kg. If ${10}^{23}$ hydrogen molecules strikes per second at 2 $c{m}^2$ area of a rigid wall at an angle of ${45}^{\circ }$ from the normal and rebound back with a speed of 1000 $m{s}^{-1}$, then the pressure exerted on the wall is

Explanation

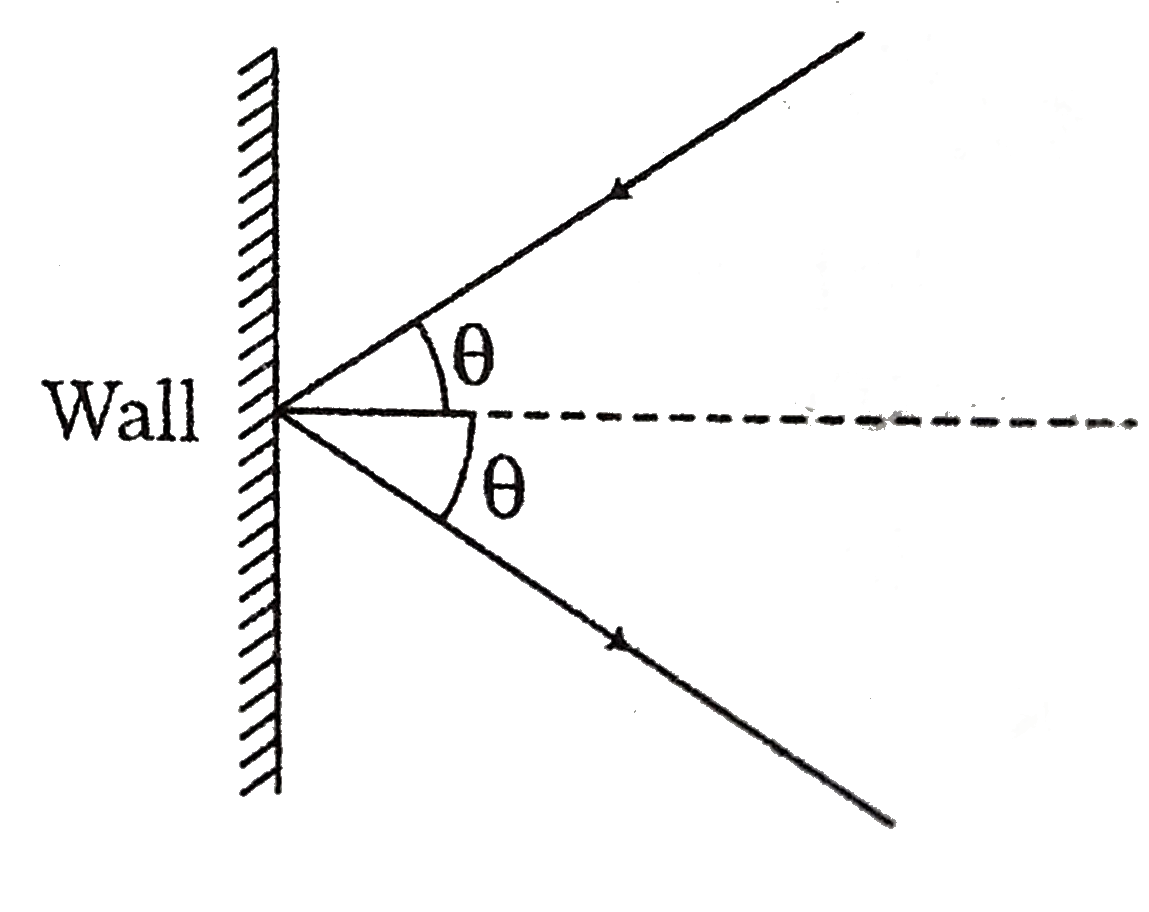
Velocity of each molecule along the normal `= v cos theta` where `theta` is the angle made by the velocity of the molecule with the normal.
Therefore, change in velocity
`= v cos theta - (- v cos theta ) = 2 v cos theta`
Change in momentum `= m xx 2v cos theta = 2 m v cos theta`
There, rate of change of momentum `= 2 m v cos theta xx N`
where N is the number, of molecules striking the wall each second.
Force exerted by molecules `= 2 m v N cos theta`
`Pressure = ("Force")/("Area")`
`= (2 m v N cos theta)/(A) = (2 xx 3.32 xx 10^(-27) xx cos 45^(@))/(2 xx 10^(-4))`
`= 2.35 xx 10^(3) N m^(-2)`