The minimum and maximum distances of a satellite from the center of the earth are 2R and 4R respectively, where R is the radius of earth and M is the mass of the earth . Find
(a) its minimum and maximum speeds,
(b) radius of curvature at the point of minimum distance.
√GM9R,√2GMR
√GM5R,√3GM2R
√GM6R,√2GM3R
√GM3R,√5GM2R
The correct Answer is:C
Applying the principle of conservation of angular momentum,
mv1(2R)=mv2(4R)
v1=2v2……i
From conservation of energy
12mv21−GMm2R=12mv22−GMm4R……….ii
Solving eqn i and ii we get
v2=√GM6R,v1=√2GM3R
If r is the radius of curvature at point A. 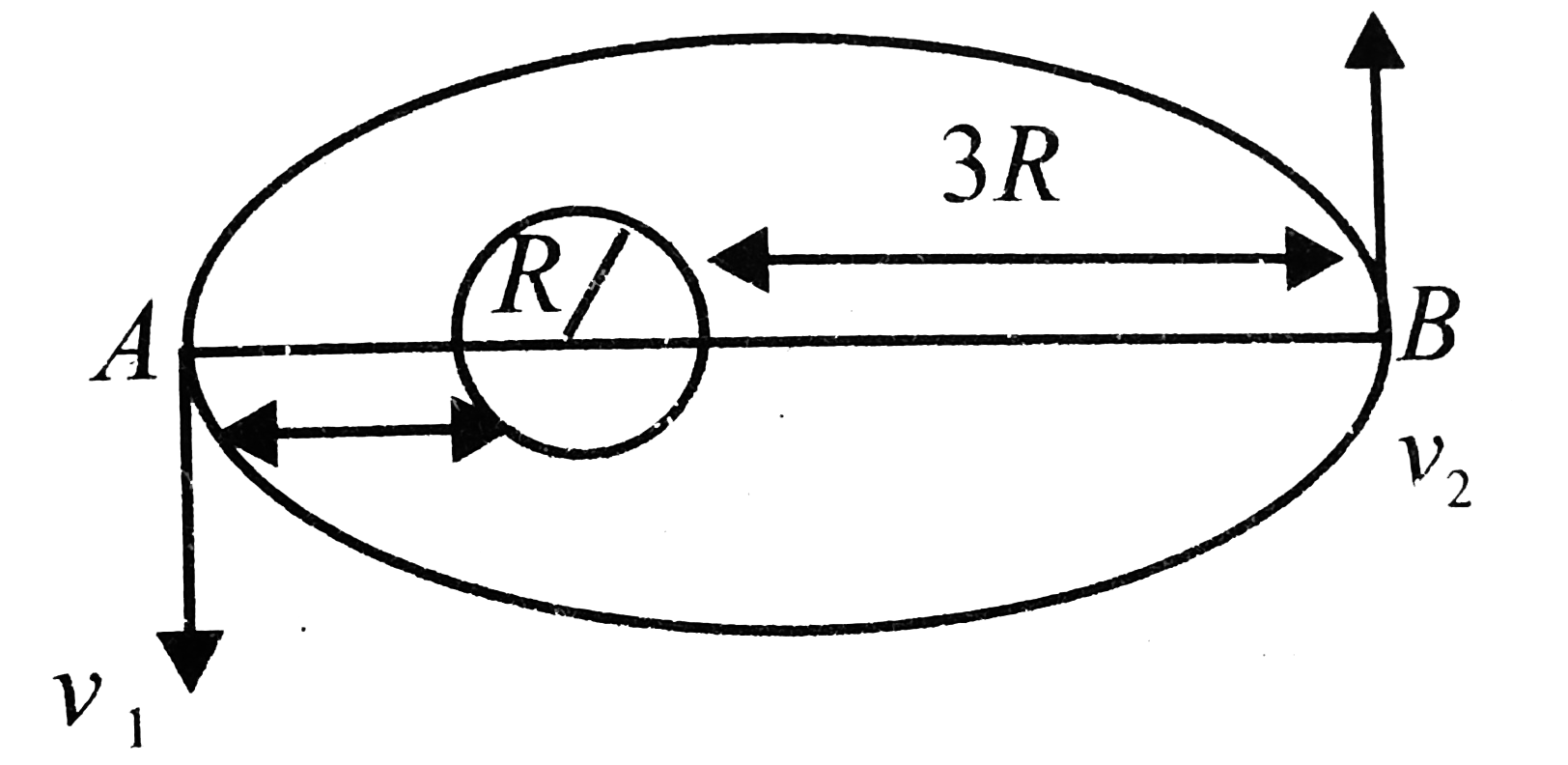
mv21r=GMm(2R)2
r=4v21GM=8R3 (putting value of v1)
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Linked Comprehension23videos
GRAVITATION
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Integer5videos
GRAVITATION
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Multiple Correct24videos
GRAVITATION
- BOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE1videos
FLUID MECHANICS
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer9videos
KINEMATICS-1
Similar Questions
The minimum and maximum distances of a satellite from the centre of the earth are 2R and 4R respectively where R is the radius of earth and M is the mass of the earth find radius of curvature at the point of minimum distance.
View SolutionThe minimum energy required to launch a m kg satellite from earth's surface in a circular orbit at an altitude of 5R, R is the radius of earth, will be
View Solution
Knowledge Check
- Question 1 - Select One
The distance of geostationary satellite from the centre of the earth (radius R) is nearest to
A5 RB6 RC7 RD8 R - Question 2 - Select One
The minimum energy required to launch a m kg satellite from earth’s surface in a circular orbit at an altitude of 2R, R is the radius of earth, will be
A35mgRB56mgRC2mgRD15mgR - Question 3 - Select One
Two identical satellites A and B are circulating round the earth at the height of R and 2 R respectively, (where R is radius of the earth). The ratio of kinetic energy of A to that of B is
A12B23C2D32
Two satellites of masses 50kg and 150kg revolve around the earth in circular orbits of radii 4R and 9Rrespectively where R is the radius of the earth. The speed of the two satellites will be in the ratio ?
View SolutionThe gravitational potential energy of a body at a distance r from the centre of the Earth is U where r>R (radius of Earth). What is the weight of body at the point ?
View SolutionTwo satellites are at distances of 2R and 4R respectively from the surface of earth. The ratio of their time periods will be :
View SolutionThe potential energy of a satellite of mass m revolving at height R above the surface of the earth where R= radius of earth is
View SolutionDistance of geostationary satellite from the surface of earth radius (Re=6400km) in terms of Re is
View Solution

