The normal body-temperature of a person is 97∘F . Calculate the rate at which heat is flowing out of his body through the clothes assuming the following values. Room temperature =47∘F , surface of the body under clothes =1.6m2 , conductivity of the cloth =0.04Js−1m−1C−1 , thickness of the cloth =0.5cm.
The correct Answer is:A, C
To solve the problem of calculating the rate at which heat is flowing out of a person's body through clothes, we will follow these steps:
Step 1: Convert Temperatures from Fahrenheit to Celsius
We need to convert the body temperature and room temperature from Fahrenheit to Celsius using the formula:
C=59(F−32)
- Body Temperature:
Cbody=59(97−32)=59(65)≈36.10∘C
- Room Temperature:
Croom=59(47−32)=59(15)≈8.33∘C
Step 2: Identify Given Values
We have the following values:
- Surface area of the body under clothes, A=1.6m2
- Thermal conductivity of the cloth, k=0.04Js⋅m⋅∘C
- Thickness of the cloth, d=0.5cm=0.005m
- Temperature difference, ΔT=Cbody−Croom=36.10−8.33=27.77∘C
Step 3: Apply the Formula for Heat Transfer
The rate of heat transfer (ΔQΔt) through conduction is given by Fourier's law:
ΔQΔt=k⋅A⋅ΔTd
Step 4: Substitute the Values into the Formula
Now we can substitute the known values into the formula:
ΔQΔt=0.04Js⋅m⋅∘C⋅1.6m2⋅27.77∘C0.005m
Step 5: Calculate the Rate of Heat Flow
Calculating the above expression:
ΔQΔt=0.04⋅1.6⋅27.770.005
=0.0443520.005=8.8704J/s≈356W
Final Answer
The rate at which heat is flowing out of the body through the clothes is approximately 356 Watts.
---
We need to convert the body temperature and room temperature from Fahrenheit to Celsius using the formula:
C=59(F−32)
Cbody=59(97−32)=59(65)≈36.10∘C
Croom=59(47−32)=59(15)≈8.33∘C
We have the following values:
- Surface area of the body under clothes, A=1.6m2
- Thermal conductivity of the cloth, k=0.04Js⋅m⋅∘C
- Thickness of the cloth, d=0.5cm=0.005m
- Temperature difference, ΔT=Cbody−Croom=36.10−8.33=27.77∘C
The rate of heat transfer (ΔQΔt) through conduction is given by Fourier's law:
ΔQΔt=k⋅A⋅ΔTd
Now we can substitute the known values into the formula:
ΔQΔt=0.04Js⋅m⋅∘C⋅1.6m2⋅27.77∘C0.005m
Calculating the above expression:
ΔQΔt=0.04⋅1.6⋅27.770.005
=0.0443520.005=8.8704J/s≈356W
The rate at which heat is flowing out of the body through the clothes is approximately 356 Watts.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - QUESTIONS FOR SHORT ANSWER11videos
HEAT TRANSFER
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - OBJECTIVE II6videos
HEAT TRANSFER
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT AND TEMPERATUREEXERCISE - Objective 26videos
HEAT AND TEMPERATURE
- BOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Question for short Answer4videos
INTRODUCTION TO PHYSICS
Similar Questions
The glass windows of a room have a total area of 5m2 and glass thickness is 3mm. Calculate the rate at which heat escapes from the room per minute by conduction when the inside of the windows is at a temperature 15∘C and the outside temperature is −10∘C. Thermal conductivity =0.84Wm−1K−1.
View SolutionSteam at 120∘C is continuously passed through a 50−cm long rubber tube of inner and outer radii 1.0cm and 1.2cm . The room temperature is 30∘C . Calculate the rate of heat flow through the walls of the tube. Thermal conductivity of rubber =0.15Js−1m−1C−1 .
View Solution
Knowledge Check
- Question 1 - Select One
A cubical ice box of side 50 cm has a thickness of 5.0 cm. if 5 kg of ice is put in the box, estimate the amount of ice remaining after 4 hours. The outside temperature is 40∘C and coefficient of thermal conductivity of the material of the box = 0.01Js−1m−1.∘C−1. Heat of fusion of ice=335Jg−1.
A3.7kgB3.9kgC4.7kgD4.9kg
A pitcher with 1−mm thick porous walls contain 10kg of water. ,Water comes to its outer surface and evaporates at the rate of 0.1gs−1 . The surface area of the pitcher (one side) =200cm2 . The room temperature =42∘C , latent heat of vaporization =2.27×106Jkg−1 , and the thermal conductivity of the porous walls =0.80js−1m−1C−1 . Calculate the temperature of water in the pitcher when it attains a constant value.
View SolutionCalculate the time in which a layer of ice 6 cm thick, on the surface of a pond will increase in thickness by 2 mm. Temperature of the surrounding air =−20∘C,L=333KJ kg−1 Conductivity of ice =0.08cal s−1cm−1∘C−1..
View SolutionA metal rod of cross sectional area 1.0cm2 is being heated at one end. At one time , the temperature gradient is 5.0∘Ccm−1 at cross section A and is 2.5∘Ccm−1 at cross section B. calculate the rate at which the temperature is increasing in the part AB of the rod. The heat capacity of the part AB=0.40J∘C−1 , thermal conductivity of the material of the rod. K=200Wm−1C−1 . Neglect any loss of heat to the atmosphere.
View Solution1.56×105J of heat is conducted through is 2m2 wall of 12 cm thick in one hour. Temperature difference between the two sides of the wall is 20∘C. The thermal conductivity of the material of the wall is (in Wm−1K−1)
View SolutionA room has s window fitted with a single 1.0m×2.0m glass of thickness 2mm. (a) Calculate the rate of heat flow through the closed window when the temperature inside the room is 32∘C and that outside is 40∘C . (b) The glass is now replaced by two glasspanes, each having a thickness of 1mm and separated by a distance of 1mm. Calculate the rate of heat flow under the same condition of temperature. Thermal conductivity of window glass =1.0Js−1m−1C−1 and that of air =0.025Js−1m−1C−1 .
View SolutionWater flows at a speed of 6 cms−1 through a tube of radius 1 cm. coefficient of viscosity of water at room temperature is 0.01 poise. Calculate the Reynolds number. Is it a steady flow.
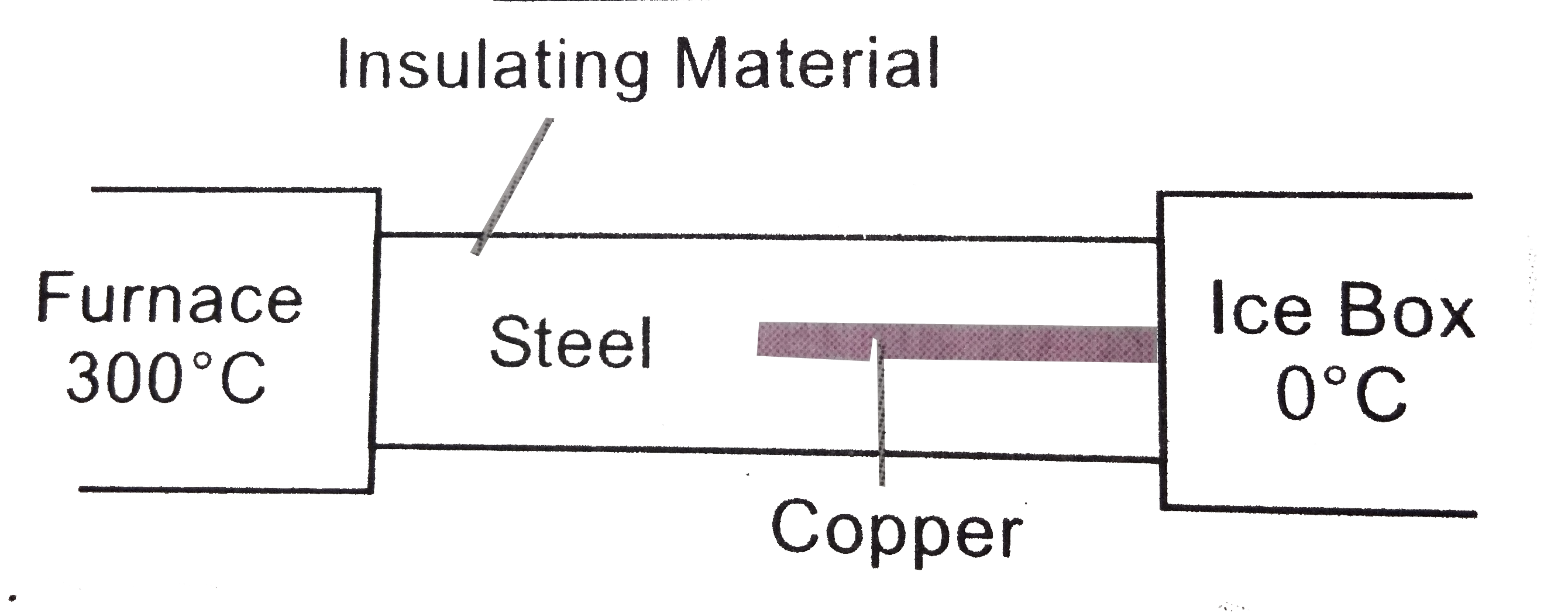
View SolutionWhat is the temperature of the steel-copper junction in the steady state system show in Fig. 7(e).17? Length of steel rod = 15.0 cm, length of the copper rod = 10.0 cm, temperature of the furnace =300∘C, temperature of other end 0∘C. The are of cross-section of the steel rod is twice that of the copper rod. (Thermal conductivity of steel =50.2js−1m−1K−1 and of copper =3895js−1m−1K−1).
 View Solution
View Solution

