The physics teacher gave a rod of length 'L' units to Roy and the teacher asked Roy to find the shift in the centre of gravity when 1/4 of the total length of the rod is removed. What will be the Roy's answer in terms of L?
Original length of rod = L units
CG = L2 units
New length of rod = L−L4=3L4
New position of CG = 3L8
Shift in CG= L2−3L8=4L−3L8=L8 units
Topper's Solved these Questions
- DYNAMICSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - DYNAMICSEXERCISE - CONCEPT APPLICATION(LEVEL 2)
19 Videos
- CHEMICAL EFFECTS OF ELECTRIC CURRENTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - CHEMICAL EFFECTS OF ELECTRIC CURRENTEXERCISE - COMPETITION CORNER (MCQ)
48 Videos
- ELECTRICITYBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - ELECTRICITYEXERCISE - CONCEPT APPLICATION (Level 3)
10 Videos
Similar Questions
Knowledge Check
A thin rod of length L is bent to form a semi circle. The mass of the rod is M. What will be the gravitational potential at the centre of the circle?
A−GMLB−GM2πLC−πGM2LD−πGMLA thin of length L is bent to form a semicircle. The mass of rod is M. What will be the gravitational potential at the centre of the circle ?
A−GMLB−GM2πLC−πGM2LD−πGMLA uniform rod of length L pivoted at the bottom of a pond of depth L/2 stays in stable equilibrium as shown in the figure.

Find the force acting at the pivoted end of the rod in terms of mass m of the rod.A(√2−1)mgB(√2+1)mgC(√3+1)mgD(√2+3)mg
A straight rod of length L has one of its end at the origin and the other at X=L. If the mass per unit length of the rod is given by Ax where A is constant, where is its centre of mass?
View SolutionThe density of a thin rod of length l varies with the distance x from one end as ρ=ρ0x2l2. Find the position of centre of mass of rod.
View SolutionA slender homogeneous rod of length 2L floats partly immersed in water, being supported by a string fastened to one of its ends, as shown in the figure. The specific gravity of the rod is 0.36. The length of the rod that extends out of the water is KL10 Find the value of K.
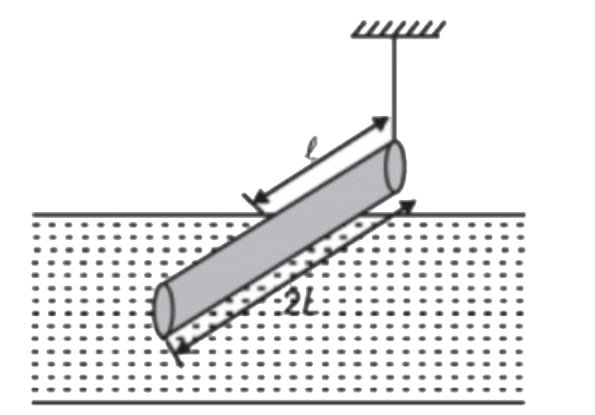
View SolutionIn example 1.49 find the velocity of the mid point of the rod in terms of its length l, v and θ.
View SolutionA rigid rod of mass m & length l is pivoted at one of its ends. If it is released from its horizontal position, find the speed of the centre of mass of the rod when it becomes vertical.
View Solution

 .
.