The ratio of velocity of light in first medium to that of velocity of light in second medium is _____
To solve the question regarding the ratio of the velocity of light in the first medium to that in the second medium, we can follow these steps:
1. Understand the Concept of Refractive Index:
The refractive index (n) of a medium is defined as the ratio of the speed of light in vacuum (c) to the speed of light in that medium (v). Mathematically, it is expressed as:
n=cv
2. Express the Speed of Light in Each Medium:
From the definition of refractive index, we can express the speed of light in a medium in terms of the speed of light in vacuum and the refractive index:
v1=cn1(for the first medium)
v2=cn2(for the second medium)
3. Set Up the Ratio of Velocities:
We want to find the ratio of the velocity of light in the first medium (v1) to the velocity of light in the second medium (v2):
v1v2=cn1cn2
4. Simplify the Ratio:
The speed of light in vacuum (c) cancels out in the ratio:
v1v2=n2n1
5. Conclusion:
Therefore, the ratio of the velocity of light in the first medium to that in the second medium is equal to the refractive index of the second medium with respect to the first medium:
v1v2=n2n1
Final Answer:
The ratio of the velocity of light in the first medium to that in the second medium is equal to the refractive index of the second medium with respect to the first medium.
---
The refractive index (n) of a medium is defined as the ratio of the speed of light in vacuum (c) to the speed of light in that medium (v). Mathematically, it is expressed as:
n=cv
From the definition of refractive index, we can express the speed of light in a medium in terms of the speed of light in vacuum and the refractive index:
v1=cn1(for the first medium)
v2=cn2(for the second medium)
We want to find the ratio of the velocity of light in the first medium (v1) to the velocity of light in the second medium (v2):
v1v2=cn1cn2
The speed of light in vacuum (c) cancels out in the ratio:
v1v2=n2n1
Therefore, the ratio of the velocity of light in the first medium to that in the second medium is equal to the refractive index of the second medium with respect to the first medium:
v1v2=n2n1
The ratio of the velocity of light in the first medium to that in the second medium is equal to the refractive index of the second medium with respect to the first medium.
Topper's Solved these Questions
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - Concept Application (Level 1) Matching
1 Videos
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - Concept Application (Level 1)
30 Videos
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - Concept Application (Level 1) True or False
7 Videos
- KINEMATICS BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - KINEMATICS EXERCISE - CONCEPT APPLICATION (Level 3) (Application Based Questions )
5 Videos
- MAGNETISM BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MAGNETISM EXERCISE - CONCEPT APPLICATION (Level 3)
4 Videos
Similar Questions
The velocity of light in first medium is 2.8×108m s−1 and that in the second medium is 2.3×108m−1. Find the refractive index of medium 2 with respect to medium 1.
View SolutionThe velocity of light in a stationary medium is u =c/n where c is the velocity of light in vacuum and n is the refractive index of the material (see 63.1). Find the velocity of light in the medium moving at a constant speed relative to the source of light.
View Solution
Knowledge Check
A ray of light is incident on a medium with angle of incidence I and refracted into a second medium with angle of refraction r. The graph of sin(i)vssin(R) is as shown in figure. Then the velocity of light in the first medium in n times the velocity of light in the second medium. What shoul be th evalue of n?
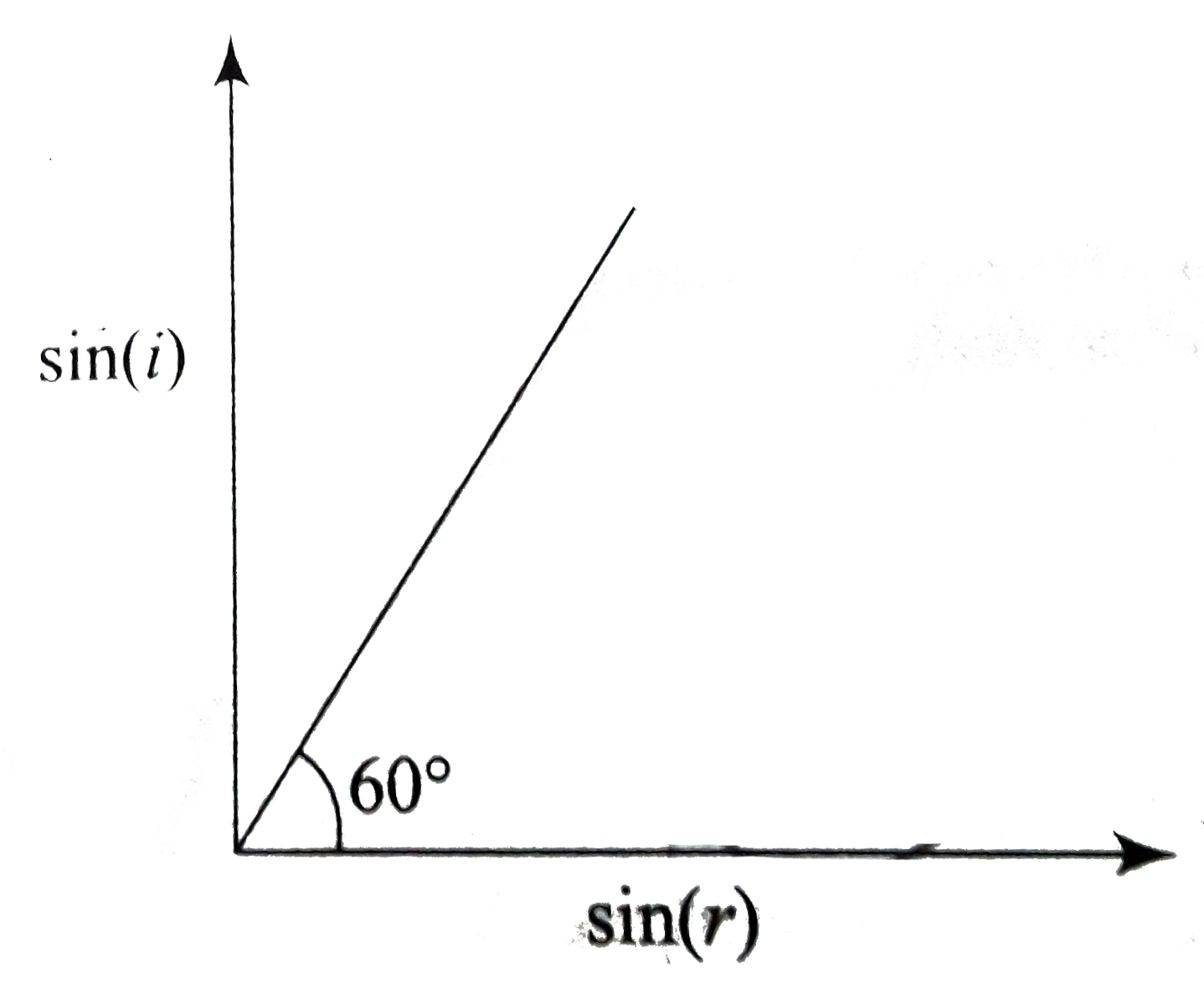 A√3B1/√3C√3/2D2/√3
A√3B1/√3C√3/2D2/√3When light is incident on a medium at angle i and refracted into a second medium at an angle r, the graph of sinr versus sini is as shown. From this one can conclude that

(i) the velocity of light in second medium is √3 times the velocity of light in the first medium
(ii) the velocity of light in the first medium is √3 times the velocity of ligth in second medium
(iii) the critical angle of the two media is given by siniC=1√3
(iv) the critical anlge of the two media is given by siniC=1√2A(i), (ii)B(ii), (iii)C(i), (iii)D(i), (iv)The velocity of light was first measured by
AEinsteinBNewtonCRoemerDGalileo
Which of the following phenomena support the wave theory of light?
1. Scattering
2.Interference
3. Diffraction
4. Velocity of light in a denser medium is less than the velocity of light in the rare medium
View SolutionThe speed of light in the medium is
View SolutionThe velocity of light in vacuum is 3×108m/s. Therefore the velocity of light in m/s in a medium of R.I. 1.25 is
View SolutionIf the refractive index of a medium is 1.7, the velocity of light in that medium is
View SolutionAccording to wave theory of light, velocity of light in rarer medium is
View Solution
