The resonant frequency of a circuit is f. If the capacitance is made 4 times the initial values, then the resonant frequecy will become
f/2
2f
f
f/4
The correct Answer is:A
To solve the problem, we need to understand the relationship between capacitance and resonant frequency in an LC circuit. The resonant frequency f is given by the formula:
f=12π√LC
where:
- L is the inductance,
- C is the capacitance.
1. Identify the Initial Resonant Frequency:
The initial resonant frequency is given as f.
2. Understand the Effect of Changing Capacitance:
We are told that the capacitance C is increased to 4C (i.e., it becomes 4 times its initial value).
3. Substitute the New Capacitance into the Resonant Frequency Formula:
The new resonant frequency f′ can be expressed as:
f′=12π√L⋅4C=12π√4LC=12π⋅2√LC=12⋅12π√LC
4. Relate the New Frequency to the Initial Frequency:
Since 12π√LC=f, we can substitute this into our equation:
f′=12f
5. Conclusion:
Therefore, when the capacitance is increased to 4 times its original value, the new resonant frequency f′ becomes:
f′=f2
Final Answer:
The new resonant frequency will be f2.
- L is the inductance,
- C is the capacitance.
The initial resonant frequency is given as f.
We are told that the capacitance C is increased to 4C (i.e., it becomes 4 times its initial value).
The new resonant frequency f′ can be expressed as:
f′=12π√L⋅4C=12π√4LC=12π⋅2√LC=12⋅12π√LC
Since 12π√LC=f, we can substitute this into our equation:
f′=12f
Therefore, when the capacitance is increased to 4 times its original value, the new resonant frequency f′ becomes:
f′=f2
The new resonant frequency will be f2.
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - A2ZCHAPTER - ALTERNATING CURRENTEXERCISE - Power In Ac Circuits
21 Videos
- ALTERNATING CURRENTBOOK - A2ZCHAPTER - ALTERNATING CURRENTEXERCISE - Problems Based On Mixed Concepts
40 Videos
- ALTERNATING CURRENTBOOK - A2ZCHAPTER - ALTERNATING CURRENTEXERCISE - Connected With Ac
42 Videos
- ATOMIC PHYSICSBOOK - A2ZCHAPTER - ATOMIC PHYSICSEXERCISE - Section D - Chapter End Test
30 Videos
Similar Questions
Knowledge Check
The resonant frequency of an L - C circuit is
A12π√LCB12π√LCC12π√LCD12π√CLIn the circuit shown in fig, the resonant frequency is
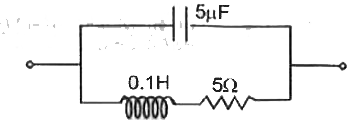 A75kcsB750kcsC7.5kcsD75mcs
A75kcsB750kcsC7.5kcsD75mcsThe resonant frequency of a circuit is 100 Hz. If the capacitor is replaced by another capacitor of capacity = 4 times the original capacity, then the resonant frequency will be
A25 HzB100 HzC50 HzD75 Hz
Reduction of resonant frequency in LCR circuit with a generator is done by:
View SolutionIn an LCR series circuit the capacitance is changed from C to 4C For the same resonant fequency the inductance should be changed from L to .
View SolutionIn a series resonant L-C-R circuit, the capacitance is changed from C to 3C. For the same resonant frequency, the inductance should be changed from L to
View SolutionIn series L−C−R resonant circuit, to increase the resonant frequency
View SolutionIn a series LCR circuit, at resonant frequency.
View Solution
