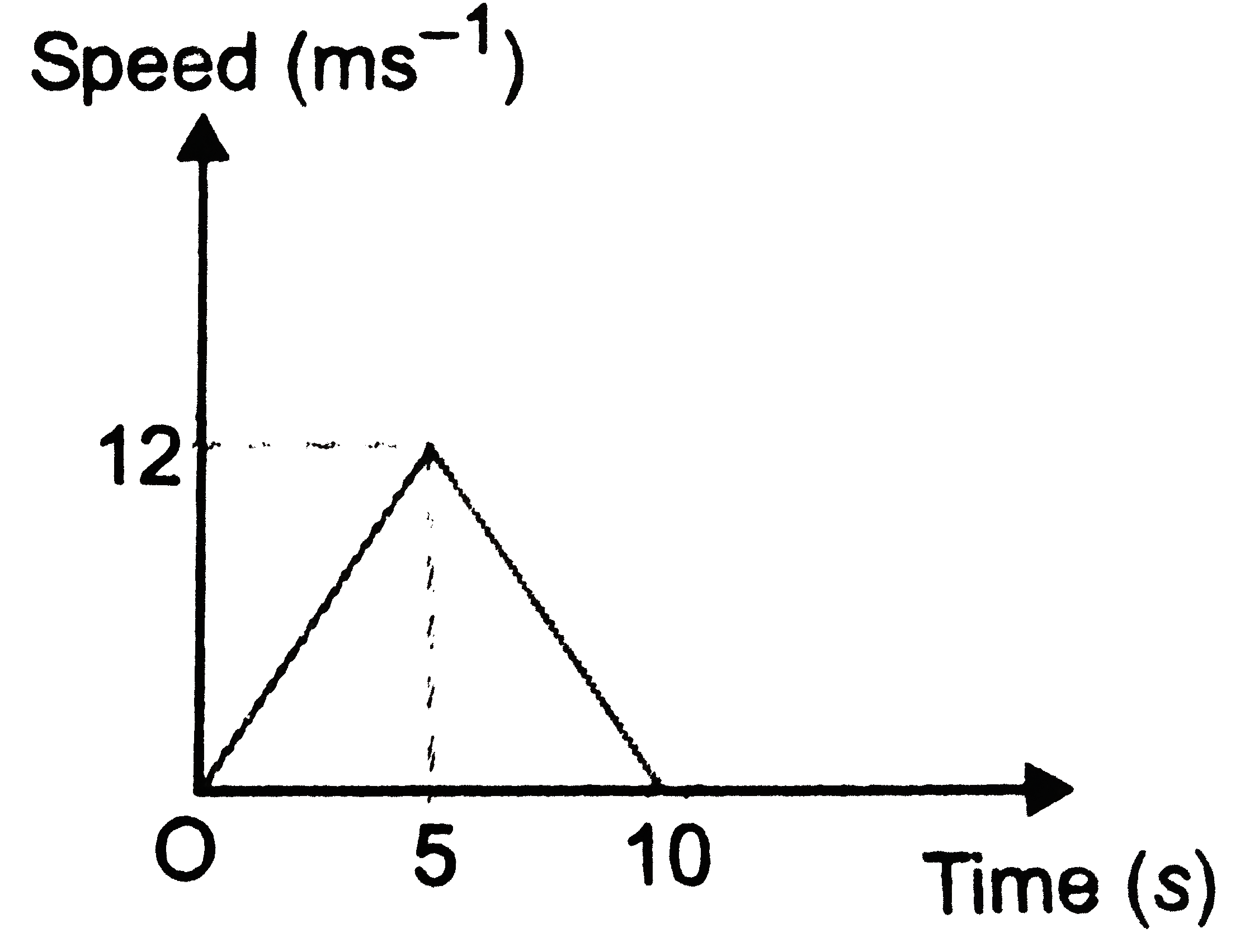
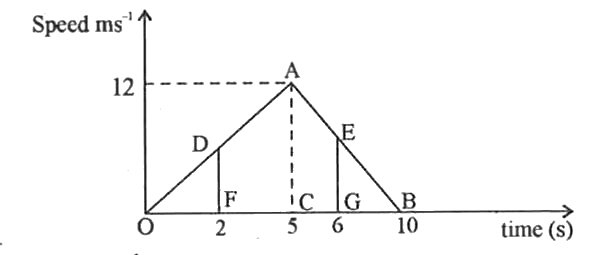
The speed-time graph of a particle moving along a fixed direction is shown in figure. Obtain the distance traversed by the particle between (a)t=0s to 10s.(b)t=2s to 6s.
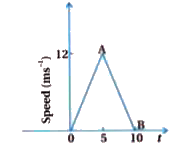
What is the average speed of the particle over the intervals in (a) and (b) ? Solution in Gujrati
The distance travelled = Area under the v→tgraph.
For the first case,
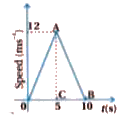
Area = Area of ΔOAB=12×10×2=60m
Constant acceleratioon during
time 0 s to 5 s = slope of the graph OA
∴a=125=2.4ms−2
Now velocity of a particle after t = 2 s is
v(2)=v0+at=0+2.4×2=4.8ms−1
For the distance travels between time t= 2 s to t = 5 s,
d1=v(2)×(5−2)+12a(5−2)2
=(4.8×3)+12×2.4×(3)2=14.4+1.2×9=14.4+10.8
∴d1=25.2m ...(1)
Now, during motion on AB path, constant retardation a.= slope of the line AB
∴a.=12−5=−2.4ms−2
Now time interval t.=6sec−5sec=1sec
d2=v0t+12a.t. when v0=12ms−1
d2=(12×1)+12(−2.4)×1
=12−1.2=10.8m
If total distance travels by a particle during t=2 s to t = 6 s
∴d=d1+d2
=25.2+10.8
∴d=36.0m
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - SECTION-C43videos
MOTION IN A STRAIGHT LINE
- BOOK - KUMAR PRAKASHANCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - SECTION-D26videos
MOTION IN A STRAIGHT LINE
- BOOK - KUMAR PRAKASHANCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - SECTION-A TRY YOUR SELF (VSQs)52videos
MOTION IN A STRAIGHT LINE
- BOOK - KUMAR PRAKASHANCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - QUESTION PAPER (SECTION -C)3videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - KUMAR PRAKASHANCHAPTER - OBJECTIVE QUESTIONS AS PER NEW PAPER STYLE EXERCISE - CHAPTER - 8 (Match Type questions)5videos
OBJECTIVE QUESTIONS AS PER NEW PAPER STYLE
Similar Questions
What is acceleration ? What is the direction ? Also gives its unit.
05:59View SolutionAmong the four graph shown in the figure there is only one graph for which average velocity over the time interval (O,T) can vanish for a suitably chosen T. Which one is it ?
04:56View SolutionHow can we determine whether the object is in rest or not?
02:43View SolutionGlycerine flows steadily through a horizontal tube of length 1.5m and radius 1.0 cm . If the amount of glycerine collected per second at one is 4.0×10−3kgs−1 , what is the pressure difference between the two ends of the tube ? (Density of glycerine =1.3×10103kgm−3 and viscosity of glycerine =0.83 Pa s). [ you may also like to check if the assumption of laminar flow in the tube is correct]
12:50View SolutionType of satellites are given in Column-I and their uses are given in Column-II. Match them properly :
Column-IColumn-II(1) Polar satellite(a) Tele communication(2) Geo-stationary satellite(b) Investigation(c) For information about atmosphere11:58View SolutionVelocity-time graph of a particle moving in a straight line is shown in figure. In the time interval from t=0 to t=14s, find
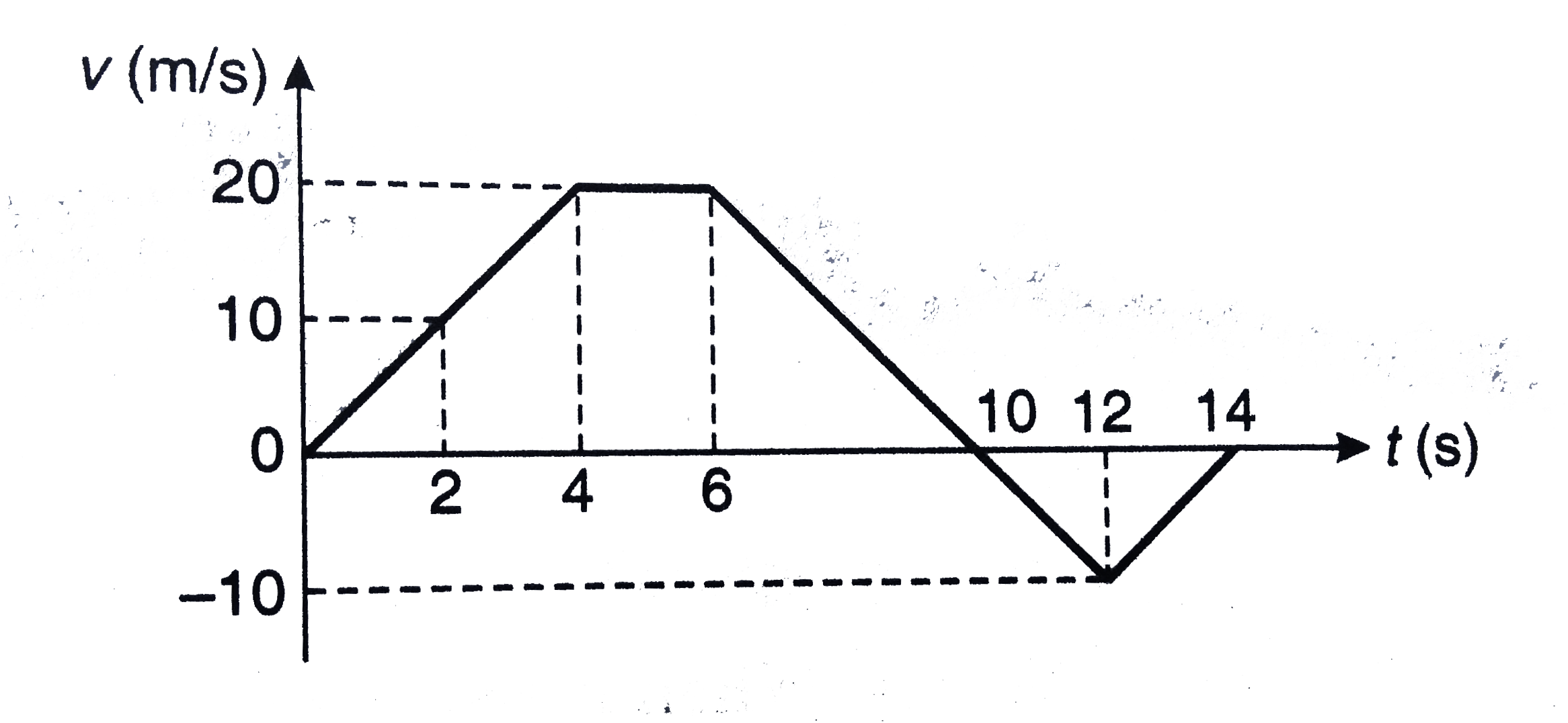
(a) average velocity and
(b) average speed of the particle.09:01View SolutionThe speed-time graph of a particle moving along a fixed direction is shown in the Fig. 2 (CF) . 15. The distance traversed by the particle between t=2s 6s is .
 .04:00View Solution
.04:00View SolutionThe speed-time graph of a particle moving along a fixed direction as shown in the figure. The distance transversed by the particle between t = 0 s to t = 10 s is
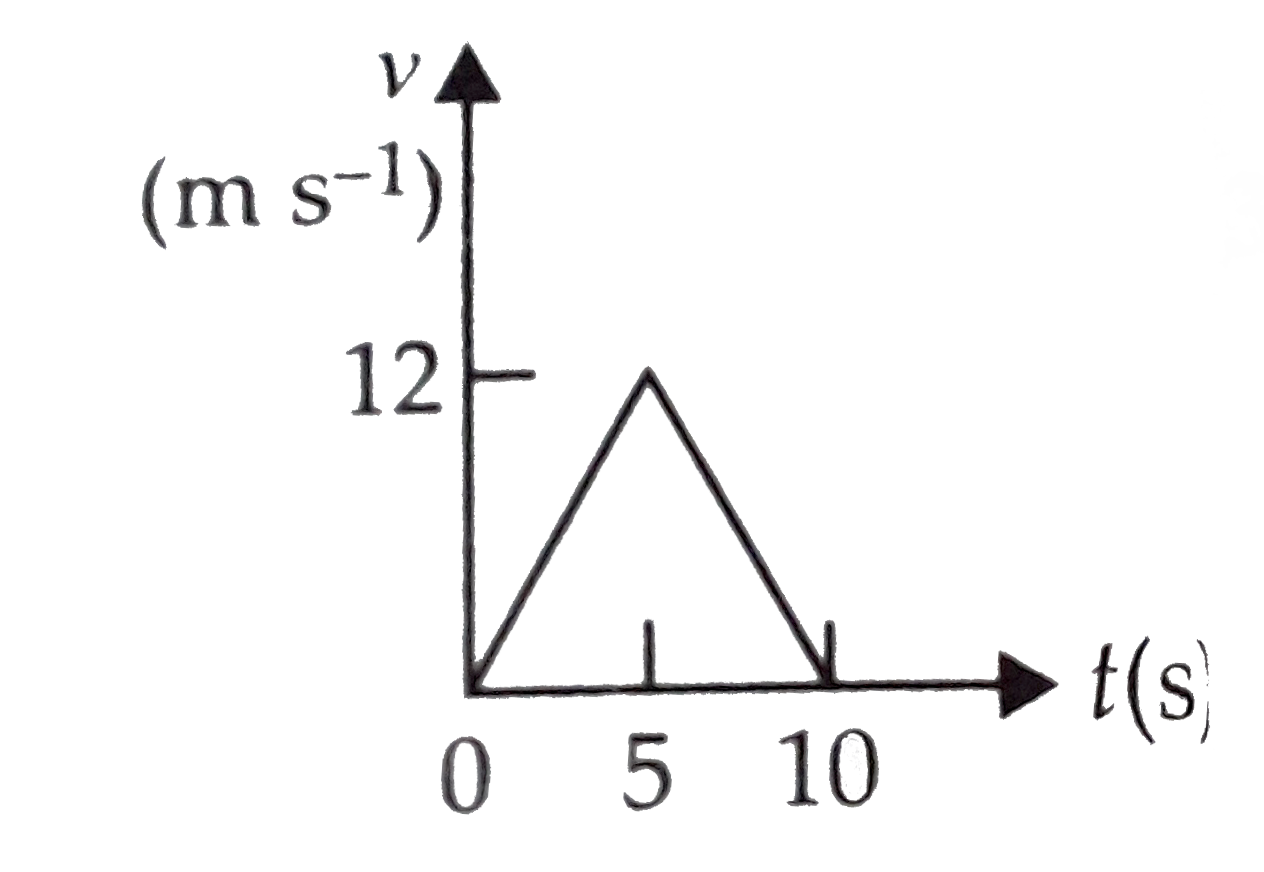 01:43View Solution
01:43View Solutionकिसी निश्चित दिशा के अनुदिश चल रहे किसी कण का चाल-समय ग्राफ चित्र 3.28 में दिखाया गया है | कण द्वारा (a) t=0 s से t=10 s, (b) t=2 s से 6s के बीच तय की गई दूरी ज्ञात कीजिए |
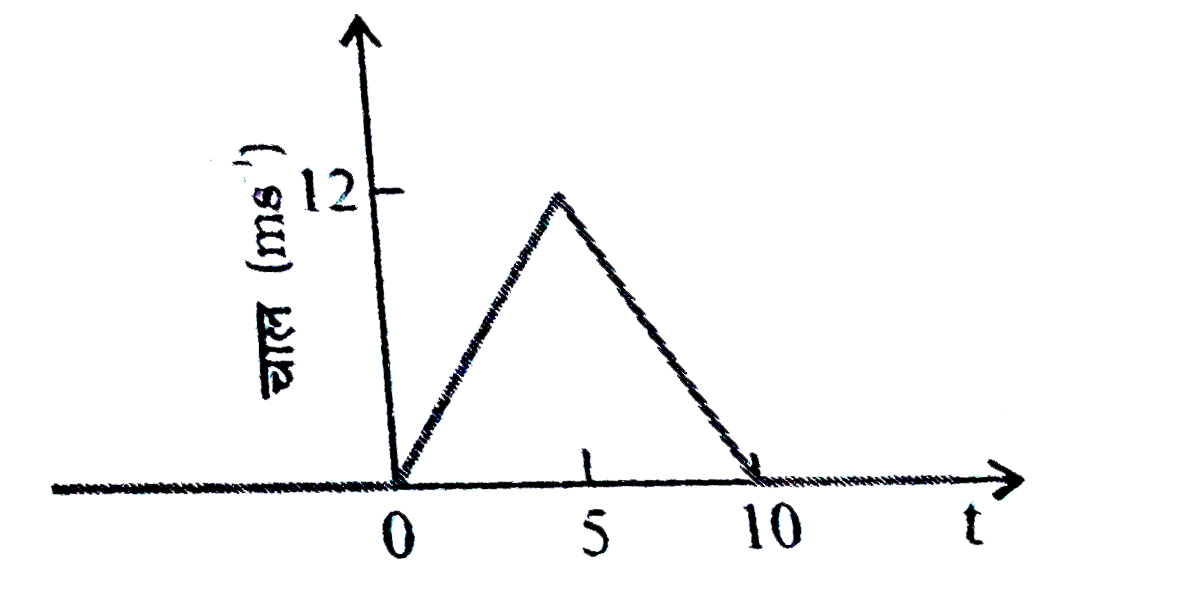
(a) तथा (b) में दिए गए अंतरालों की अवधि में कण की औसत चाल क्या है ?06:20View Solutionकिसी निश्चित दिशा में अनुदिश चल रहे किसी कण का चाल-समय ग्राफ चित्र में दिखाया गया है । कण द्वारा (a) t=0s से t=10s, (b) t=2s से 6s के बीच तय की गई दूरी ज्ञात कीजिए । (c ) (a) तथा (b) में दिए गए अन्तरालों की अवधि में कण की औसत चाल क्या है ?
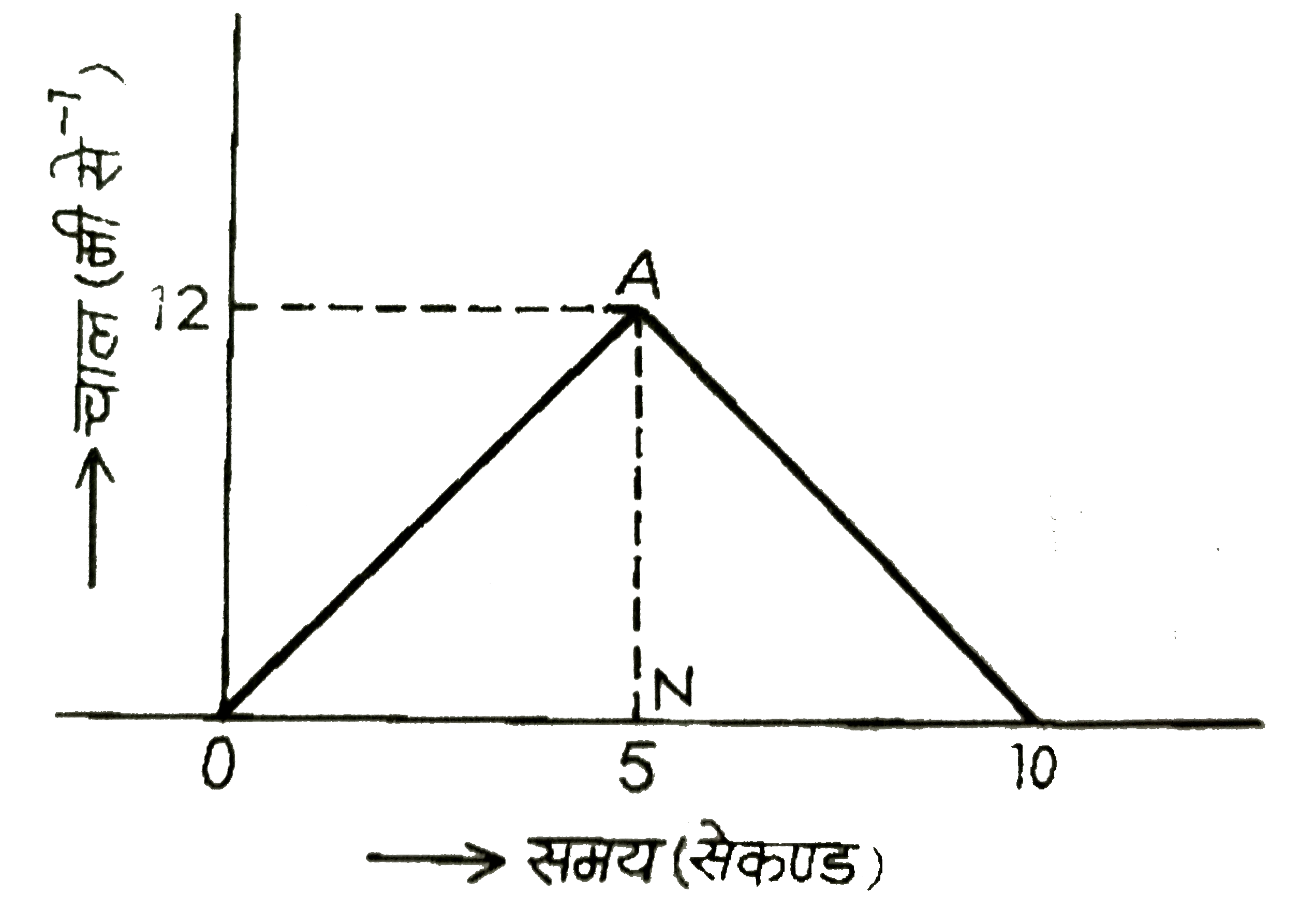 06:06View Solution
06:06View Solutionकिसी निश्चित दिशा में अनुदिश चल रहे किसी कण का चाल - समय ग्राफ चित्र 3.60 में दिखाया गया है । कण द्वारा
(a) t=0 सेकण्ड से t=10 सेकण्ड,
(b) t=2 सेकण्ड से 6 सेकण्ड के बीच तय की गई दूरी ज्ञात कीजिए |
(a) तथा (b) में दिए गए अंतरालों की अवधि में कण की औसत चाल क्या है ?
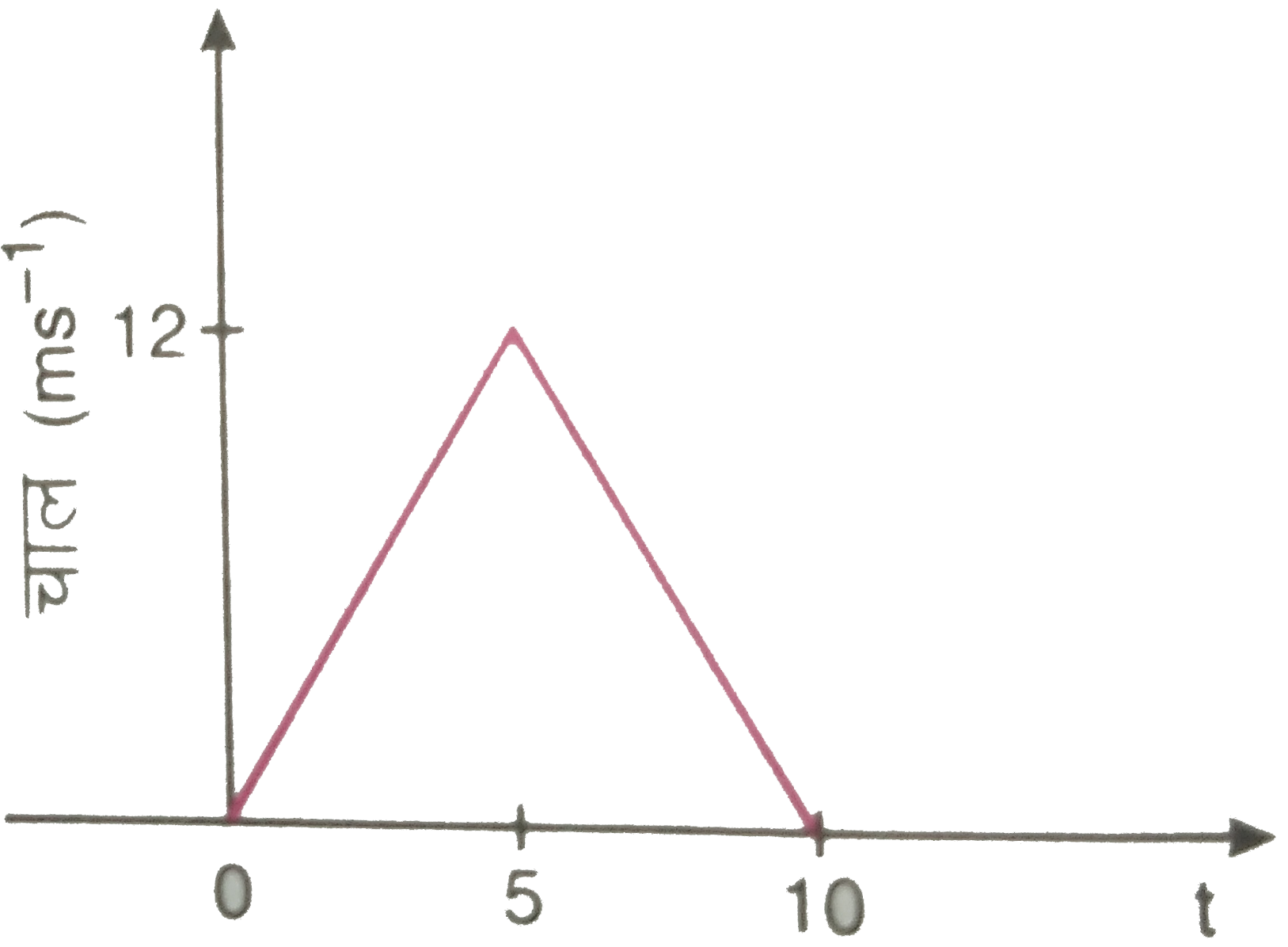 View Solution
View SolutionThe speed time graph of a particle moving along a fixed direction is as shown in the figure. Obtain the distance travelled by the particle from,
(a) t = 0 to 10s
(b) t= 2s to 6s
(c) What is the average speed of the particle over the intervals in (a) and (b)?
 02:05View Solution
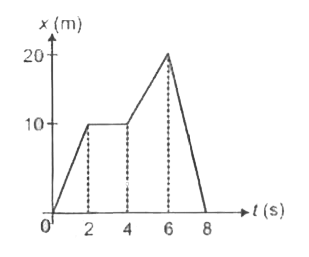
02:05View SolutionThe position (x) - time (t) graph for a particle moving along a straight line is shown in figure. The average speed of particle in time interval t = 0 to t = 8 s is
 02:25View Solution
02:25View SolutionThe speed-time graph of a particle moving along a fixed direction is shown in figure. Obtain the distance traversed by the particle between (a)t=0s to 10s(b)t=2s to 6s What is the average speed of the particle over the intervals in a and b?
01:52View SolutionThe speed-time graph of a particle moving along a fixed direction is shown in Fig. Obtain the distance traversed by the particle between (a) t = 0 s to 10 s, (b) t = 2s to 6s.
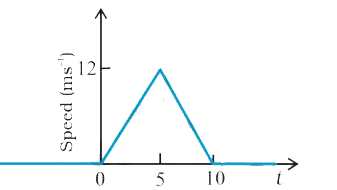
What is the average speed of the particle over the intervals in (a) and (b) ?04:13View Solution

