Two identical copper spheres are separated by 1m in vacuum. How many electrons would have to be removed from one sphere and added to the other so that they now attract each other with a force of 0.9N?
6.25×1015
6.25×1015
6.25×1013
0.65×1013
The correct Answer is:C
To solve the problem, we need to find out how many electrons must be transferred between two identical copper spheres so that they attract each other with a force of 0.9 N when separated by 1 meter in vacuum.
1. Understand the Problem:
We have two identical copper spheres, and we need to calculate how many electrons need to be transferred to create a force of attraction of 0.9 N between them.
2. Use Coulomb's Law:
The force F between two charges q1 and q2 separated by a distance r is given by Coulomb's law:
F=kq1q2r2
where k is Coulomb's constant, approximately 9×109N m2/C2.
3. Set Up the Equation:
Since we are transferring n electrons, the charge of one electron is e=1.6×10−19C. If we remove n electrons from one sphere, it gains a charge of +ne, and the other sphere, which gains those electrons, has a charge of −ne. Thus, we have:
q1=neandq2=−ne
4. Substitute into Coulomb's Law:
Substitute q1 and q2 into the equation:
F=k(ne)(−ne)r2=k−n2e2r2
Since force is a magnitude, we can ignore the negative sign:
F=kn2e2r2
5. Plug in the Values:
We know F=0.9N and r=1m:
0.9=9×109n2(1.6×10−19)212
6. Solve for n2:
Rearranging gives:
n2=0.9×129×109×(1.6×10−19)2
Calculate (1.6×10−19)2:
(1.6×10−19)2=2.56×10−38
Now substitute this value:
n2=0.99×109×2.56×10−38
n2=0.92.304×10−28≈3.91×1027
7. Calculate n:
Taking the square root:
n≈√3.91×1027≈6.25×1013
Final Answer:
The number of electrons that need to be removed from one sphere and added to the other is approximately 6.25×1013.
We have two identical copper spheres, and we need to calculate how many electrons need to be transferred to create a force of attraction of 0.9 N between them.
The force F between two charges q1 and q2 separated by a distance r is given by Coulomb's law:
F=kq1q2r2
where k is Coulomb's constant, approximately 9×109N m2/C2.
Since we are transferring n electrons, the charge of one electron is e=1.6×10−19C. If we remove n electrons from one sphere, it gains a charge of +ne, and the other sphere, which gains those electrons, has a charge of −ne. Thus, we have:
q1=neandq2=−ne
Substitute q1 and q2 into the equation:
F=k(ne)(−ne)r2=k−n2e2r2
Since force is a magnitude, we can ignore the negative sign:
F=kn2e2r2
We know F=0.9N and r=1m:
0.9=9×109n2(1.6×10−19)212
Rearranging gives:
n2=0.9×129×109×(1.6×10−19)2
Calculate (1.6×10−19)2:
(1.6×10−19)2=2.56×10−38
Now substitute this value:
n2=0.99×109×2.56×10−38
n2=0.92.304×10−28≈3.91×1027
Taking the square root:
n≈√3.91×1027≈6.25×1013
The number of electrons that need to be removed from one sphere and added to the other is approximately 6.25×1013.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Exercise
57 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise-I
80 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
42 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
Similar Questions
Two identical spherical masses are kept at some distance. Potential energy when a mass m is taken from the surface of one sphere to the other
View Solution(a) Two protons are placed at some separation in vacuum. Find the ratio of electric and gravitational force acting between them.
(b) Two point charges are placed at separation 3m in vacuum. What can be the minimum force between them.
(c ) A charge Q is to be divided on two objects. What should be the value of the charges in the objects so that the force between them is maximum ?
(d) Two insulating small spheres are rubbed against each other and placed 1.6cm apart. If they attract each other with a force of 0.9N, how many electrons were transferred from one sphere to the other during rubbing ?
View SolutionTwo identical conducting spheres 1 and 2 carry equal amounts of charge and are ficed apart at a certain distance larger than their diameters. The spheres repel each other with an electrical force of 88mN. A thired identical sphere 3, having an insulating handle and initially uncharged, is touched first to sphere 1 and then to sphere 2 and finally removed. Find the force between spheres 1 and 2 as shown if Figure.
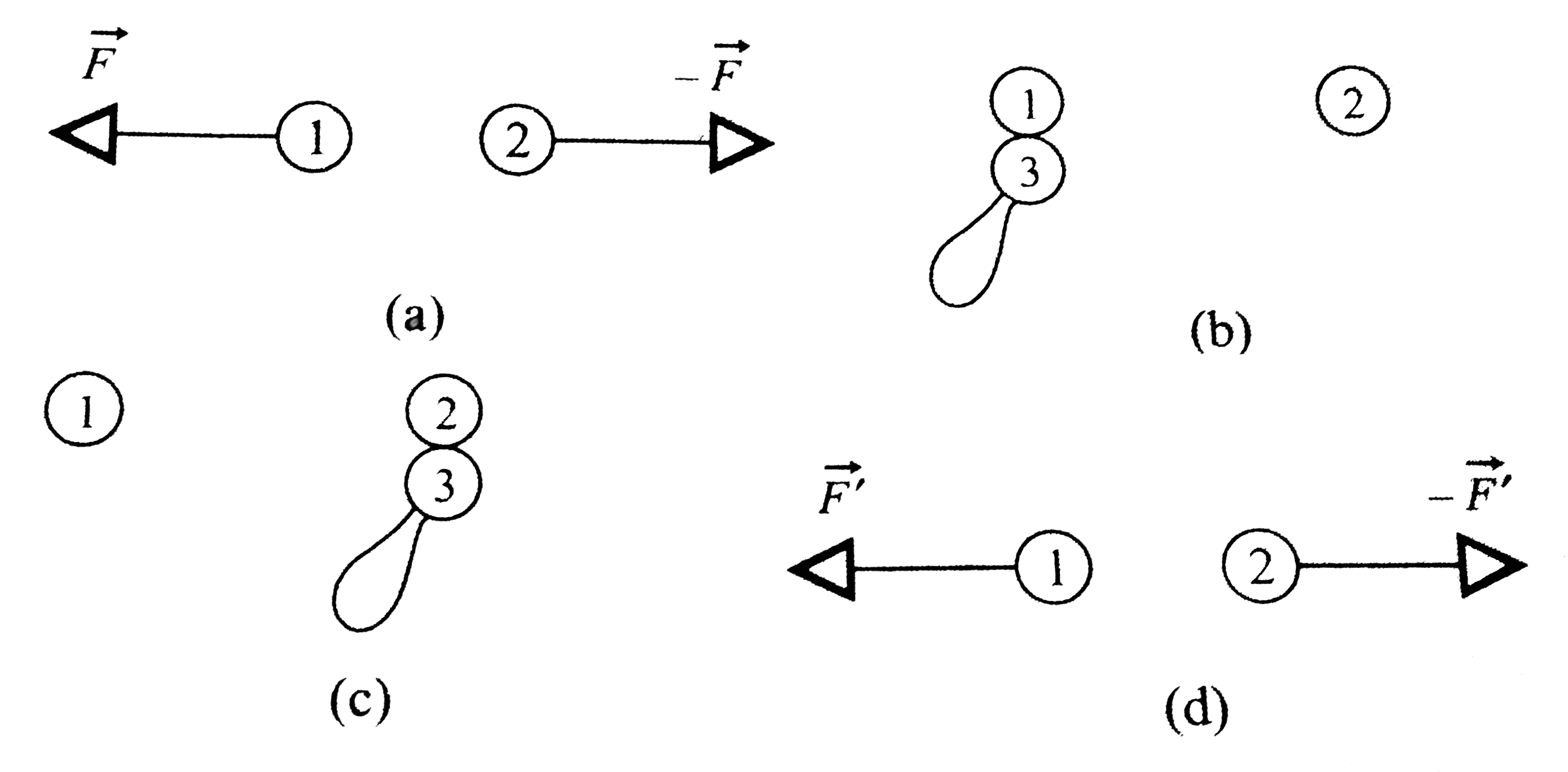 ,
,
View SolutionTwo point charges are placed in vacuum. It is observed that electrostatic force applied by one charge on the other is F in magnitude away from each other Now both the charges are placed in medium of relative permitivity ∈r at the same distance (medium extends into infinity). Select correct options
View SolutionTwo charges spheres are separated at a distance d exert a force F on each other, if charges are doubled and distance between them is doubled then the force is
View Solution(a) Two identical sphereshaving positive charges are placed 3m apart repel each other with a force 8×10−3N. Now charges are connected by a metallic wire, they begin to repel each other with a force of 9×10−3N. Find initial charges on the spheres.
(b) Two identical spheres having charges of opposite signare placed 3m apart attract other with a force 2.4×10−3N. Now spheres are touched and then placed at original separation repel with a force 10−3N. Find initial charges on the spheres.
View SolutionTwo identical copper spheres of radius R are in contact with each other. If the gravitational attraction between them is F, find the relation between F and R.
View SolutionTwo identical metal spheres having charges +q and +q respectively. When they are separated by distance r, exerts force of repulsion F on each other. The spheres are allowed to touch and then moved back to same separation. The new force of repulsion will be
View SolutionTwo identical particles are charged and held at a distance of 1m form each pther. They are found to be attraction each other with a force of 0.027N . Now they are connected by a conducting wire so that charge folws between them. Whe the charge flow stop, they are found to be repelling each other with a force of 0.009N. Find the initial charge on each particle.
View SolutionTwo copper balls, each weighing 10g are kept in air 10cm apart. If one electron from every 106 atoms in trandferred from one ball to the other, the coulomb force between them is (atomic weight of copper is 63.5)
View Solution
