Two narrow cylindrical pipes $A$ and $B$ have the same length. Pipe $A$ is open at both ends and is filled with a monoatomic gas of molar mass $M_{A}$ . Pipe $B$ is open at one end and closed at the other end and is filled with a diatomic gas of molar mass $M_{B}$ . Both gases are at the same temperature.
(a) If the frequency of the second harmonic of the fundamental mode in pipe $A$ is equal to the frequency of the third harmonic of the fundamental mode in pipe $B$ , determine the value of $\frac{m_{A}}{M_{B}}$ .
(b) Now, the open end of pipe $B$ is also closed (so that the pipe is closed at both ends.) Find the ratio of the fundamental frequency in pipe $A$ to that in pipe $B$ .

Video Solution

Text Solution

Verified by Experts

a. If $L$ is the length of each pipe $A and B$ , then fundamental frequency of pipe $A$ ( open at both ends )
$n_{A} = \frac{v_{A}}{2 L}$ (i)
Fundamental frequency of pipe $B$ ( closed at one end)
$n_{B} = \frac{v_{B}}{4 L}$ (ii)
Given $2 n_{A} = 3 n_{B}$
$2 (\frac{v_{A}}{2 L}) = 3 (\frac{v_{B}}{4 L})$
$\Rightarrow \frac{v_{A}}{v_{B}} = \frac{3}{4}$
But $v_{A} = \sqrt{\frac{γ_{A} R T_{A}}{M_{A}}}, v_{B} = \sqrt{\frac{γ_{B} R T_{B}}{M_{B}}}$
Given $T_{A} = T_{B}$
For monoatomic get
$γ_{A} = \frac{5}{3}$
For diatomic get
$γ_{B} = \frac{7}{5}$
$∴ \frac{v_{A}}{v_{B}} = \sqrt{{\frac{γ_{A}}{γ_{B}}) \frac{M_{B}}{M_{A}}}$
$\sqrt{\frac{(5 / 3)}{(7 / 5)} \frac{M_{B}}{M_{A}}}$
$= \sqrt{\frac{25}{21} \frac{M_{B}}{M_{A}}}$
(i) From Eqs.(iii) and (iv)
$\sqrt{\frac{25}{21} \frac{M_{B}}{M_{A}}} = \frac{3}{4}$
(ii) $\frac{M_{A}}{M_{B}} = {(\frac{4}{3})}^{2} \times \frac{25}{21} = \frac{400}{189}$
b. When pipe $B$ is closed at both ends , fundamental frequency of pipe $B$ becomes
$n_{B} = \frac{v_{B}}{2 L}$ (v)
Using Eqs. (i) ,(iii) and (v) , we get
iii. $\frac{n_{A}}{n_{B}} = \frac{v_{A}}{v_{B}} = \frac{3}{4}$

Updated on:21/07/2023

|

Class 11 PHYSICS TRAVELLING WAVES

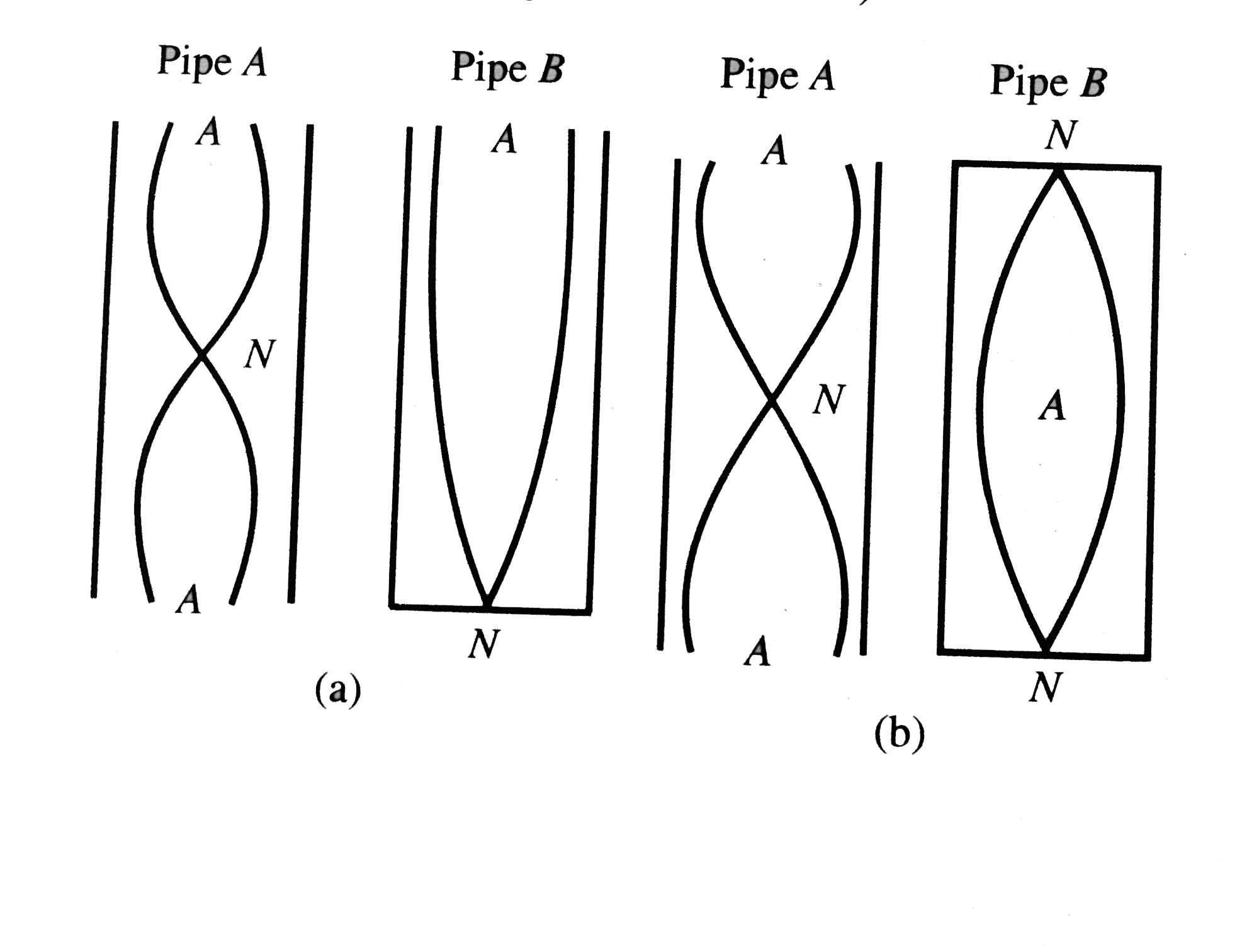
Similar Questions

Recommended Questions

Home
Ask Doubt
Profile