Two particles A and B are moving with constant velocities V1=ˆj and v2=2ˆi respectively in XY plane. At time t=0, the particle A is at co-ordinates (0,0) and B is at (-4,0). The angular velocities of B with respect to A at t=2s is (all physical quantities are in SI units)
12rad/s
2rad/s
4rad/s
1rad/s
The correct Answer is:D
To find the angular velocity of particle B with respect to particle A at time t=2 seconds, we can follow these steps:
Step 1: Determine the positions of particles A and B at t=2 seconds.
- Particle A starts at the origin (0,0) and moves with a velocity V1=^j (which means it moves in the positive y-direction).
- The displacement of particle A after 2 seconds is:
Displacement of A=V1⋅t=^j⋅2=(0,2)
- Therefore, the position of particle A at t=2 seconds is:
Position of A=(0,0)+(0,2)=(0,2)
- Particle B starts at (−4,0) and moves with a velocity V2=2^i (which means it moves in the positive x-direction).
- The displacement of particle B after 2 seconds is:
Displacement of B=V2⋅t=2^i⋅2=(4,0)
- Therefore, the position of particle B at t=2 seconds is:
Position of B=(−4,0)+(4,0)=(0,0)
Step 2: Calculate the relative position vector R from A to B.
- The position vector from A to B at t=2 seconds is given by:
R=Position of B−Position of A=(0,0)−(0,2)=(0,−2)
Step 3: Calculate the velocities of particles A and B.
- The velocity of particle A is:
VA=^j
- The velocity of particle B is:
VB=2^i
Step 4: Calculate the relative velocity VBA.
- The relative velocity of B with respect to A is:
VBA=VB−VA=(2^i)−(^j)=(2,−1)
Step 5: Calculate the magnitude of the position vector R.
- The magnitude of the position vector R is:
|R|=√(0)2+(−2)2=√4=2
Step 6: Calculate the angular velocity ω of B with respect to A.
- The angular velocity ω can be calculated using the formula:
ω=|VBA||R|
- First, we need to find the magnitude of VBA:
|VBA|=√(2)2+(−1)2=√4+1=√5
- Now substituting the values:
ω=√52
Final Answer:
The angular velocity of B with respect to A at t=2 seconds is √52 radians per second.
---
- The displacement of particle A after 2 seconds is:
Displacement of A=V1⋅t=^j⋅2=(0,2)
- Therefore, the position of particle A at t=2 seconds is:
Position of A=(0,0)+(0,2)=(0,2)
- The displacement of particle B after 2 seconds is:
Displacement of B=V2⋅t=2^i⋅2=(4,0)
- Therefore, the position of particle B at t=2 seconds is:
Position of B=(−4,0)+(4,0)=(0,0)
R=Position of B−Position of A=(0,0)−(0,2)=(0,−2)
VA=^j
- The velocity of particle B is:
VB=2^i
VBA=VB−VA=(2^i)−(^j)=(2,−1)
|R|=√(0)2+(−2)2=√4=2
ω=|VBA||R|
- First, we need to find the magnitude of VBA:
|VBA|=√(2)2+(−1)2=√4+1=√5
- Now substituting the values:
ω=√52
The angular velocity of B with respect to A at t=2 seconds is √52 radians per second.
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - More than one option is correct
36 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Comprehension Type Questions
40 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
Similar Questions
Two objects A and B are moving in opposite directions with velocities vAandvB respectively, the magnitude of relative velocity of A w.r.t. B is
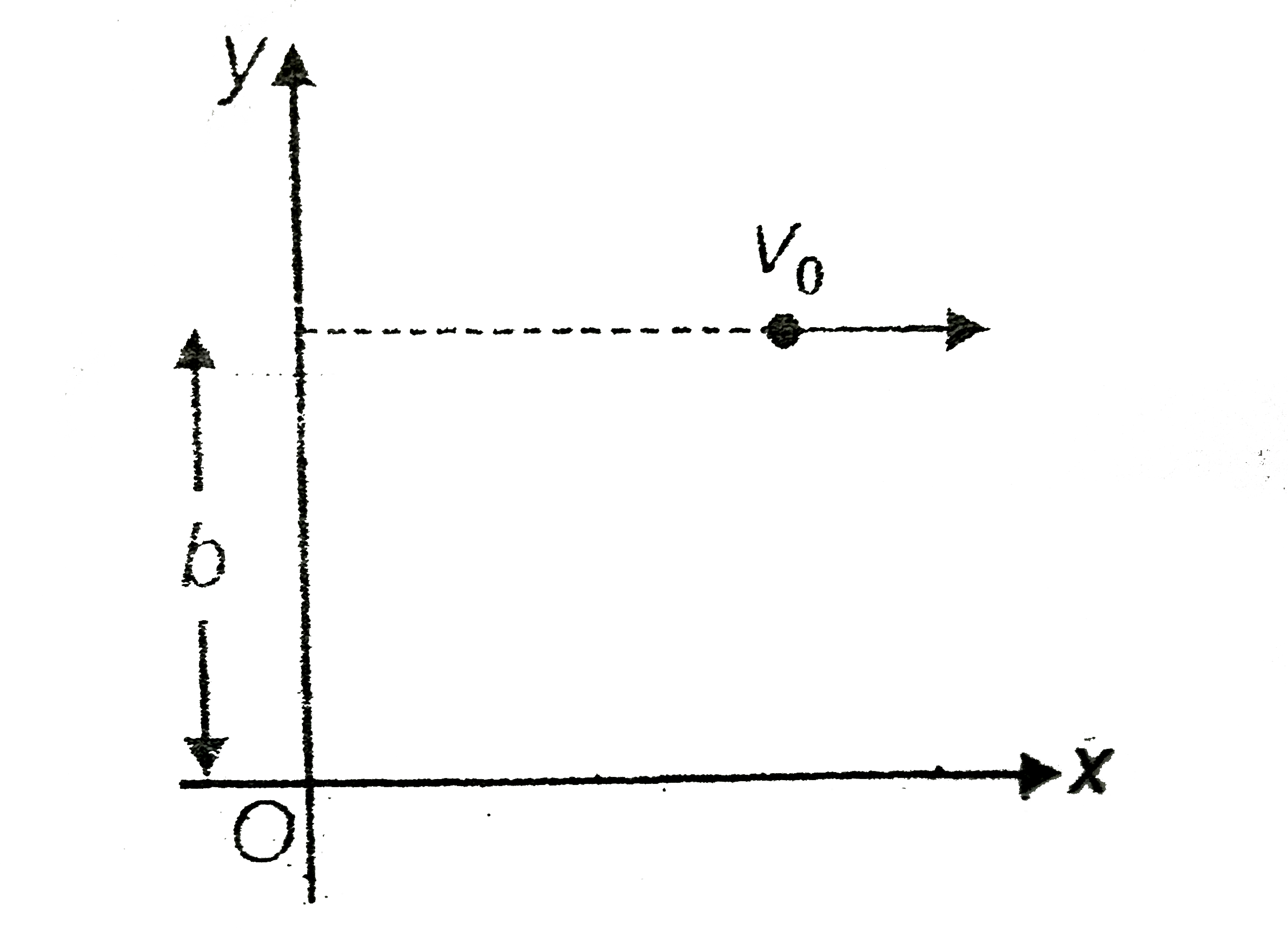
View SolutionA particle of mass m is moving with constant velocity v0 along the line y=b. At time t=0 it was at the point (0,b). At time t=bv0, the
View SolutionTwo particles are moving with velocities v1=ˆi−tˆj+ˆk and v2=tˆi+tˆj+2ˆkm/s respectively. Time at which they are moving perpendicular to each other is.____________(second)
View SolutionTwo particles of equal mass m are moving with velocities vˆi and v(ˆi+ˆj2) respectively. If the particles collide completely inelastically, then the energy lost in the process is
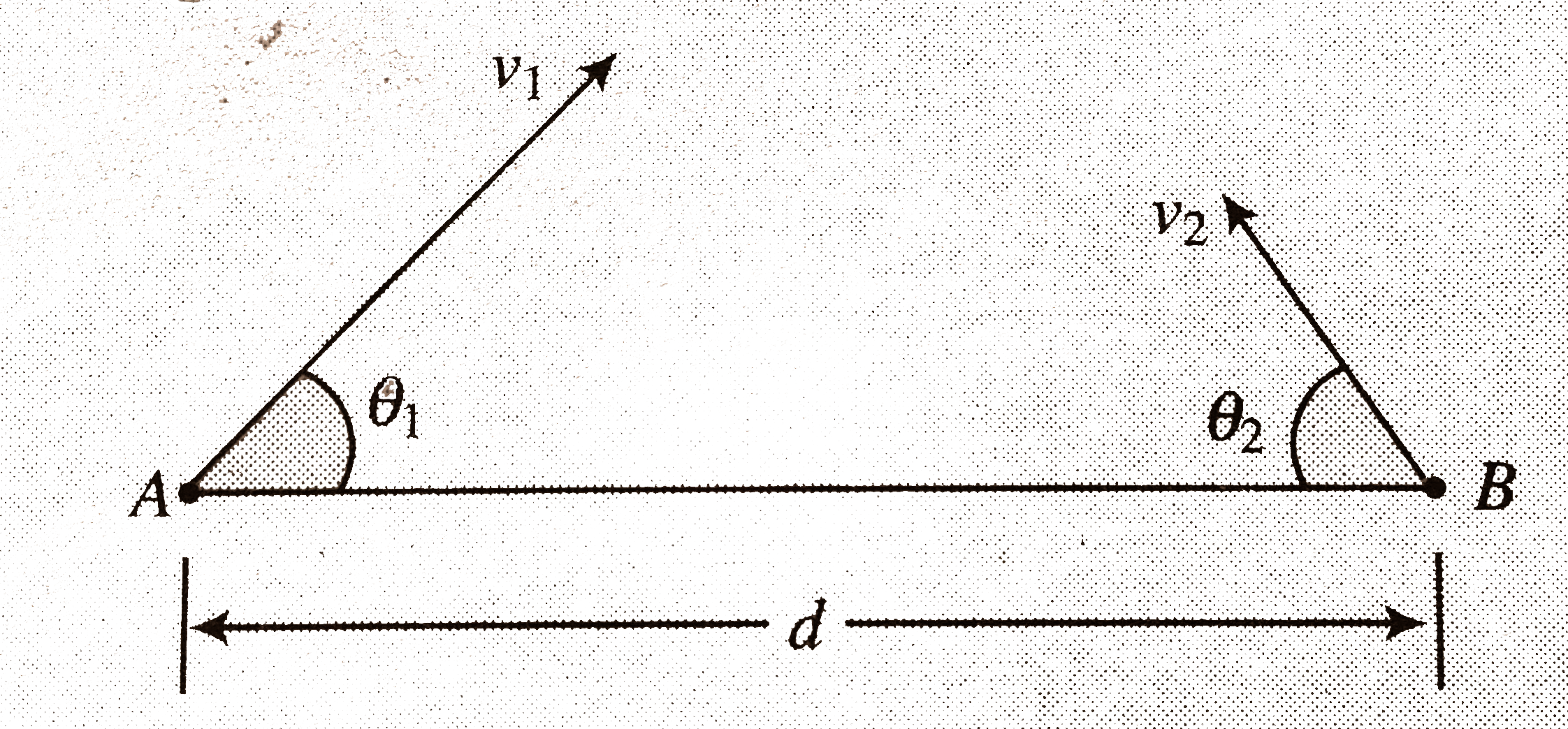
View SolutionTwo particles AandB are moving with constant velocities v1andv2. At t=0, v1 makes an angle θ0 with the line joining AandBandv2 makes an angle θ2 with the line joining AandB.
(a) Find the condition for AandB to collide.
(b) Find the time after which AandB will collide if separation between them is d at t=0.
 .
.
View SolutionTwo cars of same mass are moving with velocities v1andv2 respectively. If they are stopped by supplying same breaking power in time t1andt2 respectively then v1v2 is
View SolutionA particle moves with initial velocity v0 and retardation αv, where v is velocity at any instant t. Then the particle
View SolutionA particle at origin (0,0) moving with initial velocity u=5msˆj and acceleration 10ˆi+4ˆj. After t time it reaches at position (20,y) then find t & y
View SolutionParticles AandB move with constant and equal speeds in a circle as shown in (Fig. 5.164). Find the angular velocity of the particle A with respect to B, if the angular velocity of particle Aw.r.t.O is ω.
 .
.
View SolutionTwo particles start moving simultaneously from points (0, 0) and (1, 0) respectively in the OXY plane with uniform velocities v1 and v2 as shown in the figure. It is found that they collide. Then

View Solution
