Two particles have equal masses of 5.0 g each and opposite charges of +4.0×10−5C. They are released from rest with a separation of 1.0 m between them. Find the speeds of the particles when the separation is reducced to 50 cm.
Here, m1=m2=5.0g=5×10−3kg.
r1=1.0m,r2=50cm=12m
From symmetry , v1=v2=v=? As increase in K.E. = loss in P.E, - initial P.E.
∴2×12mv2=|q1||q2|4π∈0[1r2−1r1]
5×10−3v2=9×109(4×10−5)2[11/2−1]
=9×16×10−1(2−1)
v2=1.445×10−3=2.88×103
v=53.7m/s
Similar Questions
Two particles, each having a mass of 5 gm and charge 1.0×10−7C, stay in limiting equilirbium on a horizontal table with a separation of 10 cm between them. The coefficient of friction between each particle and the table is the same. Find the value of this coefficient.
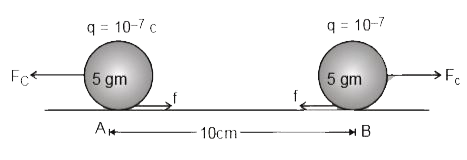
View SolutionTwo particles, each having a mass of 5 g and charge. 1.0×10−7 C, stay in limiting wquilibrium on a horizontal. table with a seaprtion of 10 cm between them. The coefficient of friction beteen each partcle and the table. is the same. Find the value of this coefficient.
View Solution
Knowledge Check
Two charged particles having charges 1and−1μC and of mass 50g each are held at rest while their separation is 2m. Now the charges are released. Find the speed of the particles when their separation is 1m.
A15ms−1B35ms−1C310ms−1D27ms−1Two point charges q1 and q2 are released from rest in a gravity free hall when distance between them is a. Find the maximum speeds of charged particle. The mass of each charged particle is m.
Aq1q24πε0aB√(q1q2)4πε0ma)C√(2q1q24πε0ma)Dnone of theseIn above question 1, find the speed of each speed of each particle, when the separation reduces to half its initial value
A√GmdB√2GmdC√Gm2dDNone of these
Two charged particles, having equal charges of 2.0×10−5C each, are brought from infinity to within a separation of 10 cm. Find the increase in the electric potential energy during the process.
View SolutionTwo identical particles, each having a charge of 2.0×10−4C and then released. What would be the speeds of the particles when the separtion becomes large?
View SolutionTwo small bodies of masses 10 kg and 20 kg are kept a distnce 1.0 m apart and released. Assuming that only mutual gravitational force are acting, find the speeds of te particles when the separation decreases to 0.5 m.
View SolutionTwo charged particles having charge 1μC and −μC and of mass 50gm each are held ar rest while their separtion is 2m. Find the relative velocity of the particles when their separation is 0.5m.
View SolutionOne particle has a mass of 3.0×10−3kg and a charge of +8.00μC. A second particle has a mass of 6.0×10−3 kg and place and then released. The particles fly apart, and when the separation between them is 0.100 m, the speed of the 3.00×10−3 kg particle is 125 mis. Find the initial separation between the particles.
View Solution
