Two point charges Q and -3Q are placed at some distance apart. If the electric field at the location of Q is →E, the field at the location of - 3Q is
→E
−→E
+→E3
−→E3
The correct Answer is:C
To solve the problem, we need to find the electric field at the location of the charge -3Q, given that the electric field at the location of charge Q is →E.
1. Understanding Electric Field due to Point Charges:
The electric field →E due to a point charge Q at a distance r is given by the formula:
→E=kQr2
where k is Coulomb's constant.
2. Identify the Charges and Their Positions:
Let’s denote the position of charge Q as point A and the position of charge -3Q as point B. The distance between the two charges is r.
3. Calculate Electric Field at Point A (due to -3Q):
The electric field at point A (where charge Q is located) due to charge -3Q is directed towards charge -3Q (since it is negative). The magnitude of this electric field is:
→EA=k−3Qr2
4. Given Electric Field at Point A:
We are given that the electric field at the location of charge Q (point A) is →E. This means:
→E=k−3Qr2
5. Calculate Electric Field at Point B (due to Q):
Now, we need to find the electric field at point B (where charge -3Q is located) due to charge Q. The electric field at point B due to charge Q is directed away from charge Q (since it is positive). The magnitude of this electric field is:
→EB=kQr2
6. Relate the Electric Fields:
Since we have established that →E=k−3Qr2, we can express Q in terms of →E:
Q=−Er23k
Now substituting this into the expression for →EB:
→EB=kQr2=k−Er23kr2=−E3
7. Final Result:
Therefore, the electric field at the location of charge -3Q is:
→EB=−→E3
Conclusion:
The electric field at the location of charge -3Q is −→E3.
The electric field →E due to a point charge Q at a distance r is given by the formula:
→E=kQr2
where k is Coulomb's constant.
Let’s denote the position of charge Q as point A and the position of charge -3Q as point B. The distance between the two charges is r.
The electric field at point A (where charge Q is located) due to charge -3Q is directed towards charge -3Q (since it is negative). The magnitude of this electric field is:
→EA=k−3Qr2
We are given that the electric field at the location of charge Q (point A) is →E. This means:
→E=k−3Qr2
Now, we need to find the electric field at point B (where charge -3Q is located) due to charge Q. The electric field at point B due to charge Q is directed away from charge Q (since it is positive). The magnitude of this electric field is:
→EB=kQr2
Since we have established that →E=k−3Qr2, we can express Q in terms of →E:
Q=−Er23k
Now substituting this into the expression for →EB:
→EB=kQr2=k−Er23kr2=−E3
Therefore, the electric field at the location of charge -3Q is:
→EB=−→E3
The electric field at the location of charge -3Q is −→E3.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Exercise
57 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise-I
80 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
42 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
Similar Questions
The point charges Q and −2Q are placed at some distance apart. If the electirc field at the location of Q is E, the electric field at the location of −2Q will be
View Solution10C and 20C are separated by a distance d. If the electric field at the location of a charge 10C is →E, the field at the location of 20C is
View SolutionTwo point charges +Q and -Q are separated by a certain distance. The resultant electric field is parallel to the line joining the charges at the points
View SolutionTwo charges 4q and q are placed 30 cm. apart. At what point the value of electric field will be zero
View SolutionTwo point charges +q and −q are placed a distance x apart. A third charge is so placed that at the three charges are in equilibrium. Then
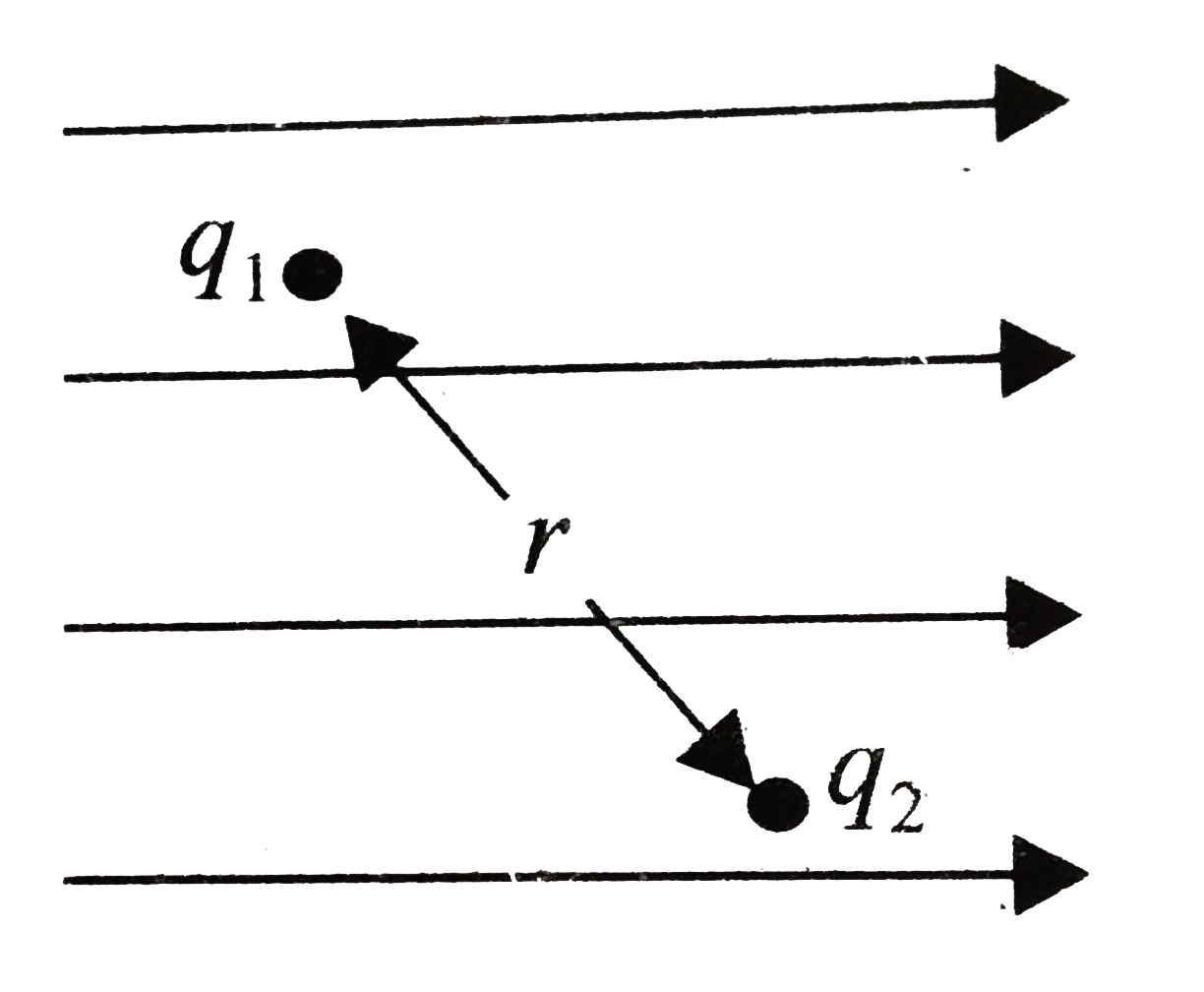
View SolutionTwo point charges q1andq2 are placed in an external uniform electric field as shown in figure. The potential at the location of q1andq2 are V1andV2, i.e., V1andV2 are potentials at location of q1andq2 due to external unspecified charges only. Then electric potential energy for this configuration of two charged particle is
View SolutionTwo point charges q and -q are separated by a distance 2a. Evaluate the flux of the electric field strength vector across a circle of radius R.

View SolutionCharges Q and -2Q are placed at some distance. The locus of points in the plane of the charges where the potential is zero will be :
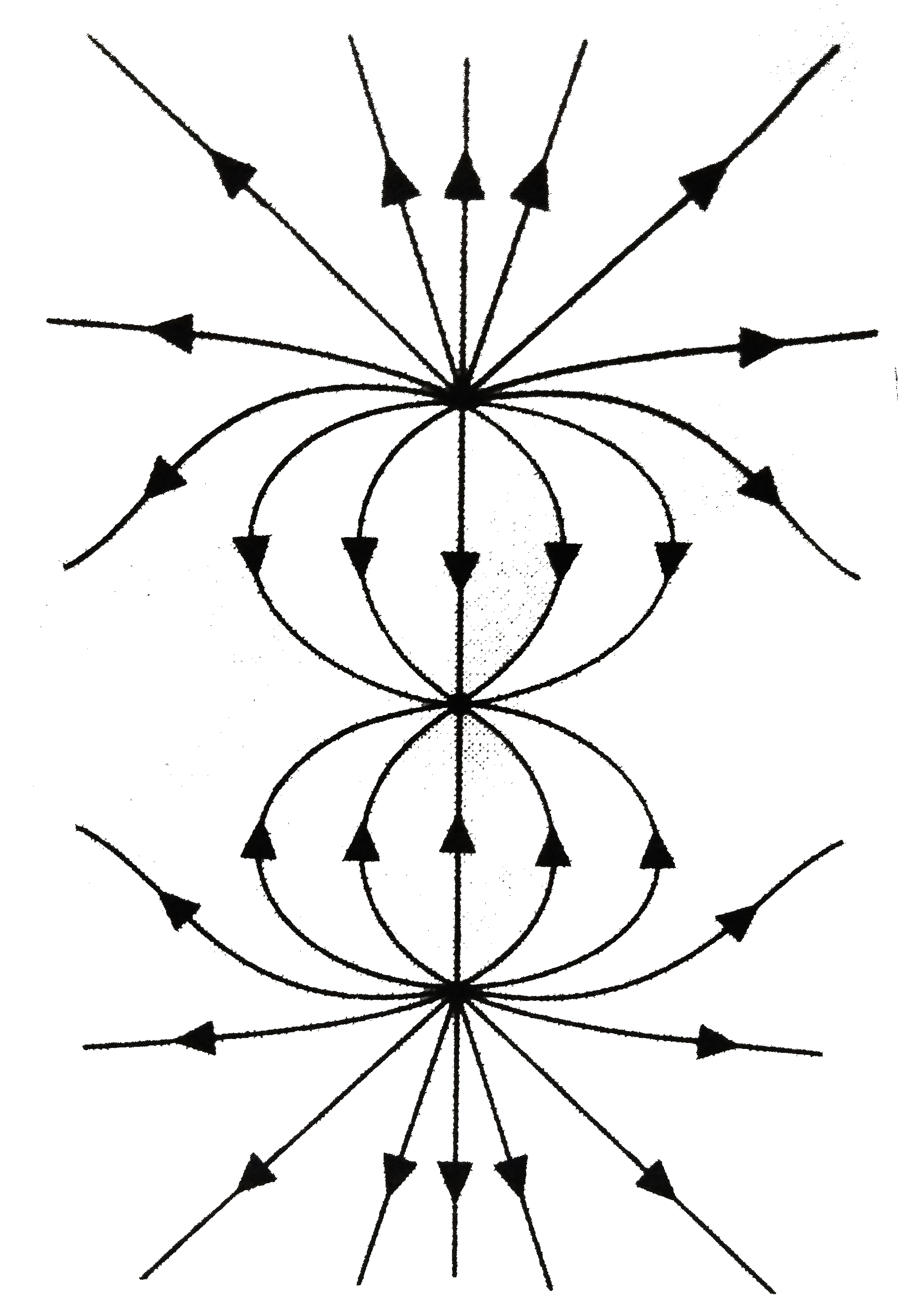
View SolutionFig. shows some of the electric field lines due to three point charges arranged along the vertical axis. All three charges have the same magnitude.
(a) What are the signs of each of the three charges? Explain your reasoning.
(b) At what points(s) is the magnitude of the electric field the smallest? Explain your reasoning. Explain how the fields produced by each individual point charge combine to give a small net field at this point or points.
(c ) Two point charges q and -q are placed at a distance d apart. What are the points at which the resultant electric field is parallel to the line joining the two charge?
 .
.
View SolutionTwo point charges +4q and +q are placed at a distance L apart. A third charge Q is so placed that all the three charges are in equilibrium. Then location. And magnitude of the third charge will be
View Solution
