Two spherical planets P and Q have the same uniform density ρ, masses MpandMQ and surface areas A and 4A respectively. A spherical planet R also has uniform density ρ and its mass is (MP+MQ). The escape velocities from the plantes P,Q and R are VPVQandVR respectively. Then
VQ>VR>VP
VR>VQ>VP
VR/VP=3
VP/VQ=12
The correct Answer is:B, D
To solve the problem, we need to find the relationship between the escape velocities of the three planets P, Q, and R based on their masses and radii.
Step 1: Understanding Escape Velocity
The escape velocity V from a planet is given by the formula:
V=√2GMR
where G is the gravitational constant, M is the mass of the planet, and R is the radius of the planet.
Step 2: Relating Surface Area to Radius
Given that the surface area A of planet P is A and that of planet Q is 4A, we can relate the radii of the two planets using the formula for surface area of a sphere:
A=4πR2
For planet P:
A=4πR2P⟹R2P=A4π⟹RP=√A4π
For planet Q:
4A=4πR2Q⟹R2Q=4A4π⟹RQ=√4A4π=2√A4π=2RP
Thus, we have:
RQ=2RP
Step 3: Relating Mass to Radius
The mass of a planet is given by:
M=ρ⋅V=ρ⋅(43πR3)
For planet P:
MP=ρ⋅43πR3P
For planet Q:
MQ=ρ⋅43πR3Q=ρ⋅43π(2RP)3=ρ⋅43π⋅8R3P=8MP
Thus, we have:
MQ=8MP
Step 4: Mass of Planet R
The mass of planet R is given as:
MR=MP+MQ=MP+8MP=9MP
Step 5: Finding Radius of Planet R
Using the mass of planet R, we can find its radius:
MR=ρ⋅43πR3R⟹9MP=ρ⋅43πR3R
Substituting MP:
9(ρ⋅43πR3P)=ρ⋅43πR3R
This simplifies to:
9R3P=R3R⟹RR=91/3RP=3RP
Step 6: Finding Escape Velocities
Now we can express the escape velocities:
VP=√2GMPRP,VQ=√2G(8MP)2RP=√16GMP2RP=√8GMPRP=2√2GMPRP=2VP
For planet R:
VR=√2G(9MP)3RP=√18GMP3RP=√6GMPRP=√3VP
Step 7: Comparing Escape Velocities
Now we have:
- VP=VP
- VQ=2VP
- VR=√3VP
Thus, the relationship is:
VQ>VR>VP
Final Answer
The correct relationship is:
VQ>VR>VP
The escape velocity V from a planet is given by the formula:
V=√2GMR
where G is the gravitational constant, M is the mass of the planet, and R is the radius of the planet.
Given that the surface area A of planet P is A and that of planet Q is 4A, we can relate the radii of the two planets using the formula for surface area of a sphere:
A=4πR2
For planet P:
A=4πR2P⟹R2P=A4π⟹RP=√A4π
For planet Q:
4A=4πR2Q⟹R2Q=4A4π⟹RQ=√4A4π=2√A4π=2RP
Thus, we have:
RQ=2RP
The mass of a planet is given by:
M=ρ⋅V=ρ⋅(43πR3)
For planet P:
MP=ρ⋅43πR3P
For planet Q:
MQ=ρ⋅43πR3Q=ρ⋅43π(2RP)3=ρ⋅43π⋅8R3P=8MP
Thus, we have:
MQ=8MP
The mass of planet R is given as:
MR=MP+MQ=MP+8MP=9MP
Using the mass of planet R, we can find its radius:
MR=ρ⋅43πR3R⟹9MP=ρ⋅43πR3R
Substituting MP:
9(ρ⋅43πR3P)=ρ⋅43πR3R
This simplifies to:
9R3P=R3R⟹RR=91/3RP=3RP
Now we can express the escape velocities:
VP=√2GMPRP,VQ=√2G(8MP)2RP=√16GMP2RP=√8GMPRP=2√2GMPRP=2VP
For planet R:
VR=√2G(9MP)3RP=√18GMP3RP=√6GMPRP=√3VP
Now we have:
- VP=VP
- VQ=2VP
- VR=√3VP
VQ>VR>VP
The correct relationship is:
VQ>VR>VP
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - AR_TYPE2videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - LC_TYPE8videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - SCQ_TYPE29videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
Similar Questions
Three particales P,Q and R are placedd as per given Masses of P,Q and R are √3m√3m and m respectively The gravitational force on a fourth particle 'S' of mass m is equal to
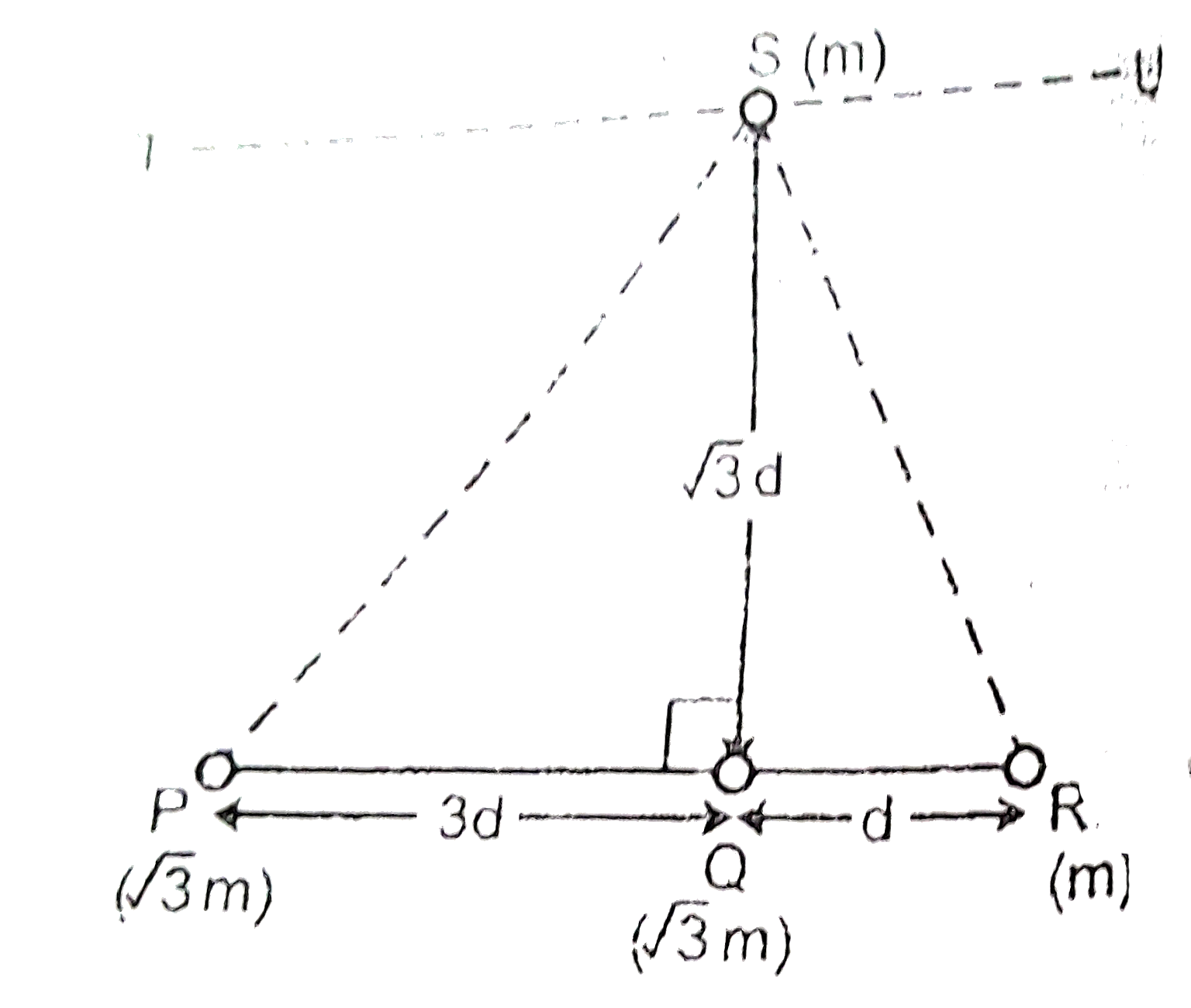 .View Solution
.View SolutionTwo particles p and q located at distances rp and rq respectively from the centre of a rotating disc such that rp>rq.
View SolutionTwo planets of masses M and M2 have radii R and R2 respectively. If ratio of escape velocities from their surfaces v1v2 is n4 , then find n :
View SolutionThe (m+n)thand(m−n)th terms of a G.P. are pandq respectively. Show that the mth and nth terms are √pqandp(qp)m/2n respectively.
View SolutionA planet is revolving around the sun. its distance from the sun at apogee is rA and that at perigee is rp. The masses of planet and sun are 'm' and M respectively, VA is the velocity of planet at apogee and VP is at perigee respectively and T is the time period of revolution of planet around the sun, then identify the wrong answer.
View SolutionTwo points P and Q are maintained at the potentials of 10V and -4V, respectively. The work done in moving 100 electrons from P to Q is:
View SolutionThe quantities P and Q are related by PQ=μ where μ is the linear mass density and Q is specific latent heat. Then dimension of P will be same as
View SolutionIn ΔPQR, if PQ=QR and L,M and N are the mid-points of the sides PQ,QR and RP respectively. Prove that LN=MN.
View SolutionTwo satellites P and Q go round a planet in circular orbits of radii 9R and R respectively. If the speed of the satellite P is 4V, then speed of satellite Q will be
View SolutionSum of the first p, q and r terms of an A.P are a, b and c, respectively.Prove that ap(q−r)+bq(r−p)+cr(p−q)=0
View Solution
