Question

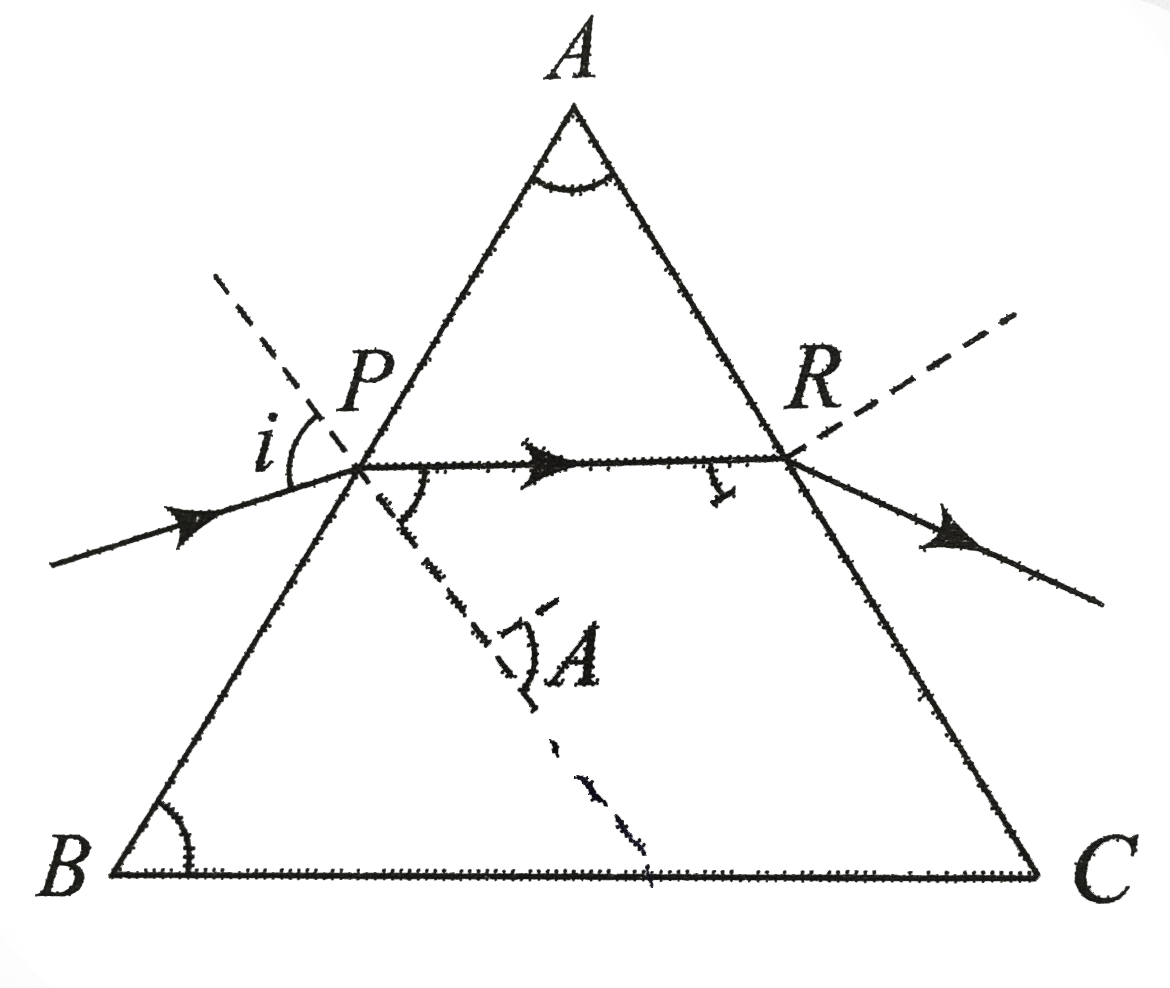
What is the angle of incidence for an equilateral prism of refractive index $\sqrt{3}$ so that the ray si parallel to the base inside the prism?

Options

A. `30^(@)`
B. `45^(@)`
C. `60^(@)`
D. Either `30^(@)` or `60^(@)`

Answer

c.

Explanation

To find the angle of incidence for an equilateral prism with a refractive index of $$\sqrt{3}$$ such that the ray is parallel to the base inside the prism, we can follow these steps:

1. Understand the Problem: We need to find the angle of incidence \( i \) such that the light ray inside the prism is parallel to the base. This condition occurs when the light ray experiences the angle of minimum deviation \( \delta_m \).

2. Use the Formula for Minimum Deviation: The formula relating the refractive index \( \mu \), the angle of the prism \( A \), and the angle of minimum deviation \( \delta_m \) is given by:
\( \mu = \frac{\sin\left(\frac{A + \delta_m}{2}\right)}{\sin\left(\frac{A}{2}\right)} \)

3. Identify Known Values:
- The refractive index \( \mu = \sqrt{3} \)
- For an equilateral prism, the angle of the prism \( A = 60^\circ \).

4. Substitute Values into the Formula:
\( \sqrt{3} = \frac{\sin\left(\frac{60^\circ + \delta_m}{2}\right)}{\sin\left(\frac{60^\circ}{2}\right)} \)
\( \sqrt{3} = \frac{\sin\left(\frac{60^\circ + \delta_m}{2}\right)}{\sin(30^\circ)} \)

5. Calculate \( \sin(30^\circ) \):
\( \sin(30^\circ) = \frac{1}{2} \)
Thus, the equation simplifies to:
\( \sqrt{3} = 2 \cdot \sin\left(\frac{60^\circ + \delta_m}{2}\right) \)

6. Rearranging the Equation:
\( \sin\left(\frac{60^\circ + \delta_m}{2}\right) = \frac{\sqrt{3}}{2} \)

7. Finding the Angle:
The value of \( \sin^{-1}\left(\frac{\sqrt{3}}{2}\right) \) corresponds to \( 60^\circ \):
\( \frac{60^\circ + \delta_m}{2} = 60^\circ \)
Therefore,
\( 60^\circ + \delta_m = 120^\circ \implies \delta_m = 60^\circ \)

8. Using the Relation for Angle of Incidence:
For minimum deviation, the relationship between angle of incidence \( i \) and angle of emergence \( e \) is:
\( i + e = A + \delta_m \)
Since \( i = e \) at minimum deviation, we have:
\( 2i = A + \delta_m \)
Substituting the known values:
\( 2i = 60^\circ + 60^\circ = 120^\circ \)
Thus,
\( i = \frac{120^\circ}{2} = 60^\circ \)

Final Answer:
The angle of incidence \( i \) is \( 60^\circ \).