White light is incident normally on a glass plate (in air) of thickness 500nm and refractive index of 1.5. The wavelength (in nm) in the visible region (400nm−700nm) that is strongly reflected by the plate is:
450
600
400
500
The correct Answer is:B
To solve the problem, we need to determine the wavelength of light that is strongly reflected by a glass plate of thickness 500 nm and refractive index 1.5. The steps to find the solution are as follows:
Step 1: Understand the Path Difference
When light reflects off the surface of the glass plate, it travels a distance of 2t (where t is the thickness of the plate) in the glass. The path difference (Δ) for light reflecting off the two surfaces of the glass plate is given by:
Δ=2μt
where μ is the refractive index of the glass.
Step 2: Calculate the Path Difference
Given:
- Thickness t=500nm
- Refractive index μ=1.5
Substituting these values into the equation:
Δ=2×1.5×500nm=1500nm
Step 3: Determine the Condition for Strong Reflection
For constructive interference (strong reflection), the path difference must satisfy the condition:
Δ=(2n−1)λ2
where n is an integer (1, 2, 3, ...), and λ is the wavelength of light.
Step 4: Rearranging the Equation
Rearranging the equation to solve for λ:
λ=4Δ2n−1
Step 5: Substitute the Path Difference
Substituting Δ=1500nm:
λ=4×15002n−1=60002n−1
Step 6: Calculate Wavelength for Different Values of n
Now, we will calculate λ for different integer values of n:
- For n=1:
λ=60002×1−1=60001=6000nm(Not in visible range)
- For n=2:
λ=60002×2−1=60003=2000nm(Not in visible range)
- For n=3:
λ=60002×3−1=60005=1200nm(Not in visible range)
- For n=4:
λ=60002×4−1=60007≈857.14nm(Not in visible range)
- For n=5:
λ=60002×5−1=60009≈666.67nm(In visible range)
- For n=6:
λ=60002×6−1=600011≈545.45nm(In visible range)
Conclusion
The wavelengths in the visible region that are strongly reflected by the glass plate are approximately 666.67nm and 545.45nm. The strongest reflection occurs at 600nm when n=5.
When light reflects off the surface of the glass plate, it travels a distance of 2t (where t is the thickness of the plate) in the glass. The path difference (Δ) for light reflecting off the two surfaces of the glass plate is given by:
Δ=2μt
where μ is the refractive index of the glass.
Given:
- Thickness t=500nm
- Refractive index μ=1.5
Δ=2×1.5×500nm=1500nm
For constructive interference (strong reflection), the path difference must satisfy the condition:
Δ=(2n−1)λ2
where n is an integer (1, 2, 3, ...), and λ is the wavelength of light.
Rearranging the equation to solve for λ:
λ=4Δ2n−1
Substituting Δ=1500nm:
λ=4×15002n−1=60002n−1
Now, we will calculate λ for different integer values of n:
λ=60002×1−1=60001=6000nm(Not in visible range)
λ=60002×2−1=60003=2000nm(Not in visible range)
λ=60002×3−1=60005=1200nm(Not in visible range)
λ=60002×4−1=60007≈857.14nm(Not in visible range)
λ=60002×5−1=60009≈666.67nm(In visible range)
λ=60002×6−1=600011≈545.45nm(In visible range)
The wavelengths in the visible region that are strongly reflected by the glass plate are approximately 666.67nm and 545.45nm. The strongest reflection occurs at 600nm when n=5.
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - LEVEL 2 (single correct option)
1 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 2 More Than One Correct
6 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Subjective Questions
5 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
Similar Questions
White light is incident normally on a glass plate of thickness 0.50×10−6 and index of refraction 1.50. Which wavelength in the visible region (400 nm - 700 nm) are strongly reflacted by the plate ?
View SolutionA parallel beam of white light is incident normally on a water film1.0x10−4 cm thick. Find the wavelength in the visible range (400 nm-700 nm) which are strongly transmitted by the film Refractive index of water = 1.33.
View Solution
Knowledge Check
A beam of light is incident at the polarizing angle of 35∘ on a certain glass plate. The refractive index of the glass plate is:
Asin35∘Btan35∘Ctan55∘Dsin55∘
A glass of refractive index 1.5 is coated with a thin layer of thickness t and refractive index 1.8 . Light of wavelength λ travelling in air is incident normally on the layer. It is partly reflected at the upper and the lower surfaces of the layer. It is partly reflected at the upper and the lower surfaces of the layer ant the two reflected rays interface . If λ=648nm, obtain the least value of t(in10−8m) which the rays interface constructively.
View SolutionA glass surface is coated by an oil film of uniform thickness 1.00×10−4cm. The index of refraction of the oil is 1.25 and that of the glass is 1.50. Find the wavelengths of light in the visible region (400nm-750nm) which are completely transmitted by the oil film under normal incidence.
View SolutionFind the speed of light of wavelength λ=780nm (in air) in a medium of refractive index μ=1.55.
(b) What is the wavelength of this light in the given medium ?
View SolutionA YDSE is performed in a medium of refractive index 4/3, A light of 600 nm wavelength is falling on the slits having 0.45 nm separation . The lower slit S2 is covered b a thin glass plate of thickness 10.4 mm and refractive index 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in figure. (All the wavelengths in this problem are for the given medium of refractive index 4/3, ignore absorption.)
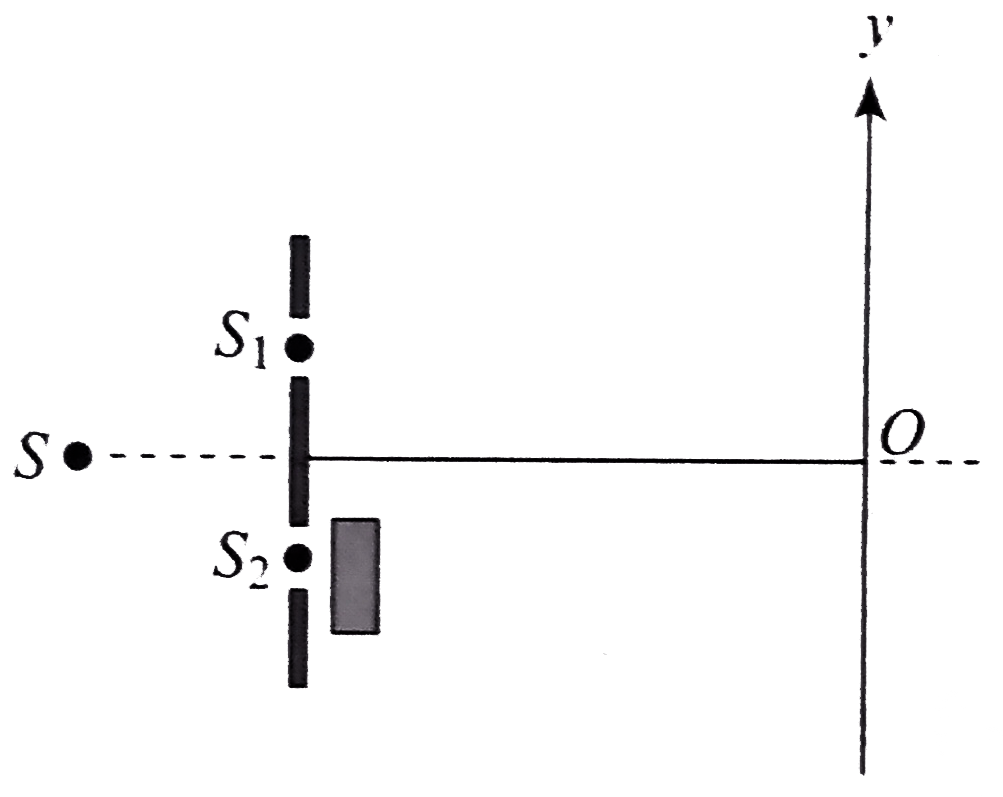
Now, if 600 nm, find the wavelength of the ligth that forms maximum exactly at point O.
View SolutionA YDSE is performed in a medium of refractive index 4/3, A light of 600 nm wavelength is falling on the slits having 0.45 nm separation . The lower slit S2 is covered b a thin glass plate of thickness 10.4 mm and refractive index 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in figure. (All the wavelengths in this problem are for the given medium of refractive index 4/3, ignore absorption.)
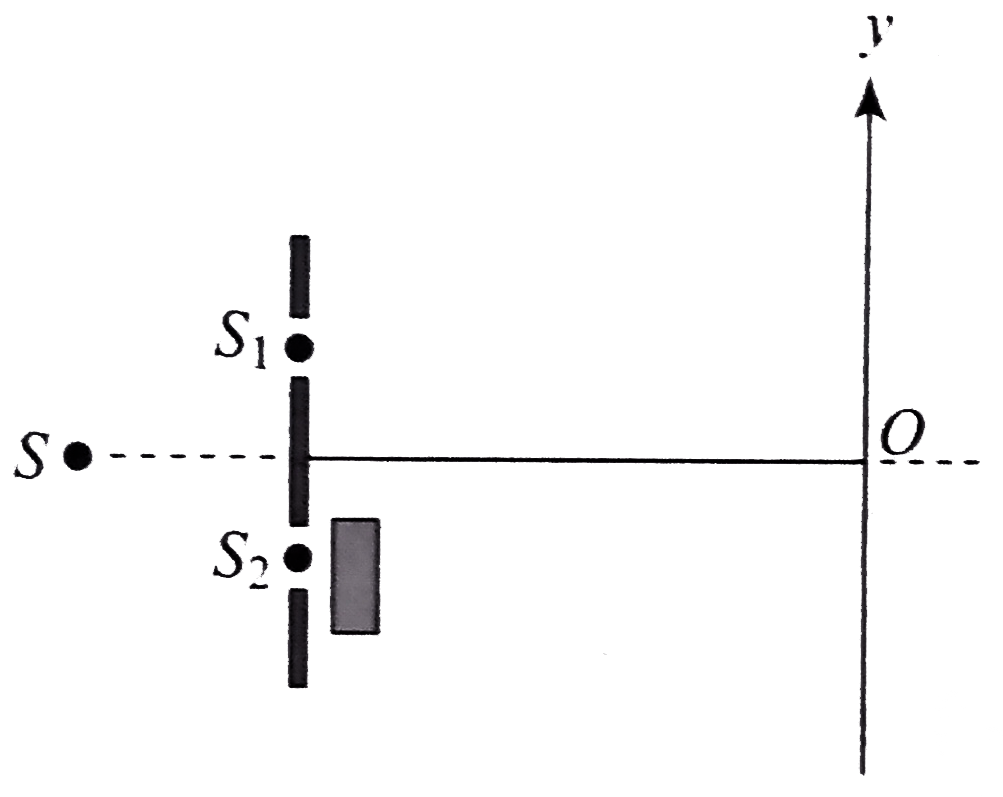
Find the light intensity at point O relative t maximum fringe intensity.
View SolutionFind the wavelength in a hydrogen spectrum between the range 500nm→700nm
View SolutionA soap bubble (n=1.33) is floating in air. If the thickness of the bubble wall is 115 nm, what is the wavelength of the light that is most strongly reflected?
View Solution
