YDSE is carried out in a liquid of refractive index μ=1.3 and a thin film of air is formed in front of the lower slit as shown in the figure. If a maxima of third order is formed at the origin O, find the thickness of the air film. Find the positions of the fourth maxima. The wavelength of light is air is λ0=0.78μm and D/d=1000.
The correct Answer is:A, B, D
To solve the problem step by step, we will follow the concepts of Young's Double Slit Experiment (YDSE) and the interference of light in a medium with a refractive index.
Step 1: Understanding the Setup
In the YDSE setup, we have two slits (S1 and S2) and a screen where the interference pattern is observed. The experiment is conducted in a liquid with a refractive index μ=1.3, and there is a thin film of air in front of the lower slit (S2).
Step 2: Wavelength in the Medium
The wavelength of light in the medium (liquid) can be calculated using the formula:
λ=λ0μ
where λ0=0.78μm (wavelength in air) and μ=1.3.
Calculating this gives:
λ=0.78μm1.3=0.6μm
Step 3: Condition for Maxima
For constructive interference (maxima), the condition is given by:
t=(m+12)λμ
where t is the thickness of the air film, m is the order of the maxima, and λ is the wavelength in the medium.
Step 4: Finding Thickness for Third Order Maxima
Given that the third order maximum is formed at the origin (O), we take m=3:
t=(3+12)⋅λμ=(3.5)⋅0.6μm1.3
Calculating this gives:
t=2.1μm1.3≈1.615μm
Step 5: Finding Position of Fourth Maxima
For the fourth order maximum (m=4):
t=(4+12)⋅λμ=(4.5)⋅0.6μm1.3
Calculating this gives:
t=2.7μm1.3≈2.077μm
Step 6: Finding Position on the Screen
The position of the maxima on the screen can be calculated using the formula:
y=m⋅D⋅λd
where D is the distance from the slits to the screen, and d is the distance between the slits. Given Dd=1000, we can express y for the fourth maximum:
y4=4⋅D⋅λd
Substituting λ=0.6μm:
y4=4⋅1000⋅0.6μm1=2400μm=2.4mm
Final Results
1. The thickness of the air film for the third order maximum is approximately 1.615μm.
2. The position of the fourth maximum is approximately 2.4mm.
In the YDSE setup, we have two slits (S1 and S2) and a screen where the interference pattern is observed. The experiment is conducted in a liquid with a refractive index μ=1.3, and there is a thin film of air in front of the lower slit (S2).
The wavelength of light in the medium (liquid) can be calculated using the formula:
λ=λ0μ
where λ0=0.78μm (wavelength in air) and μ=1.3.
λ=0.78μm1.3=0.6μm
For constructive interference (maxima), the condition is given by:
t=(m+12)λμ
where t is the thickness of the air film, m is the order of the maxima, and λ is the wavelength in the medium.
Given that the third order maximum is formed at the origin (O), we take m=3:
t=(3+12)⋅λμ=(3.5)⋅0.6μm1.3
Calculating this gives:
t=2.1μm1.3≈1.615μm
For the fourth order maximum (m=4):
t=(4+12)⋅λμ=(4.5)⋅0.6μm1.3
Calculating this gives:
t=2.7μm1.3≈2.077μm
The position of the maxima on the screen can be calculated using the formula:
y=m⋅D⋅λd
where D is the distance from the slits to the screen, and d is the distance between the slits. Given Dd=1000, we can express y for the fourth maximum:
y4=4⋅D⋅λd
Substituting λ=0.6μm:
y4=4⋅1000⋅0.6μm1=2400μm=2.4mm
1. The thickness of the air film for the third order maximum is approximately 1.615μm.
2. The position of the fourth maximum is approximately 2.4mm.
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 2 Single Correct
11 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - LEVEL 2 (single correct option)
1 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 1Subjective
22 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
Similar Questions
When the plastic thin film of refractive index 1.45is placed in the path of one of the interfering waves then the central fringe is displaced through width of five fringes.The thickness of the film will be ,if the wavelength of light is5890Å
View SolutionA glass sphere of refractive index 1.5 forms the real image of object O at point I as shown in the figure when kept in air of refractive index 1. If the value of x is kR. Find k
View Solution
Knowledge Check
On introducing a thin film in the path of one of the two interfering beam, the central fringe will shift by one fringe width. If μ=1.5, the thickness of the film is (wavelength of monochromatic light is λ)
A4λB3λC2λDλ
In the YDSE shown the two slits are covered with thin sheets having thickness t & 2t and refractive index 2μ and μ .Find the position (y) of central maxima.
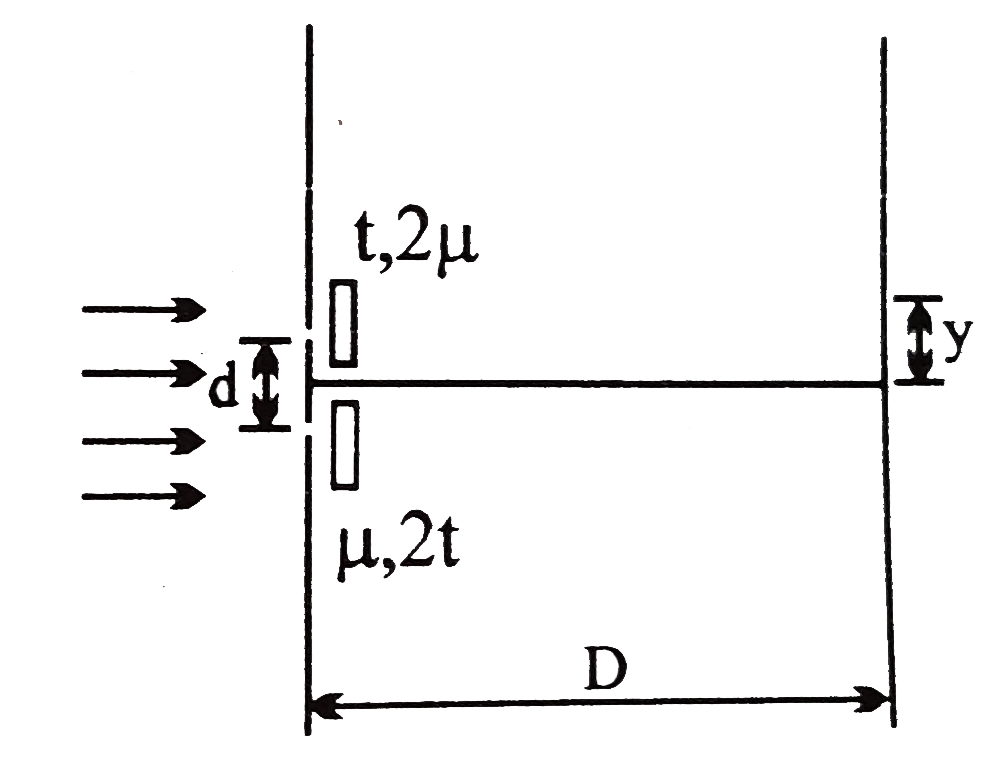
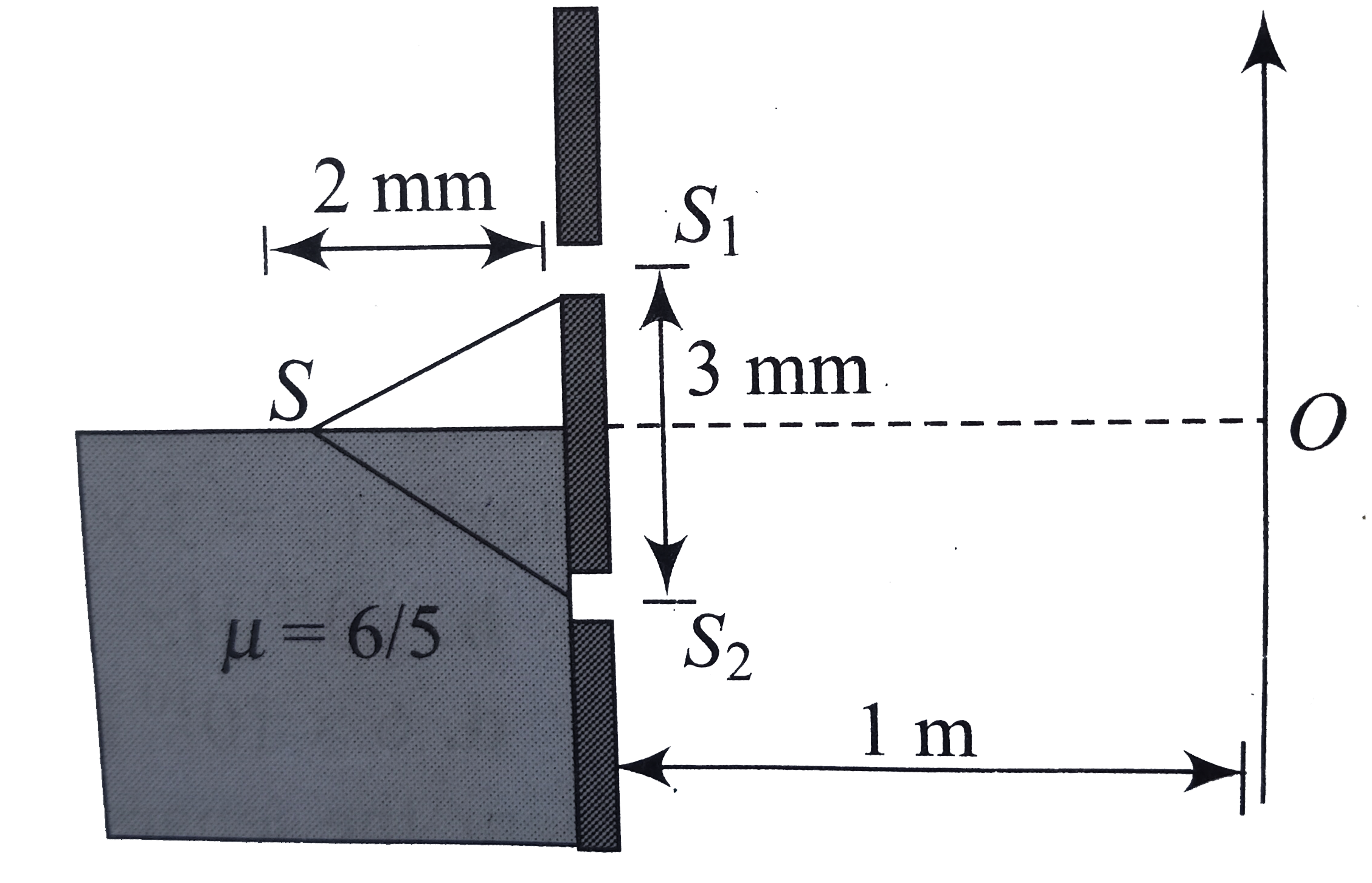
View SolutionIn Young's double-slit experiment, a point source is placed on a solid slab of refractive index 6/5 at a distance of 2 mm from two slits spaced 3 mm apart as shown and at equal distacne from both the slits. The screen is at a distance of 1 m from the slits. Wavelength of light used is 500 nm.
a. Find the position of the central maximum.
b. Find the order of the fringe formed at O.
c. A film of refractive index 1.8 is to be placed in front of S1 so that central maxima is formed where 200th maxima was formed. Find the thickness of film.
View SolutionIn YDSE when slab of thickness t and refractive index μ is placed in front of one slit then central maxima shifts by one fringe width. Find out t in terms of λ and μ.
View SolutionA light of wavelength 5890Å falls normally on a thin air film. The minimum thickness of the film such that the film appears dark in reflected light is
View SolutionA thin lens made of a material of refractive index μ0 has a focal length f0 in air. Find the focal length of this lens if it is immersed in a liquid of refractive index μ.
View SolutionFrom the given figure, find :
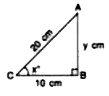
x∘
View SolutionCalcium is tarnished in air , because it is covered with a thin film of
View Solution
