सिद्ध कीजिए की दी हुई तिर्यक ऊंचाई और महत्तम आयतन वाले शंकु का अर्ध शीर्ष कोण tan−1√2 होता है |
2
1
√2
√3
The correct Answer is:C
Volume of cone V=π3r2h
⇒V=π3r2√l2−r2
On differentiating both sides w.r.t r we get
dVdr=π3[2r√l2−r2+r22√l2−r2(−2r)]
Put dVdr=0
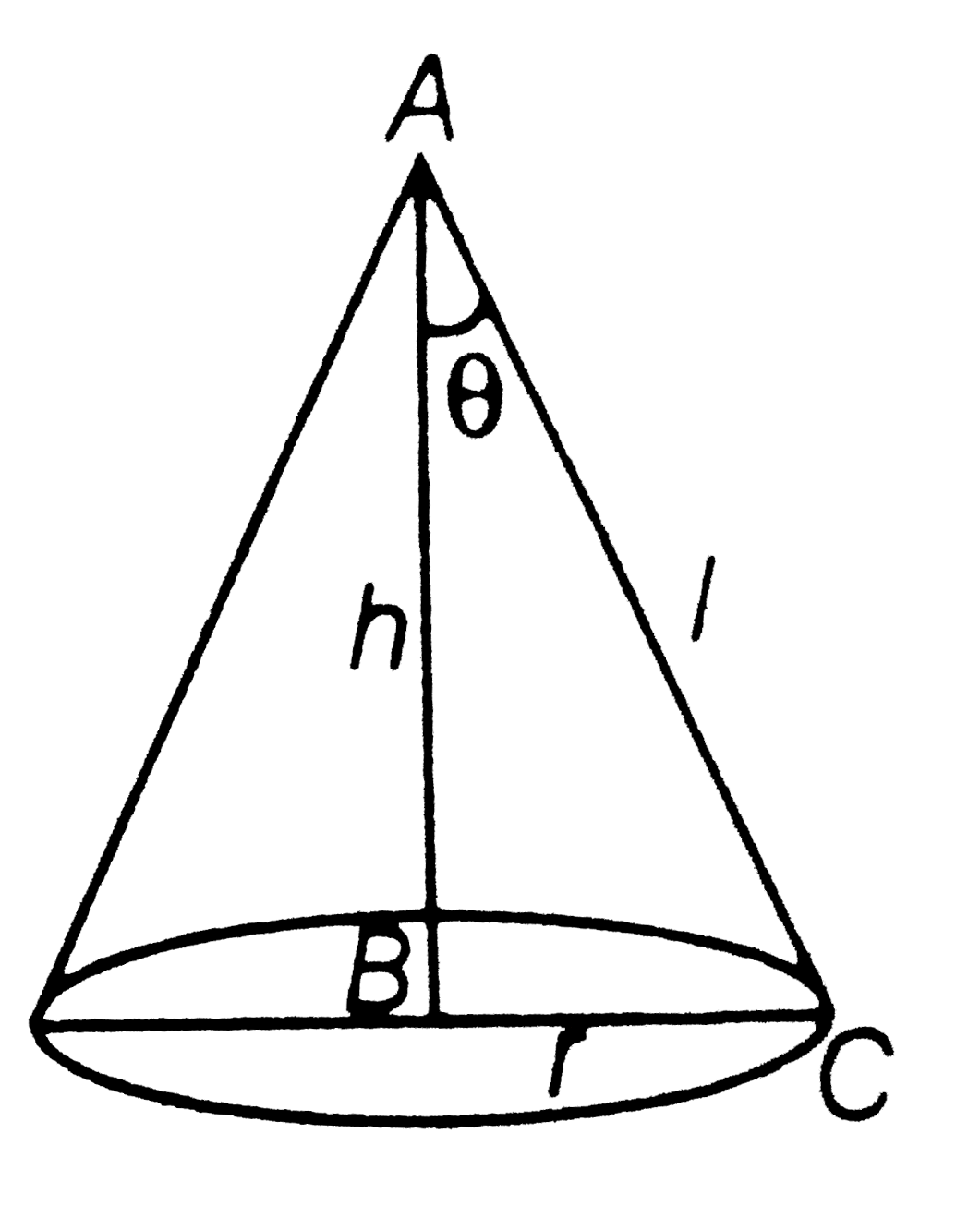
⇒2r(√l2−r2)−r3√l2−r2=0⇒r[2(l2−r2)−r2]=0
⇒2l2−3r2⇒r=±l√23
At r=l√23,d2Vdr2<0, maxima
∴h=√l2−23l2=l√3
In ΔABC,tanθ=rh=l√23l√3=√2
Topper's Solved these Questions
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - MISCELLANEOUS PROBLEMS80videos
APPLICATIONS OF DERIVATIVES
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - MHT CET CORNER21videos
APPLICATIONS OF DERIVATIVES
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - MHT CET Corner6videos
APPLICATIONS OF DEFINITE INTEGRALS
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - BINOMIAL DISTRIBUTIONEXERCISE - MHT CET Corner6videos
BINOMIAL DISTRIBUTION
Similar Questions
The height of a cone is 30cm. A small cone is cut off at the top by a plane parallel to the base. If its volume be 127 th of the volume of the given cone, at what height above the base is the section made?
एक शंकु की ऊँचाई 30 सेमी है । एक छोटा शंकु आधार के समानांतर एक तल द्वारा शीर्ष पर काट दिया जाता है। यदि इसका आयतन दिए गए शंकु के आयतन का 127 हो, तो तल से कितनी ऊँचाई पर खंड किया जाएगा?View SolutionA ladder leaning against a wall makes an angle θ with the horizontal ground such that sinθ=1213. If the foot of the ladder is 7.5 m from a wall, then what is the height of the point from where the top of the ladder touches the wall?
किसी दीवार पर झुकी हुई एक सीढ़ी क्षेतिज भूमि के साथ θ कोण बनाती है जो इस प्रकार है कि sinθ=1213 है | यदि सीढ़ी का तल दीवार से 7.5 मीटर है, तो उस बिंदु की ऊंचाई ज्ञात करें जहाँ से सीढ़ी का शीर्ष भाग दीवार को स्पर्श करता है |View Solution
Knowledge Check
- Question 1 - Select One
गैसीय अभिक्रिया की दर K [A] [B] द्वारा दी जाती है। यदि अभिक्रिया पात्र का आयतन प्रारंभिक आयतन का 14 रह जाता है, तो वास्तविक दर के सापेक्ष अभिक्रिया दर है
A116 गुनाB18 गुनाC8 गुनाD16 गुना - Question 2 - Select One
एक संकुल को CoCl3.xH2O के रूप में प्रदर्शित किया जाता है जलिये माध्यम में इसके ०.1 m विलयन का ΔTf=0.558K होता है जल के लिए Kf=1.86kgmol−1 K है और यह मानते हुए की समन्वय संख्या 6 वाले संकुल का आयतन 100 % होता है तो संकुल का सूत्र है
A[Co(H2O)4Cl]Cl2B[Co(H2O)4Cl]ClC[Co(H2O)6]Cl3D[Co(H2O)5Cl]Cl2
The radius and height of a cone are 20 cm and 21 cm respectively. The total surface area (cm2) of the cone is :
किसी शंकु की त्रिज्या 20 cm तथा ऊंचाई 21 cm है, शंकु का कुल पृष्ठ क्षेत्रफल (cm2 में) है: (π=227)View SolutionThe area of the base of a right circular cone is 400π and its height is 15 cm. The curved surface area of the cone (in cm2) is :
एक लम्ब वृत्तीय शंकु के आधार का क्षेत्रफल 400π है और इसकी ऊंचाई 15 सेमी है | इस शंकु का वक्र पृष्ठ क्षेत्रफल ( वर्ग सेमी में ) है :View SolutionThe given pie chart shows the percentage distribution of 450 employees in an organization. Study the pie chart and answer the questions that follow
दिए गए पाई चार्ट एक संगठन में 450 कर्मचारियों के प्रतिशत को दर्शाता है। पाई चार्ट का अध्ययन करें और आने वाले प्रश्नों का उत्तर दें।
What is the central angle of the sector representing the number of employees in department A?
विभाग A में कर्मचारियों की संख्या का प्रतिनिधित्व करने वाले क्षेत्र का केंद्रीय कोण क्या है?View SolutionThe volume of a right circular cylinder is 3 times the volume of a right circular cone. The radius of the cone and the cylinder are 3cm and 6cm respectively. If the height of the cylinder is 1cm, then what is the slant height of the cone?
किसी लम्ब वृत्तीय बेलन का आयतन एक लम्ब वृत्तीय शंकु के आयतन से तीन गुना है | शंकु तथा बेलन की त्रिज्या क्रमशः 3 सेमी और 6 सेमी है | यदि बेलन की ऊंचाई 1 सेमी है, तो शंकु की तिर्यक ऊंचाई ज्ञात करें |View SolutionThe radii of two circular faces of the frustum of a cone of height 21 cm are 3 cm and 2 cm respectively. What is the volume of the frustum of the cone in cubic cm? (Take π=227)
21 सेमी ऊंचाई वाले शंकु के छिन्नक के दो वृताकार फलकों की त्रिज्या क्रमशः 3 सेमी और 2 सेमी है। घन सेमी में शंकु के छिन्नक का आयतन कितना होगा ?View SolutionA and B standing on the same side of a wall and observe that the angles of elevation to the top of the wall are 45∘ and 60∘ respectively. If the height of the wall is 50 m, the distance between A and B is : (Use √3=1.73 and √2=1.41)
A और B किसी दीवार के एक ही तरफ खड़े हैं तथा देखते हैं कि दीवार के शीर्ष का उन्नयन कोण क्रमशः 45∘ और 60∘ है | यदि इस दीवार की ऊंचाई 50 मीटर है, तो A और B के बीच दूरी ज्ञात करें|View Solution

