A cone of radius 8 cm and height 12 cm is divided into two parts by a plane thrpugh the mid-point of its axis parallel to its base. Find the ratio of the volumes of two parts.
We can solve this using similarity
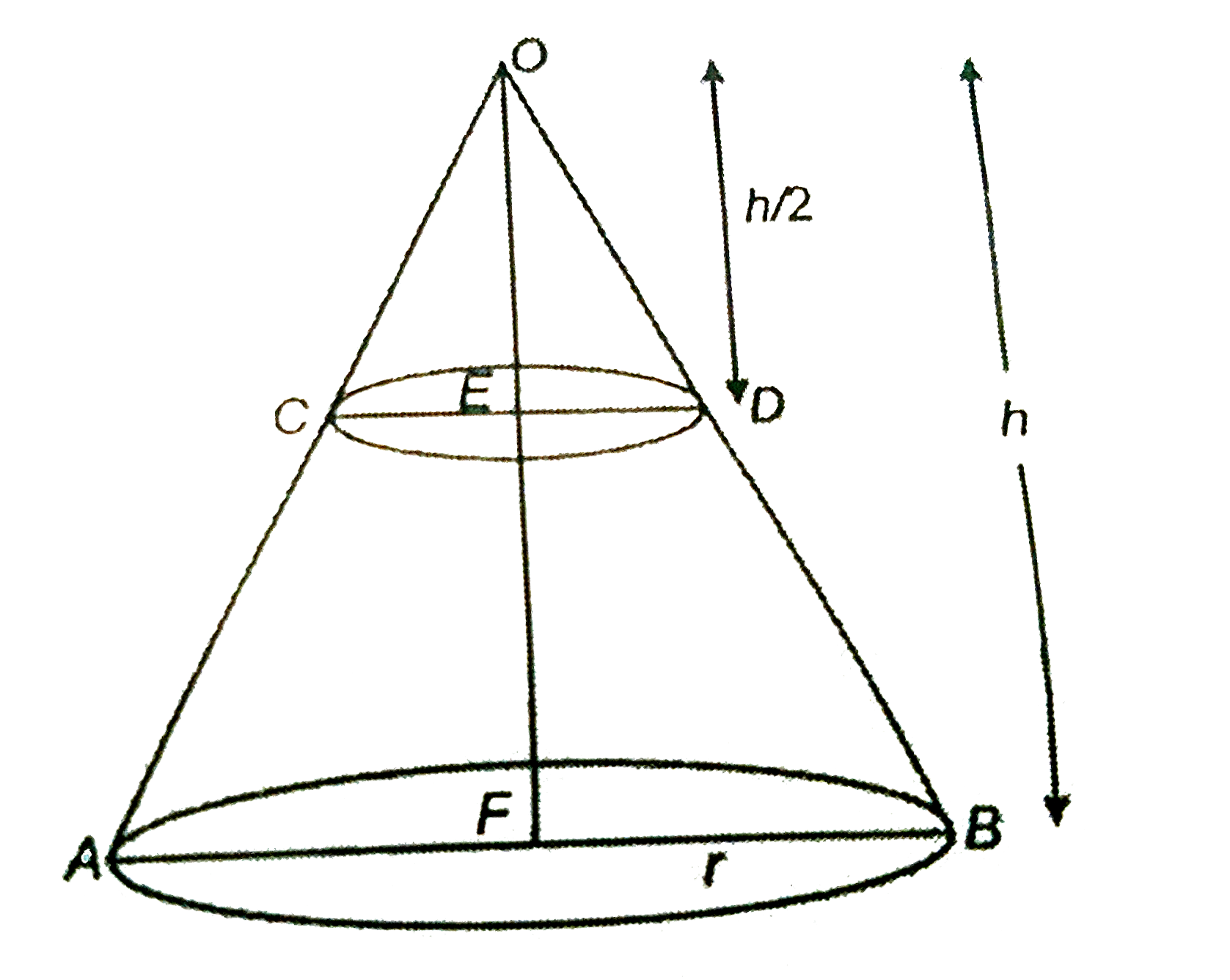
Let r and h be the radius and height of a cone OAB
Let OE=h2
As OED and OFB are similar
there OEOF=EDFBh/2h=EDr
ED=r32
Now volume of cone IOCD =13πr2h=13×π×(r2)2×h2=πr2h24
and volume of cone OAB=13×π×r2×h=πr2h3
∴volume of part OCBvolume of part CDAB=πr2hπr2h3−πr2h24=12413−124=1248−2124=17
Topper's Solved these Questions
- VOLUME AND SURFACE AREA OF SOLIDSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Problems From NCERT/exemplar
10 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Exercise
68 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Revision Exercise Long Questions
1 Videos
Similar Questions
A cone of radius 8 cm and height 12 cm is divided into two parts by a plane through the mid-point of its axis parallel to its base Find the ratio of the volumes of two parts
View SolutionIf a cone of radius 10cm is divided into two parts by drawing a plane through the mid-point of its axis, parallel to its base. Compare the volumes of the two parts.
View SolutionA cone of radius 4 cm is divided into two parts by drawing a plane through the mid-point of its axis and parallel to its base. Compare the volumes of the two parts.
View SolutionA solid cone of base radius 10 cm is cut into two parts through the mid-point of its height, by a plane parallel to its base. Find the ratio in the volumes of two parts of the cone.
View SolutionA solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. The ratio of the volume of the smaller cone to the whole cone is:
View SolutionIf a cone is cut into two parts by a horizontal plane passing through the mid-point of its axis, the ratio of the volumes of upper and lower part is (a) 1:2 (b) 2:1 (c) 1:7 (d) 1:8
View SolutionA solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. The ratio of the volume of the smaller cone to the whole cone is: 1:2 (b) 1:4 (c) 1:6 (d) 1:8
View SolutionA solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. The ratio of the volume of the smaller cone to the whole cone is: 1:2 (b) 1:4 (c) 1:6 (d) 1:8
View SolutionIf a cone is cut into two parts by a horizontal plane passing through the mid-point of its axis, the ratio of the volumes f upper and lower part is (a) 1:2 (b) 2:1 (c) 1:7 (d) 1:8
View SolutionA metallic right circular cone 20 cm high and whose vertical angle is 60∘is cut into two parts at the middle of its height by a plane parallel to its base. If the frustum so obtained be drawn into a wire of diameter 116cm find the length of the wire.
View Solution
