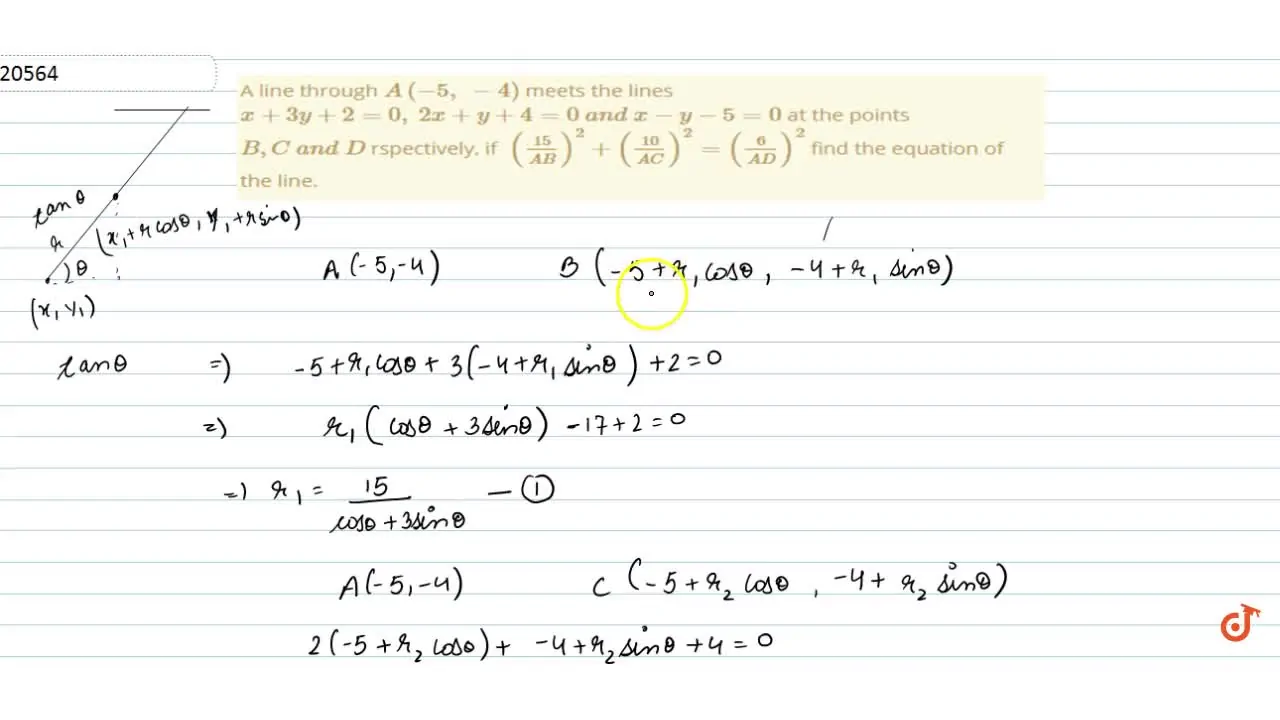
A line through A(−5,−4) meets the lines x+3y+2=0,2x+y+4=0andx−y−5=0 at the points B,CandD rspectively, if (15AB)2+(10AC)2=(6AD)2 find the equation of the line.
Let θ be the inclination of line through A(−5,−4)
Therefore, equation of this line is
x−x1cosθ=y−y1sinθ=r
x+5cosθ=y+4sinθ=r1
So,
x=(rcosθ−5) and y=(rsinθ−4)
So,
Coordinates of A are
(−5+rcosθ,−4+rsinθ)
If AB=r1,AC=r2 and AD=r3,then
B(r1cosθ−5,r1sinθ−4)
C(r2cosθ−5,r2sinθ−4)
D(r3cosθ−5,r3sinθ−4)
given B lies on x+3y+2=0
So,
x+3y−2=0
r1cosθ−5+3r1sinθ−12+2=0
⇒r1cosθ+3r1sinθ−=15
⇒r1(cosθ+3sinθ=15
⇒15cosθ+3sinθ=r1
or 15AB=cosθ+3sinθ−−→(1)
Similarly,
C lies on 2x+y+4=0
we have 2(r2cosθ−5)+(r2sinθ−4)+4=0
⇒2r2cosθ−10+r2sinθ−4+4=0
⇒2r2cosθ+r2sinθ−10=0
⇒r2(2cosθ+sinθ)=10
⇒r2=(2cosθ+sinθ)=10AC→(2)
Similarly, D lies on x−y−5=0
Therefore,
r3cosθ−5−r3sinθ+4−5=0
⇒r3cosθ−r3sinθ−6=0
⇒r3(cosθ−sinθ)=6
⇒(cosθ−sinθ)=6AD→(3)
Now, given that
(15AB)2+(10AC)2=(6AD)2
Putting values from (1),(2) and (3)
(cosθ+3sinθ)2+(2cosθ+sinθ)2
=(cosθ−sinθ)2[Using(1),(2),and(3)]
⇒4cos2θ+9sin2θ+12sinθcosθ=0
or (2cosθ+3sinθ)2=0ortanθ=−23
Hence, the equation of the required line is
y+4=−23(x+5)
=>3y+12=−2x−10 or 2x+3y+22=0
Related Playlists
Similar Questions
A line through A(−5,−4) meets the lines x+3y+2=0,2x+y+4=0andx−y−5=0 at the points B,CandD rspectively, if (15AB)2+(10AC)2=(6AD)2 find the equation of the line.
View SolutionA line through A(-5,-4) meets the lines x+3y+2=0,2x+y+4=0, andx−y−5=0 at B,C and D respectively if
(15AB)2+(10AC)2=(6AD)2 ,then the equation of the lines isView SolutionThe lines x−y−2=0,x+y−4=0,x+3y=6 meet at the point
View SolutionThe lines x+2y−5=0,2x−3y+4=0,6x+4y−13=0
View SolutionA line parallel to the line x−3y=2 touches the circle x2+y2−4x+2y−5=0 at the point
View SolutionFind the acute angle between the lines 2x−y+3=0 and x+y+2=0.
View SolutionGiven the four lines with the equations
x+2y−3=0, 3x+4y−7=0,
2x+3y−4=0, 4x+5y−6=0, thenView SolutionIf the lines 3x+2y−5=0,2x−5y+3=0,5x+by+c=0 are concurrent then b+c=
View SolutionThe equation of the line through the intersection of the lines 2x−3y=0 and 4x−5y=2 and
 View Solution
View SolutionFind the point of intersection of the lines 2x−3y+8=0 and 4x+5y=6
View Solution