A rectangular fish tank fish has a base 2 feet wide and 2 feet long. When the tank is partially filled with water, a solid cube with an edge length of 1 foot is placed in the tank. If no overflow of water from the tank is assumed, by how many inches will be level of the water in the tank rise when the cube becomes completely submerged?
16
12
2
3
The correct Answer is:D
To solve the problem of how much the water level in the tank rises when a solid cube with an edge length of 1 foot is submerged, we can follow these steps:
Step 1: Calculate the Volume of the Cube
The volume V of a cube is given by the formula:
V=side3
Given that the edge length of the cube is 1 foot, we convert this to inches (1 foot = 12 inches):
V=12×12×12=1728 cubic inches
Hint: Remember to convert all measurements to the same unit before performing calculations.
Step 2: Determine the Base Area of the Tank
The base area A of the rectangular tank can be calculated using the formula:
A=width×length
The width and length of the tank are both 2 feet, which is equivalent to 24 inches. Therefore:
A=24×24=576 square inches
Hint: Ensure you square the dimensions when calculating area.
Step 3: Relate the Volume of the Displaced Water to the Rise in Water Level
When the cube is submerged, it displaces an amount of water equal to its volume. The volume of water displaced will cause the water level to rise. The volume of the displaced water can also be expressed in terms of the base area and the height of the rise h:
Volume of displaced water=A×h
Setting the volume of the cube equal to the volume of the displaced water gives:
1728=576×h
Hint: This equation relates the volume of the cube to the rise in water level.
Step 4: Solve for the Height h
To find h, rearrange the equation:
h=1728576
Calculating this gives:
h=3 inches
Hint: When dividing, simplify the numbers if possible to make calculations easier.
Final Answer
The water level in the tank rises by 3 inches when the cube is completely submerged.
The volume V of a cube is given by the formula:
V=side3
Given that the edge length of the cube is 1 foot, we convert this to inches (1 foot = 12 inches):
V=12×12×12=1728 cubic inches
The base area A of the rectangular tank can be calculated using the formula:
A=width×length
The width and length of the tank are both 2 feet, which is equivalent to 24 inches. Therefore:
A=24×24=576 square inches
When the cube is submerged, it displaces an amount of water equal to its volume. The volume of water displaced will cause the water level to rise. The volume of the displaced water can also be expressed in terms of the base area and the height of the rise h:
Volume of displaced water=A×h
Setting the volume of the cube equal to the volume of the displaced water gives:
1728=576×h
To find h, rearrange the equation:
h=1728576
Calculating this gives:
h=3 inches
The water level in the tank rises by 3 inches when the cube is completely submerged.
Topper's Solved these Questions
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - Grib-In
32 Videos
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - EXERCISE
45 Videos
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - EXERCISE
45 Videos
- ADDITIONAL TOPICS IN MATH BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATH EXERCISE - PRACTICE TEST
24 Videos
- COMPLEX NUMBERSBOOK - ENGLISH SATCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE
6 Videos
Similar Questions
A rectangular container has base of length 12 cm and width 9 cm . A cube of edge 6 cm is placed in the container and then sufficient water is filled into it so that the cube is just submerged . Find the fall in level of the water. In the container , when the cube is removed.
View SolutionA rectangular tank is placed on a horizontal ground and is filled with water to a height H above the base. A small hole is made on one vertical side at a depth D below the level of the water in the tank. The distance x from the bottom of the tank at which the water jet from the tank will hit the ground is
View Solution
Knowledge Check
A closed rectangular tank contains a certain amount of water. When the tank is placed on its 3 ft. by 4 ft. side, the height of the water in the tank is 5 ft. when the tank is placed on another side of dimension 4 ft. by 5 ft., what is the height, in feet, of the surface of the water above the ground?
A2B3C4D5An oil tank has two pipes connected to it. If the tank is empty, Pipe A can fill it in 2 hours. If the tank is full, Pipe B can empty it in 3 hours. If both pipes are activated at the same time. When the tank is empty, how many hours will it take for the tank to be filled to 60% of its capacity?
A2.4B3.6C6D60
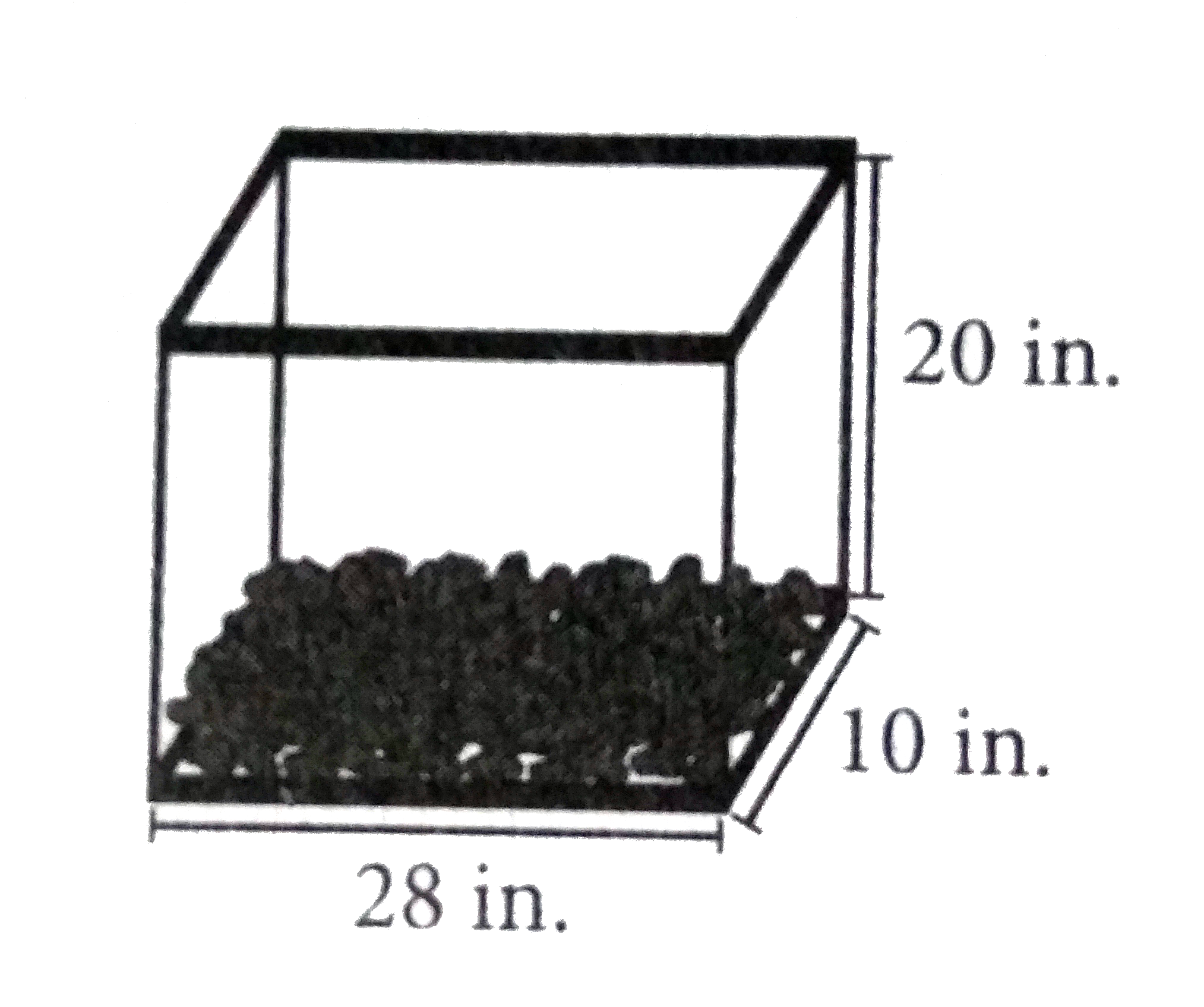
The bottom of the fish tank shown in filled with rocks. The tank is then with water ot a height of 18 inches. When the rocks are removed, the height of the water drops to 16.5 inches. How many cubic inches of water do the rocks displace?
View SolutionT=R2r2√2hg
Suppose an open cylindrical tank has a round drain with radius t in the bottom of the tank. When the tank is filled with water to a depth of h centimeters, the time it takes for all the water to drain from the tank is given by the formula above, where R is the radius of the tank (in centimeters) and g=980cm/s2 is the acceleration due to gravity. Suppose such a tank has a radius of 2 meters and is filled to a depth of 4 meters. About how many minutes does it take to empty the tank if the drain has a radius of 5 centimeters? (1 meter=100 centimeter).
View SolutionThe level of water in a tank is 5 m high . A hole of the area 10cm2 is made in the bottom of the tank . The rate of leakage of water from the hole is
View SolutionA rectangular tank is 25m long and 9.5m deep. If 600 cubic metres of water be drawn off the tank, the level of water in the tank goes down by 1.5m. Calculate :
the width of the tank
View SolutionA storage tank is in the form of a cube . When it is full of water, the volume of water is 15.625 m3. If the present depth of water is 1.3 m, then, find the volume of water already used from the tank.
View SolutionAn open rectangular tank 1.5 m wide 2m deep ad 2 m long is half filled with water. It is accelerated horizontally at 3.27m/s2 in the direction of its length determine the depth of water at each end of tank.
View Solution
