A swimming pool is to be drained by cleaning. If L represents the number of litres of water in the pool seconds after the pool has been plugged off to drain and How fast is the water ruining out at the end of 5 seconds? What is the average rate at which the water flows out during the first 5 seconds?
Let L represents the number of litres of water in the pool t seconds after the pool been plugged off to drain , then
So, Rate at which the water is running out =
=
Rate at which the water is running out at the end of 5s
=
== final rate
Since initial rate =
Therefore, Average rate during 5s =(initialrate+Finalrate)/2
=
=
Topper's Solved these Questions
Similar Questions
A swimming pool is to be drained by cleaning. If L represents the number of litres of water in the pool t seconds after the pool has been plugged off to drain and L=2000(10−t)2. How fast is the water ruining out at the end of 5 seconds? What is the average rate at which the water flows out during the first 5 seconds?
View SolutionA swimming pool is filled by three pipes with uniform flow. The first two pipes operating simultaneously fill the pool in the same time during which the pool is filled by the third pipe alone. The second pipe fills the pool 5 hours faster than the first pipe and 4 hours slower than the third pipe. The time required by the first pipe is
a. 6 hrs
b. 10 hrs
c. 15 hrs
d. 30 hrsView Solution
Knowledge Check
- Question 1 - Select One
Electric current in a wire is time rate of flow of charge. The charge in coulombs that passes through a wire after t seconds is given by the function q(t)=t2−2t2+5t+2. Determine the average current (in coulmbe per second) during the first two seconds.
A4AB5AC6AD12A - Question 2 - Select One
The main water line enters a house on the first floor . The line has a gauge pressure of 1.90×105Pa. A faucet on the second floor , 6.50 m above the first floor , is turned off . What is the gauge pressure at this faucet ?
A1.45×105PaB1.26×105PaC2.29×105PaD2.55×105Pa - Question 3 - Select One
Water drains out of a vessel filled with water upto height h through a hole of area A in t seconds.If the height of the water is 4 h then how much time will be required for the water to drain out ?[Assume A<<A0 (area of tank ) ]
At secondsB4 t secondC2 t secondsDt4 seconds
A vertical water jet flows out of a round hole. One of the horizontal sections of the jet has the diameter d=2.0mm while the other section section located l=20mm lower has the diameter which is n=1.5 times less. Find the volume of the water flowing from the hole each second.
View SolutionNazinia is fly fishing in a stream. The tip of her fishing rod is 1.8 m above the surface of the water and the fly at the end of the string rests on the water 3.6 m away and 2.4 m from a point directly under the tip of the rod. Assuming that her string (from the tip of her rod to the fly) is taut, how much string does she have out? If she pulls in the string at the rate of 5 cm per second, what will be the horizontal distance of the fly from her after 12 seconds?
View SolutionNazima is fly fishing in a stream. The tip of her fishing rod is 1.8 m above the surface of the water and the fly at the end of the string rests on the water 3.6 m away and 2.4 m from a point directly under the tip of the rod. Assuming that her string (from the tip of her rod to the fly) is taut, how much string does she have out? If she pulls in the string at the rate of 5 cm per second, what will be the horizontal distance of the fly from her after 12 seconds?
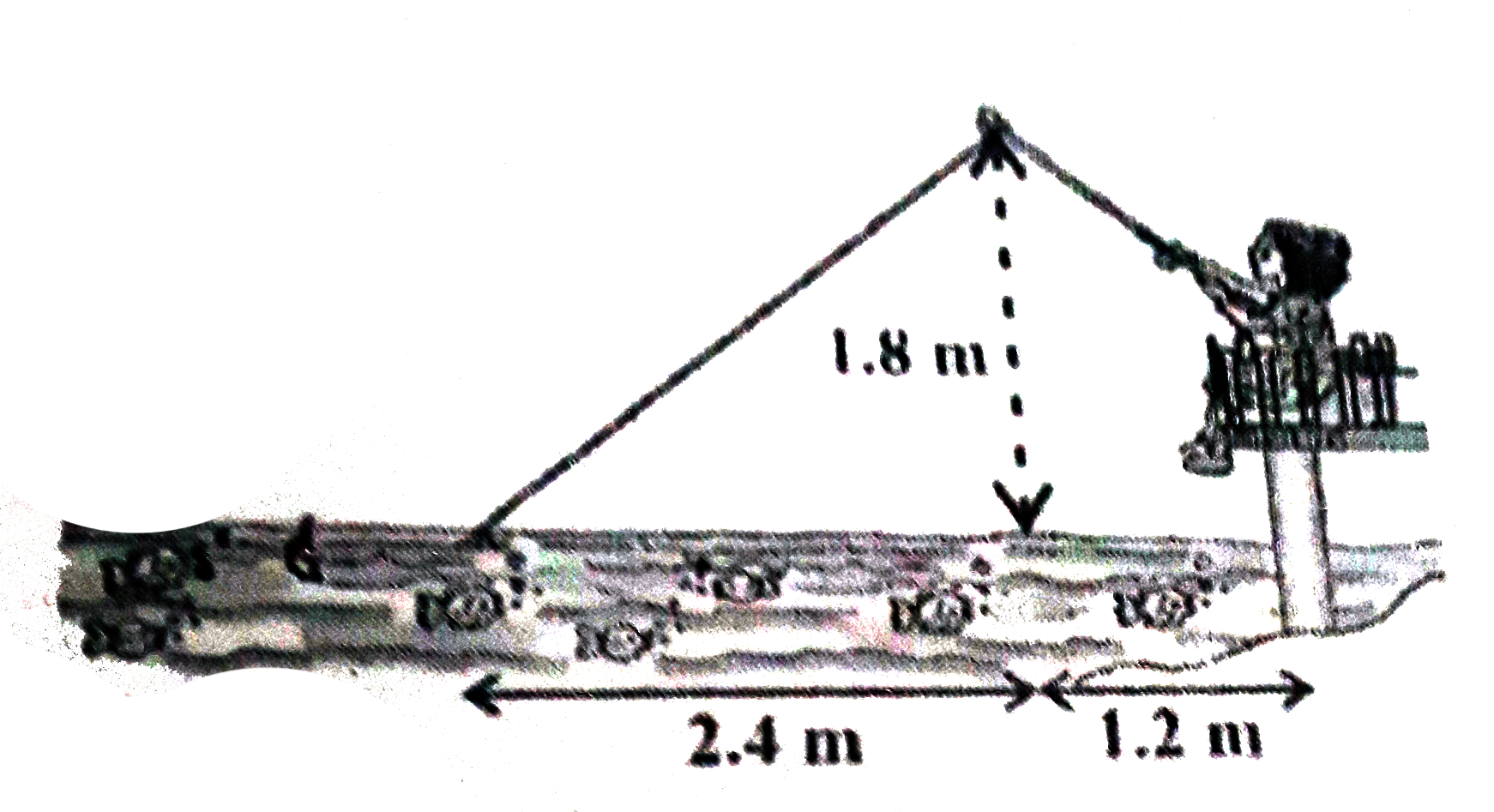 View Solution
View SolutionShailesh lies in a bigh industrial city having a large number of chemical industries onits outskirts. This city has also a very heavy traffic density. There is a big lake in the middle of this city which has much less water left init but the lake has still a large number of fish in it. During the last one and a half months, there ha been to much rain in the city with the pH of rain water being about 5.5. One day people found that many fish in the lake ha died. No one knew the reason for the death of fish in the lake or how the death of remaining fish in the lake could be prevented. Shailesh, who is a student of class X could understand the reason for the death of fish in lake. He also suggested an immediate solutioin for the prevention of death of more fish in the lake water.
(a) What conclusion do you draw the fact that the rain water has a pH of about 5.5? What name is given to such a rain?
(b) What makes the pH of rain water to be about 5.5?
(c) What happens to lake water when too much rain water haivng pH of 5.5 collects in it?
(d) Why have the fish died after the heavy rains?
(e) Why suggestion do you think has been made by Shailesh to prevent the death of more fish in the lake? Why
(f) What values are displayed by Shailesh in this episode?View SolutionSushant has a vessel of the form of an inverted cone, open at the top, of height 11 cm and radius of the top as 2.5 cm and is full of water. Metallic spherical balls each of diameter 0.5 cm are put in the vessel due to which two - fifths of the water in the vessel flows out Find how many balls were put in teh vessel. Sushant made the arrangement so that the water that flows out irrigates the flower beds. What value has been shown by Sushant ?
View Solution

